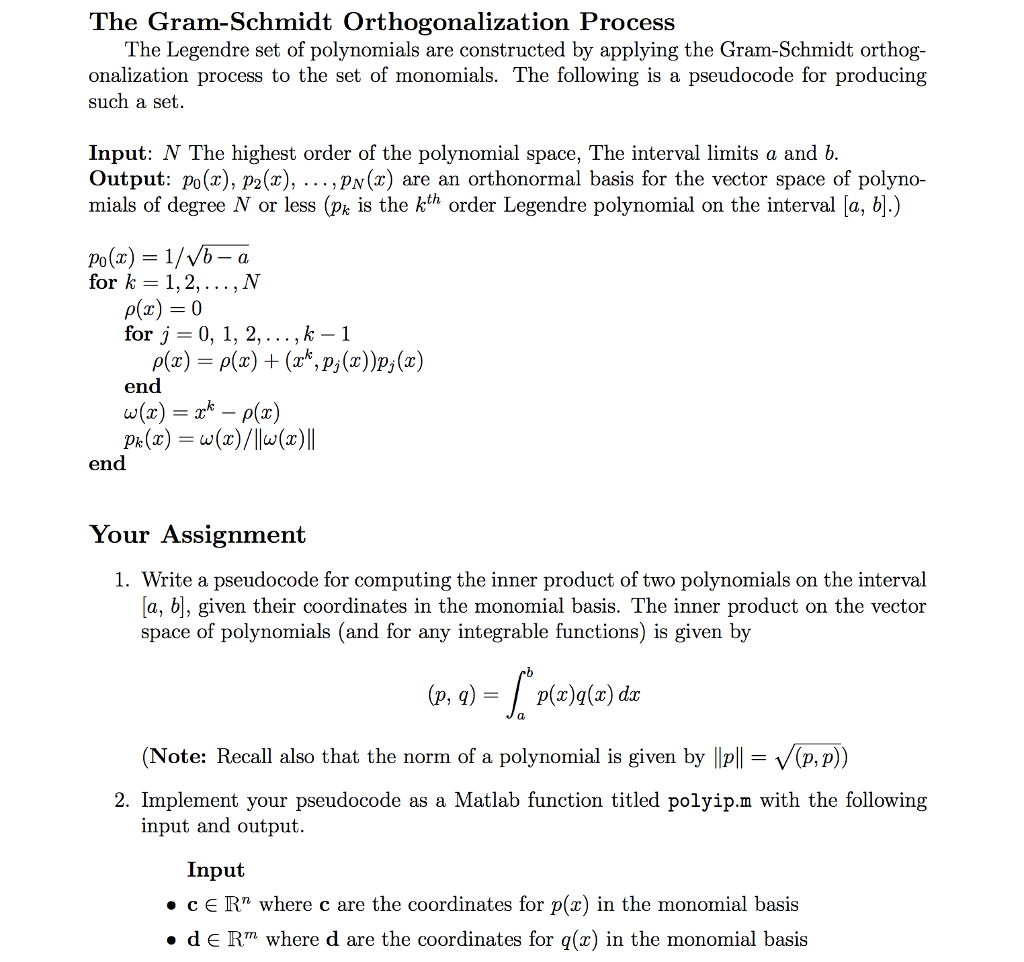
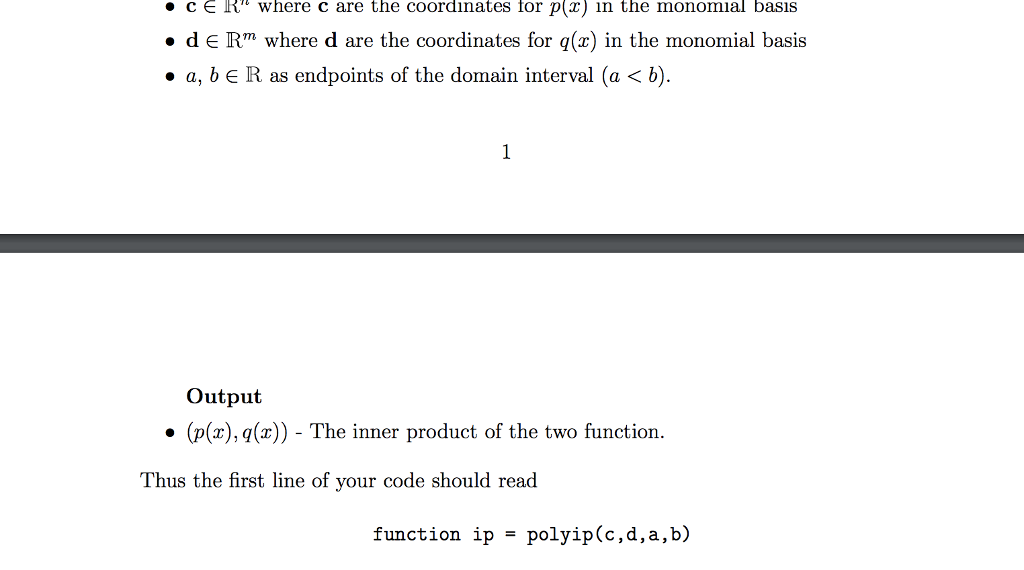
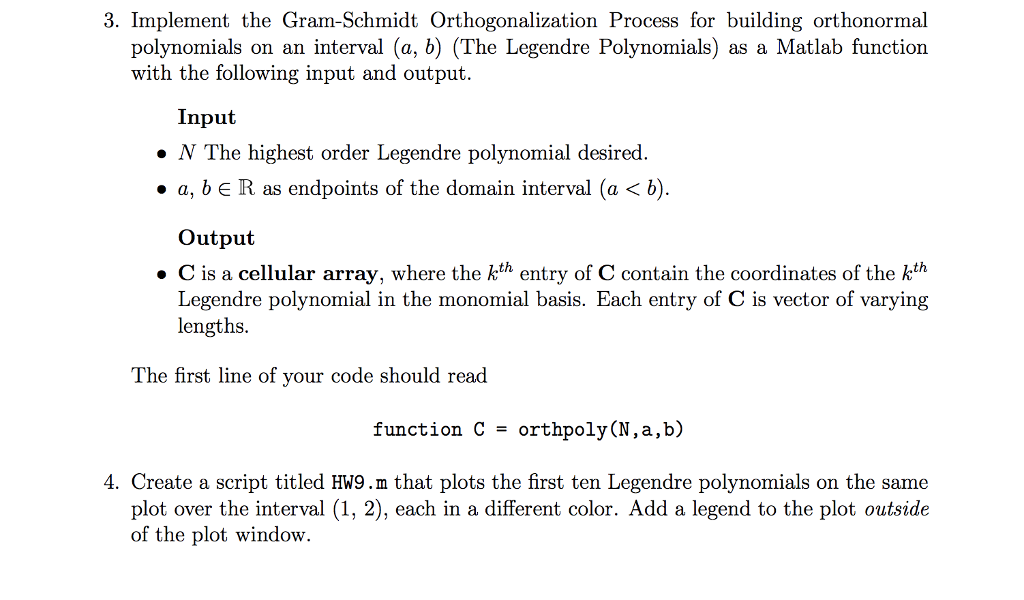
The Gram-Schmidt Orthogonalization Process The Legendre set of polynomials are constructed by applying the Gram-Schmidt orthog- onalization process to the set of monomials. The following is a pseudocode for producing such a set. Input: N The highest order of the polynomial space, The interval limits a and b Output: Po(x), p2(x), ,pN(x) are an orthonormal basis for the vector space of polyno- mials of degree N or less (pk ?s the kth order Legendre polynomial on the interval [a, b].) for k -1,2,... , N for j-0, 1, 2,..., k -1 end end Your Assignment 1. Write a pseudocode for computing the inner product of two polynomials on the interval a, b, given their coordinates in the monomial basis. The inner product on the vector space of polynomials (and for any integrable functions) is given by (p, q)-p(x)q(x) dx Note: Recall also that the norm of a polynomial is given by |Ipl-V(p, p) 2. Implement your pseudocode as a Matlab function titled polyip.m with the following input and output. Input . cER" where c are the coordinates for p(x) in the monomial basis deR" where d are the coordinates for q(x) in the monomial basis The Gram-Schmidt Orthogonalization Process The Legendre set of polynomials are constructed by applying the Gram-Schmidt orthog- onalization process to the set of monomials. The following is a pseudocode for producing such a set. Input: N The highest order of the polynomial space, The interval limits a and b Output: Po(x), p2(x), ,pN(x) are an orthonormal basis for the vector space of polyno- mials of degree N or less (pk ?s the kth order Legendre polynomial on the interval [a, b].) for k -1,2,... , N for j-0, 1, 2,..., k -1 end end Your Assignment 1. Write a pseudocode for computing the inner product of two polynomials on the interval a, b, given their coordinates in the monomial basis. The inner product on the vector space of polynomials (and for any integrable functions) is given by (p, q)-p(x)q(x) dx Note: Recall also that the norm of a polynomial is given by |Ipl-V(p, p) 2. Implement your pseudocode as a Matlab function titled polyip.m with the following input and output. Input . cER" where c are the coordinates for p(x) in the monomial basis deR" where d are the coordinates for q(x) in the monomial basis