The integrated rate law allows chemists to predict the reactant concentration after a certain amount of time, or the time it would take for a certain concentration to be reached. The integrated rate law for a first-order reaction is:

 

Now say we are particularly interested in the time it would take for the concentration to become one-half of its initial value. Then we could substitute   for A and rearrange the equation to:

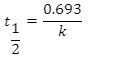
This equation calculates the time required for the reactant concentration to drop to half its initial value. In other words, it calculates the half-life.

Half-life equation for first-order reactions: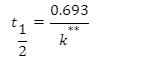

where t_1/2 is the half-life in seconds (s), and k is the rate constant in invers (s^-1).

Part A

To calculate the half-life, plug the value for k into the half-life equation a What is the half-life of a first-order reaction with a rate constant of 8.60 Express answer with the appropriate units.

 

[A] = [A]e -kt