The original question is the classic traveling salesman problem which given a 31*31 distance matrix, use GA and SA algorithms to find the shortest path through all locations. But this extended question asks whether the distance matrix can be reduced first and then the shortest path algorithm can be performed.

Consider methods: SVDPCAICA

I tried PCA, and while the distance relationship can be observed from the pc1/pc2 graph, I don't know how to proceed with the shortest path algorithm(GASA) .

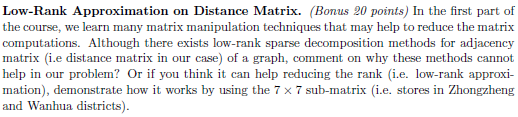
Low-Rank Approximation on Distance Matrix. (Bonus 20 points) In the first part of the course, we learn many matrix manipulation techniques that may help to reduce the matrix computations. Although there exists low-rank sparse decomposition methods for adjacency matrix (i.e distance matrix in our case) of a graph, comment on why these methods cannot help in our problem? Or if you think it can help reducing the rank (i.e. low-rank approximation), demonstrate how it works by using the 77 sub-matrix (i.e. stores in Zhongzheng and Wanhua districts)