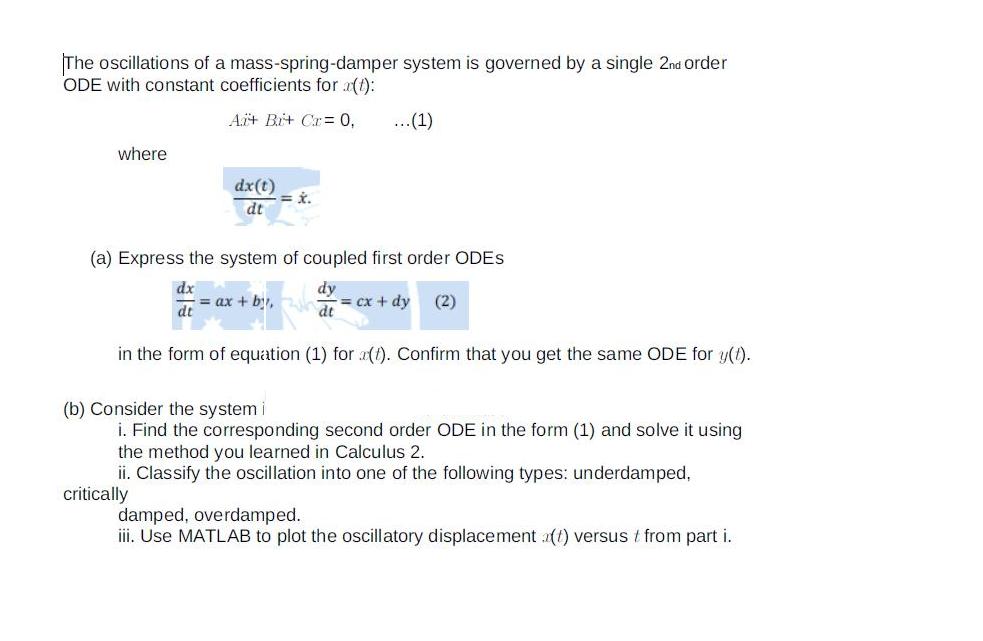
The oscillations of a mass-spring-damper system is governed by a single 2nd order ODE with constant coefficients for a(t): At Bi+ Cr= 0, where dx(t) dt = x. = ax + by, (a) Express the system of coupled first order ODES dx dt ...(1) dt = cx + dy (2) in the form of equation (1) for a(t). Confirm that you get the same ODE for y(t). (b) Consider the system i i. Find the corresponding second order ODE in the form (1) and solve it using the method you learned in Calculus 2. ii. Classify the oscillation into one of the following types: underdamped, critically damped, overdamped. iii. Use MATLAB to plot the oscillatory displacement (t) versus t from part i.