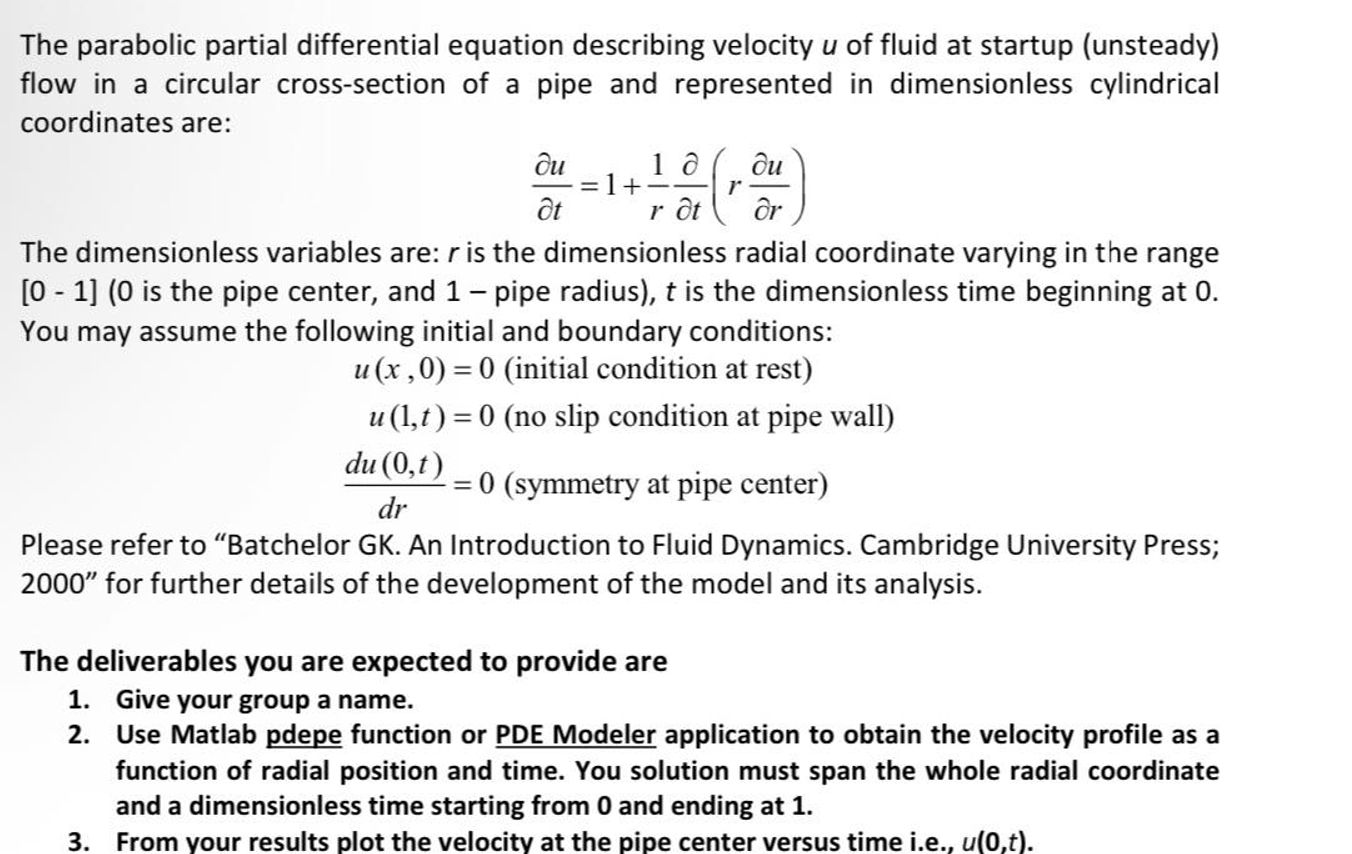
The parabolic partial differential equation describing velocity u of fluid at startup (unsteady) flow in a circular cross-section of a pipe and represented in dimensionless cylindrical coordinates are: 18 du r r t or The dimensionless variables are: r is the dimensionless radial coordinate varying in the range [01] (0 is the pipe center, and 1-pipe radius), t is the dimensionless time beginning at 0. You may assume the following initial and boundary conditions: u (x,0) = 0 (initial condition at rest) u (1,t) = 0 (no slip condition at pipe wall) du (0,t) at =1+ - = 0 (symmetry at pipe center) dr Please refer to "Batchelor GK. An Introduction to Fluid Dynamics. Cambridge University Press; 2000" for further details of the development of the model and its analysis. The deliverables you are expected to provide are 1. Give your group a name. 2. Use Matlab pdepe function or PDE Modeler application to obtain the velocity profile as a function of radial position and time. You solution must span the whole radial coordinate and a dimensionless time starting from 0 and ending at 1. 3. From your results plot the velocity at the pipe center versus time i.e., u(0,t).