Question
The path of a particle is modeled by the function f ( x ) = 1/20 x 2 + 4 x + 7 where f
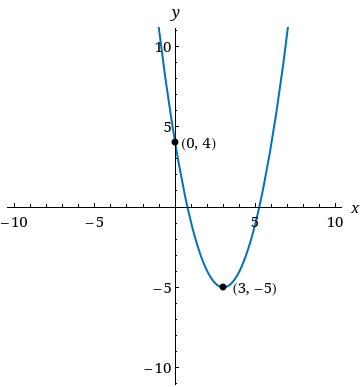
The path of a particle is modeled by the function
- f(x) = 1/20x2 + 4x + 7
where f(x) is the height (in feet) of the particle and x is the horizontal distance (in feet) from where the particle started moving
a. What is the maximum height (in ft) of the particle?
b. Which number determines the height at which the particle started moving?
c. Does changing this value change the coordinates of the maximum height of the particle? select correct answer.
- Yes, changing this value results in a vertical shift of the graph, so the maximum height changes.
- Yes, changing this value results in both a vertical and a horizontal shift of the graph, so the maximum height changes.
- No, changing this value results in a horizontal shift of the graph, so the maximum height does not change
- No, changing this value does not shift the graph, so the maximum height does not change.
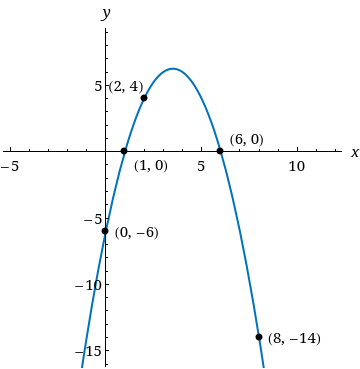
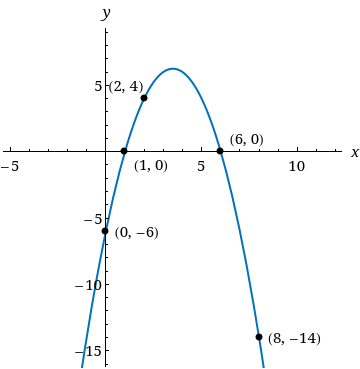
2. For each part, write a cubic function of the form f(x) = (x k)q(x) + r whose graph has the specified characteristics. (There are many correct answers.)
a. passes through the point (5, 8) and rises to the right f(x) =
b. passes through the point (6, 4) and falls to the right
f(x) =
3. Write the standard form of the equation of the parabola shown below. (Let x be the independent variable and y be the dependent variable.)

Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


