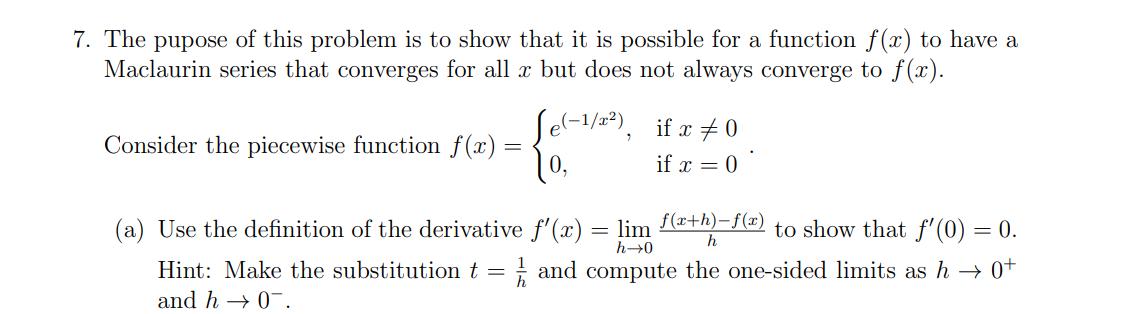
The pupose of this problem is to show that it is possible for a function f(x) to have a Maclaurin series that converges for all x but does not always converge to f(x). Consider the piecewise function f(x) = [e(-1/), if x #0 {of if x = 0 (a) Use the definition of the derivative f'(x) f(x+h)-f(x) lim = to show that f'(0) = 0. h h0 Hint: Make the substitution t = and compute the one-sided limits as h0+ and h0. (b) Assuming that f(n)(0) = 0 for n 2, find the Macluarin series for f(x) and the interval of convergence for the series. (c) Find the values of x for which the Maclaurin series converges to f(x).