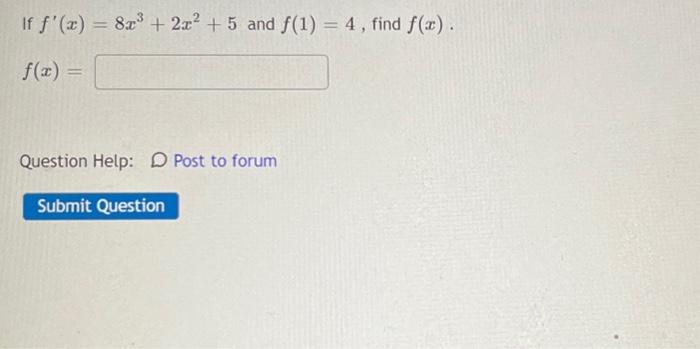
The rate of change of the population of Calcville can be modeled by the function P'(t) 8 1e0 014t where t is the number of years since 1960 In 1980, the population of Calcville was 394096 Find the function P(t) that models the population of Calcville P(t) Estimate the population of Calcville in th...

The Answer is in the image, click to view ...

The rate of change of the population of Calcville can be modeled by the function P'(t)...

Fantastic news! We've Found the answer you've been seeking!

Question:

Related Book For answer-question

Mathematical Applications for the Management Life and Social Sciences

ISBN: 978-1305108042

11th edition

Authors: Ronald J. Harshbarger, James J. Reynolds

See More Books

Posted Date: Mar 05, 2024 09:01 PM

See More Questions

The table gives the number of millions of Social Security beneficiaries (actual and projected) for selected years from 1950 through 2030. With B(t) representing the number of beneficiaries (in...
The economic dependency ratio is defined as the number of persons in the total population who are not in the workforce per 100 in the workforce. Since 1960, Baby Boomers in the workforce coupled with...
Consider the approximation of the welfare loss due to inter-area deviations from the correct rate of care. All else equal, which procedures would yield the largest welfare losses those with low price...
New City College reported deferred revenues of $482,000 as of July 1, 2016, the first day of its fiscal year. Record the following transactions related to student tuition and fees and related...
Harry Smith owns a metal-producing firm that is an unregulated monopoly. After considerable experimentation and research, he finds that the firm's marginal cost curve can be approximated by a...
During your audit of the financial statements of The Gary Manufacturing Company for the year ended December 31, 1999, you find that at January 1, 1999, the company had installed the following...
The bank reconciliation prepared by Gatz Company on May 31, 2014, appeared as follows: The Cash account in the General Ledger appeared as follows on June 30 (Gatz uses only a General Journal to...
What steps are needed to write equations for SOP and POS forms? CD AB 00 01 11 10 AB 00 1 1 1 0 00 00 H 01 0 0 1 1 01 0 CD 00 01 11 10 1 1 1 0 1 1 0 11 1 0 1 1 11 1 0 1 1 10 0 1 1 0 10 0 1 1 0
Manuco company has been offered supplies of special ingredient Z at a transfer price of GHS15 per kg by Helpco company, which is part of the same group of companies. Helpco produces and sells special...
Alatorre Corporation, which manufactures shoes, hired a recent college graduate to work in its accounting department. On the first day of work, the accountant was assigned to total a batch of...
Assume the same information as in E14-4, except that the bonds were issued at 84.95 to yield 12%. Instructions Prepare the journal entries to record the following. (Round to the nearest euro.) (a)...
Allegro Supply Company, a newly formed corporation, incurred the following expenditures related to Land, to Buildings, and to Equipment. Instructions Determine the amounts that should be debited to...
Shlee Corporation issued a 4-year, 60,000, zero-interest-bearing note to Garcia Company on January 1, 2015, and received cash of 60,000. In addition, Shlee agreed to sell merchandise to Garcia at an...
On January 1, 2015, McLean Company makes the two following acquisitions. 1. Purchases land having a fair value of 300,000 by issuing a 5-year, zero-interest-bearing promissory note in the face amount...
Complete the journal entries from 9/30/X2 to 6/30/X3 Fair value Fair value Cash Interest Interest Debt Swap out (in) paid Expense 6.41% 7/1/20X1 1,000,000 0 6.48% 9/30/20X1 998,851 (1,149) 0 16,025...
A liquid flows upward through a valve situated in a vertical pipe. Calculate the differential pressure (kPa) between points A and B. The mean velocity of the flow is 4.1 m/s. The specific gravity of...
Suppose that the total revenue function for a commodity is R = 36x - 0.01x2. (a) Find R(100) and tell what it represents. (b) Find the marginal revenue function. (c) Find the marginal revenue at x =...
The rate of production of a new line of products is given by dx/dt =200 [ 1+400/(t+40)2 where x is the number of items produced and t is the number of weeks the products have been in production. 1....
Use matrix A to encode the message "To be or not to be"?
Following are several tests of controls introduced in the chapter: Examine periodic reports by non-Purchasing Department personnel regarding purchase prices and practices. Observe Receiving...
Harold Brodkey plans to apply sequential sampling to the receiving controls used by the Ozyck Corporation, a December 31, 1999 year end manufacturer and supplier of home lighting fixtures. Based on a...
Few departments within a corporation, not-for-profit institution, or governmental entity can have quite as significant an influence on cost containment as Purchasing, since the purchasing function...

The rate of change of the population of Calcville can be modeled by the function P'(t)...

Question:

Expert Answer:

Mathematical Applications for the Management Life and Social Sciences

Students also viewed these mathematics questions