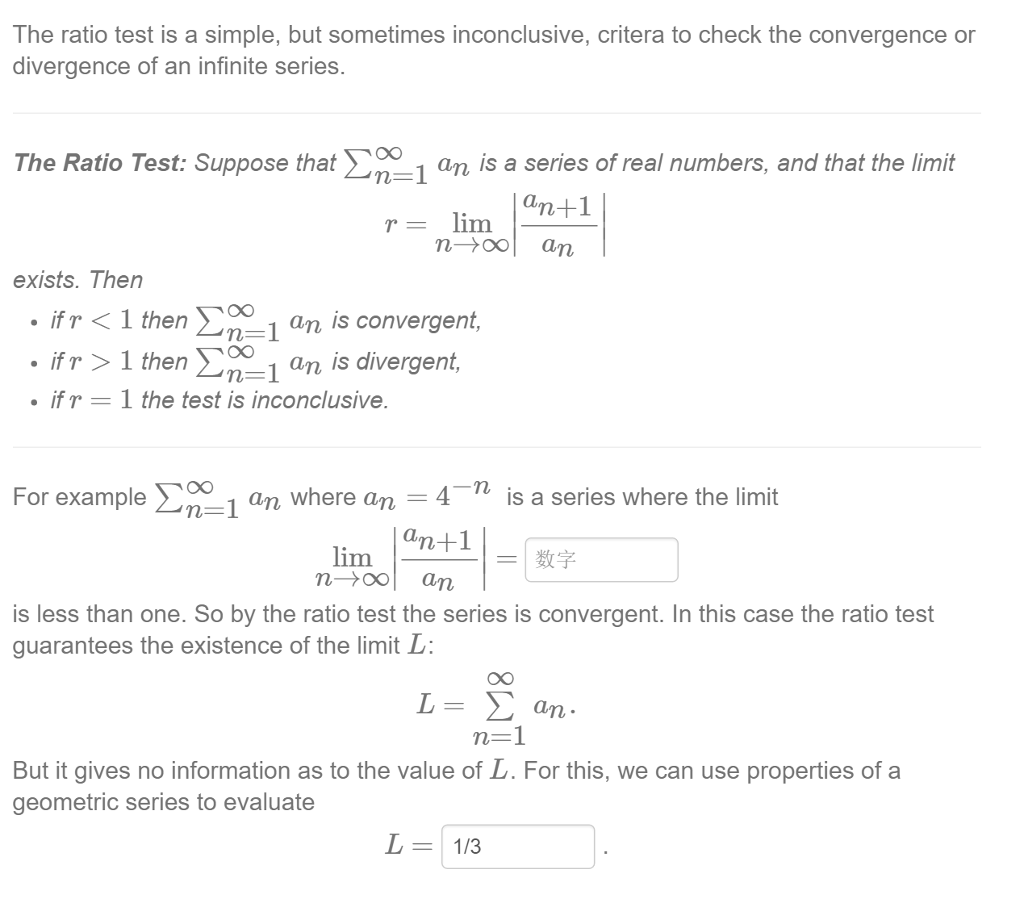
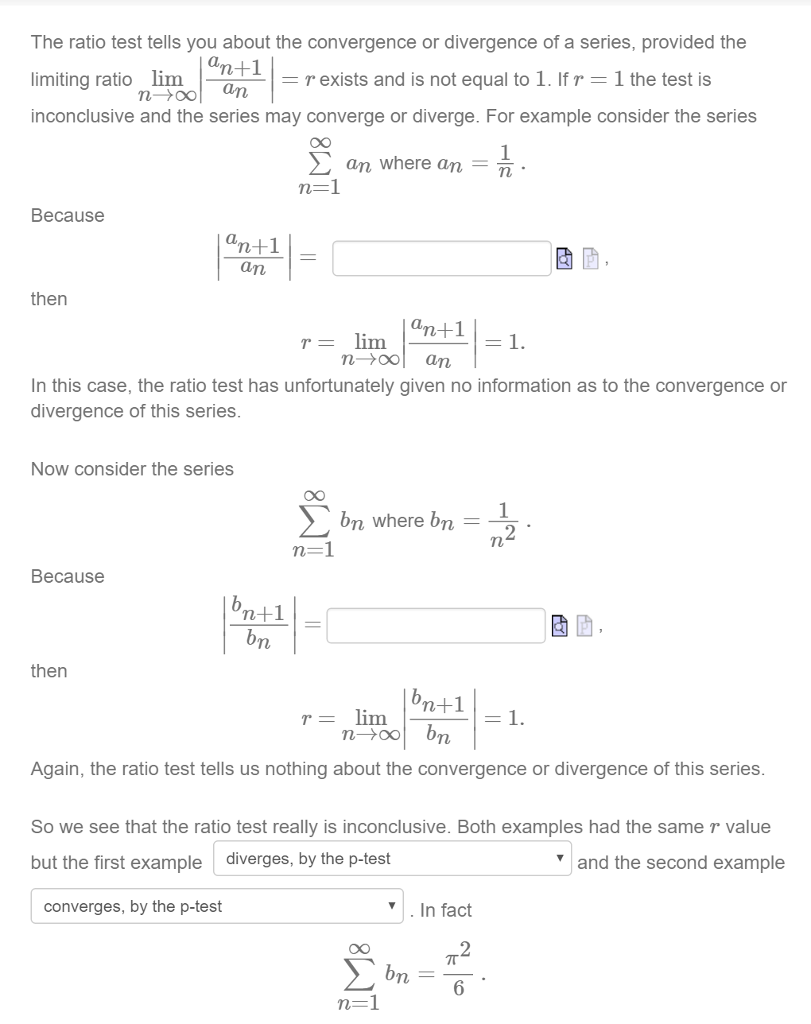
The ratio test is a simple, but sometimes inconclusive, critera to check the convergence or divergence of an infinite series. The Ratio Test: Suppose that n an is a series of real numbers, and that the limit an+1 r = lim n exists. Then if r < 1 then if r >1 then 1 an is convergent, n=1 1 an is divergent, if r 1 the test is inconclusive. n For example 1 an where an = is a series where the limit an+1 lim n an is less than one. So by the ratio test the series is convergent. In this case the ratio test guarantees the existence of the limit L: L = am - n=1 But it gives no information as to the value of L. For this, we can use properties of a geometric series to evaluate L 1/3 The ratio test tells you about the convergence or divergence of a series, provided the limiting ratio lim nx an+1 an r exists and is not equal to 1. If r = 1 the test is inconclusive and the series may converge or diverge. For example consider the series an where an = n n=1 Because an+1 an then an+1 r = lim n-xx an = 1. In this case, the ratio test has unfortunately given no information as to the convergence or divergence of this series. Now consider the series Because n+1 bn then 1 bn where bn 2 n n=1 r = lim | bn+1 bn = 1. nx Again, the ratio test tells us nothing about the convergence or divergence of this series. So we see that the ratio test really is inconclusive. Both examples had the same r value but the first example diverges, by the p-test and the second example converges, by the p-test IM8 bn In fact 6