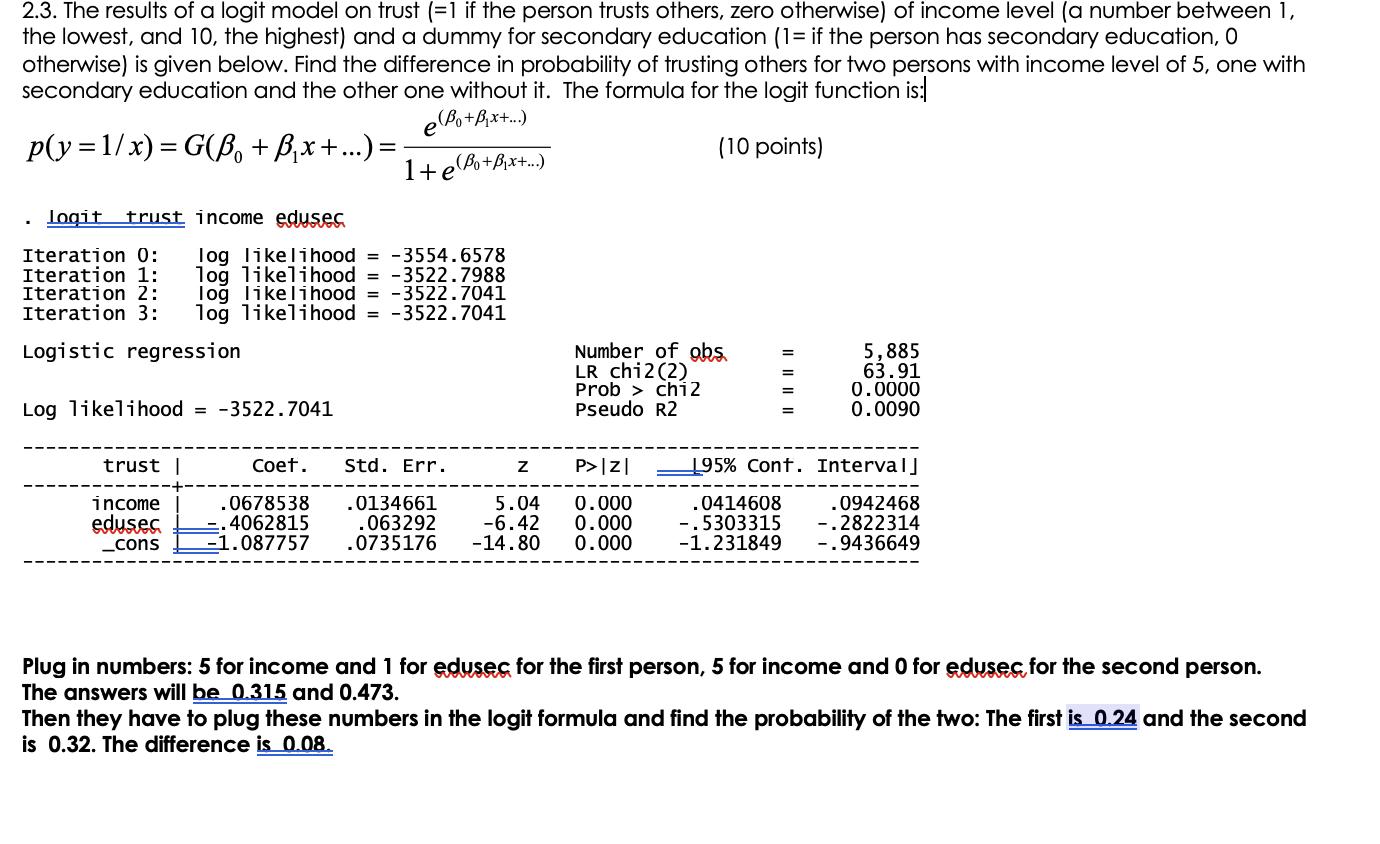
2.3. The results of a logit model on trust (=1 if the person trusts others, zero otherwise) of income level (a number between 1, the lowest, and 10, the highest) and a dummy for secondary education (1= if the person has secondary education, 0 otherwise) is given below. Find the difference in probability of trusting others for two persons with income level of 5, one with secondary education and the other one without it. The formula for the logit function is: e (Bo+Bx+...) 1+e (Bo+Bx+...) (10 points) p(y=1/x)=G(B + Bx + ...) = Logit trust income edusec Iteration 0: log likelihood = -3554.6578 Iteration 1: log likelihood Iteration 2: . -3522.7988 log likelihood = -3522.7041 Iteration 3: log likelihood = -3522.7041 Logistic regression Log likelihood = -3522.7041 trust | income edusec _cons Coef. .0678538 .0134661 4062815 .063292 1.087757 .0735176 Std. Err. Number of obs. LR chi2 (2) Prob chi2 Pseudo R2 Z P> |Z| 5.04 0.000 -6.42 0.000 -14.80 0.000 L95% Cont. .0414608 -.5303315 -1.231849 5,885 63.91 0.0000 0.0090 Interval] .0942468 -.2822314 .9436649 Plug in numbers: 5 for income and 1 for eduses for the first person, 5 for income and 0 for eduses for the second person. The answers will be 0.315 and 0.473. Then they have to plug these numbers in the logit formula and find the probability of the two: The first is 0.24 and the second is 0.32. The difference is 0.08.