The second image is the outline of the class for you to have a more general idea ( should not be beyond chapter 2)

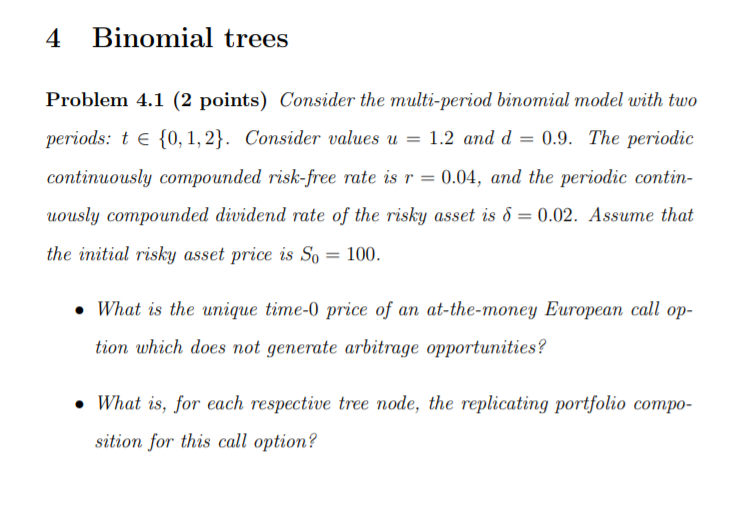
4 Binomial trees Problem 4.1 (2 points) Consider the multi-period binomial model with two periods: t e {0, 1, 2}. Consider values u = 1.2 and d = 0.9. The periodic continuously compounded risk-free rate is r = 0.04, and the periodic contin- uously compounded dividend rate of the risky asset is 8 = 0.02. Assume that the initial risky asset price is So = 100. What is the unique time-0 price of an at-the-money European call tion which does not generate arbitrage opportunities? What is, for each respective tree node, the replicating portfolio compo- sition for this call option? . . Financial derivatives: derivatives specification and cash flows, static no-arbitrage relationships, futures contract. Binomial option pricing models: risk-neutral pricing, replicating portfolio. Black-Scholes option pricing model: lognormal model, Black-Scholes formula, historical volatility. Derivatives risk management: Greeks, hedging. Mean-variance portfolio theory: mean-variance setting, efficient frontier, capital market line. Asset pricing models: CAPM, factor models. Investment Risks: risk measures. Behavioral Finance: efficient market hypothesis (EMH), anomalies, behavioral biases. . . . 4 Binomial trees Problem 4.1 (2 points) Consider the multi-period binomial model with two periods: t e {0, 1, 2}. Consider values u = 1.2 and d = 0.9. The periodic continuously compounded risk-free rate is r = 0.04, and the periodic contin- uously compounded dividend rate of the risky asset is 8 = 0.02. Assume that the initial risky asset price is So = 100. What is the unique time-0 price of an at-the-money European call tion which does not generate arbitrage opportunities? What is, for each respective tree node, the replicating portfolio compo- sition for this call option? . . Financial derivatives: derivatives specification and cash flows, static no-arbitrage relationships, futures contract. Binomial option pricing models: risk-neutral pricing, replicating portfolio. Black-Scholes option pricing model: lognormal model, Black-Scholes formula, historical volatility. Derivatives risk management: Greeks, hedging. Mean-variance portfolio theory: mean-variance setting, efficient frontier, capital market line. Asset pricing models: CAPM, factor models. Investment Risks: risk measures. Behavioral Finance: efficient market hypothesis (EMH), anomalies, behavioral biases