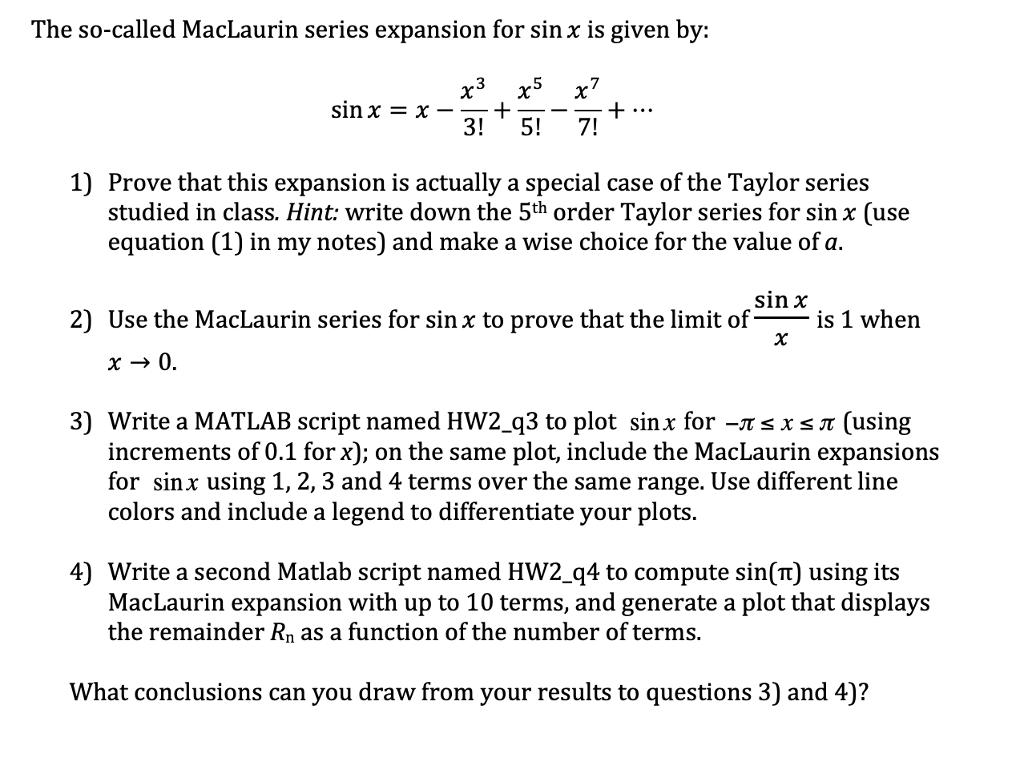
The so-called MacLaurin series expansion for sin x is given by: sin x = x - 3 x5 + - 7 x 3! 5! 7! +... 1) Prove that this expansion is actually a special case of the Taylor series studied in class. Hint: write down the 5th order Taylor series for sin x (use equation (1) in my notes) and make a wise choice for the value of a. 2) Use the MacLaurin series for sin x to prove that the limit of- x 0. sin x x is 1 when 3) Write a MATLAB script named HW2_q3 to plot sinx for - x (using increments of 0.1 for x); on the same plot, include the MacLaurin expansions for sinx using 1, 2, 3 and 4 terms over the same range. Use different line colors and include a legend to differentiate your plots. 4) Write a second Matlab script named HW2_q4 to compute sin(n) using its MacLaurin expansion with up to 10 terms, and generate a plot that displays the remainder Rn as a function of the number of terms. What conclusions can you draw from your results to questions 3) and 4)?