The standard 55 gallon drum is H=88 cm high, has an outer diameter of D =...

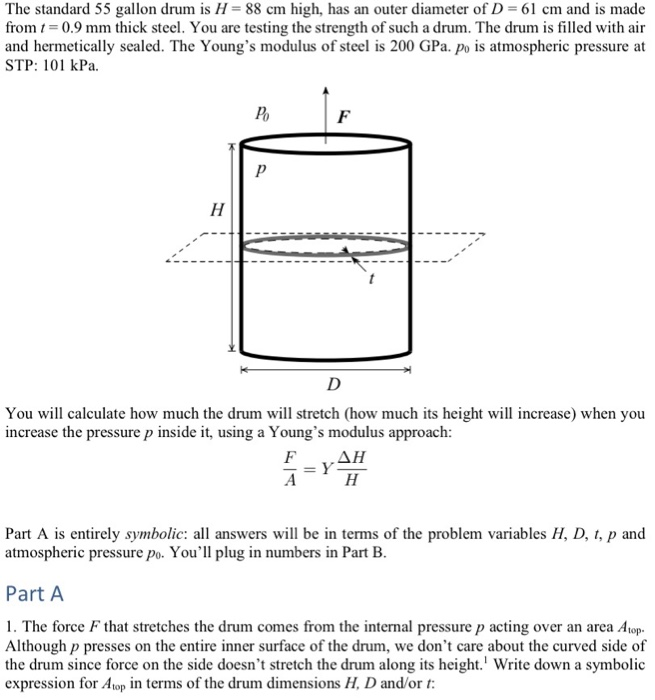
Transcribed Image Text:

The standard 55 gallon drum is H=88 cm high, has an outer diameter of D = 61 cm and is made from 0.9 mm thick steel. You are testing the strength of such a drum. The drum is filled with air and hermetically sealed. The Young's modulus of steel is 200 GPa. po is atmospheric pressure at STP: 101 kPa. Ро F P H D You will calculate how much the drum will stretch (how much its height will increase) when you increase the pressure p inside it, using a Young's modulus approach: F A = Y ΔΗ H Part A is entirely symbolic: all answers will be in terms of the problem variables H, D, t, p and atmospheric pressure po. You'll plug in numbers in Part B. Part A 1. The force F that stretches the drum comes from the internal pressure p acting over an area Atop- Although p presses on the entire inner surface of the drum, we don't care about the curved side of the drum since force on the side doesn't stretch the drum along its height.' Write down a symbolic expression for Atop in terms of the drum dimensions H, D and/or t: 2. Use Asp to write an expression for the stretching force F in terms of p, po and drum dimensions. 3. This stretching force is distributed across the load-bearing cross-sectional area A... Write down a symbolic expression for A,, in terms of the drum dimensions. 4. Use the Young's modulus formula and your answers to (b) and (c) to construct a symbolic expression for the stretch AH in terms of p. po, Y and drum dimensions. Remember that the net force on an area, which is what matters for us, is the pressure difference times the area. To visualize this area, imagine cutting through the drum perpendicular to the stretching force (this is depicted by the dotted plane in the figure): the cross-sectional area that you slice through (the grey line in the figure) is the total area over which your stretching force is distributed. This area is not the same as the one from part (la): A #Ap Hint: the area of an annulus is RZ-R? = (R²-R?)=(R, + R)(R, -R), where R. and R, are the inner and outer radii of the annulus; now relate R. and R. to the drum dimensions D and r. Sincer is so small, it's OK to set D-t D to simplify your expression a little bit. Part B Let's put numbers in your answer from part A. The drum was sealed at room temperature (20 °C) and normal atmospheric pressure. You heat it to boiling (100 °C). 1. What is the pressure in the drum at 100 °C? 2. By how much does the drum's height increase? That is, what is AH? Since your answer will be very small, please give it in mm instead of m. Part C The tensile strength of steel is 250 MPa. The drum will burst when this is reached. 1. At what pressure p will the drum burst? 2. At what temperature T (in °C) will the drum burst? 5 Hint: the tensile strength is the maximum value of F/Axs that the steel sides of the drum can withstand. "This is well above the melting point of steel. Other bad stuff is going to happen to your container well before this temperature: for instance, the steel will soften (meaning the Young's modulus will decrease substantially), and the drum will rapidly deform. The standard 55 gallon drum is H=88 cm high, has an outer diameter of D = 61 cm and is made from 0.9 mm thick steel. You are testing the strength of such a drum. The drum is filled with air and hermetically sealed. The Young's modulus of steel is 200 GPa. po is atmospheric pressure at STP: 101 kPa. Ро F P H D You will calculate how much the drum will stretch (how much its height will increase) when you increase the pressure p inside it, using a Young's modulus approach: F A = Y ΔΗ H Part A is entirely symbolic: all answers will be in terms of the problem variables H, D, t, p and atmospheric pressure po. You'll plug in numbers in Part B. Part A 1. The force F that stretches the drum comes from the internal pressure p acting over an area Atop- Although p presses on the entire inner surface of the drum, we don't care about the curved side of the drum since force on the side doesn't stretch the drum along its height.' Write down a symbolic expression for Atop in terms of the drum dimensions H, D and/or t: 2. Use Asp to write an expression for the stretching force F in terms of p, po and drum dimensions. 3. This stretching force is distributed across the load-bearing cross-sectional area A... Write down a symbolic expression for A,, in terms of the drum dimensions. 4. Use the Young's modulus formula and your answers to (b) and (c) to construct a symbolic expression for the stretch AH in terms of p. po, Y and drum dimensions. Remember that the net force on an area, which is what matters for us, is the pressure difference times the area. To visualize this area, imagine cutting through the drum perpendicular to the stretching force (this is depicted by the dotted plane in the figure): the cross-sectional area that you slice through (the grey line in the figure) is the total area over which your stretching force is distributed. This area is not the same as the one from part (la): A #Ap Hint: the area of an annulus is RZ-R? = (R²-R?)=(R, + R)(R, -R), where R. and R, are the inner and outer radii of the annulus; now relate R. and R. to the drum dimensions D and r. Sincer is so small, it's OK to set D-t D to simplify your expression a little bit. Part B Let's put numbers in your answer from part A. The drum was sealed at room temperature (20 °C) and normal atmospheric pressure. You heat it to boiling (100 °C). 1. What is the pressure in the drum at 100 °C? 2. By how much does the drum's height increase? That is, what is AH? Since your answer will be very small, please give it in mm instead of m. Part C The tensile strength of steel is 250 MPa. The drum will burst when this is reached. 1. At what pressure p will the drum burst? 2. At what temperature T (in °C) will the drum burst? 5 Hint: the tensile strength is the maximum value of F/Axs that the steel sides of the drum can withstand. "This is well above the melting point of steel. Other bad stuff is going to happen to your container well before this temperature: for instance, the steel will soften (meaning the Young's modulus will decrease substantially), and the drum will rapidly deform.

Answer rating: 100% (QA)

Answer 1 Answer 2 The increase in the drums height denoted as H can be calculated using the formula H V r12 r1 r2 r22 Where H increase in height V vol... View the full answer