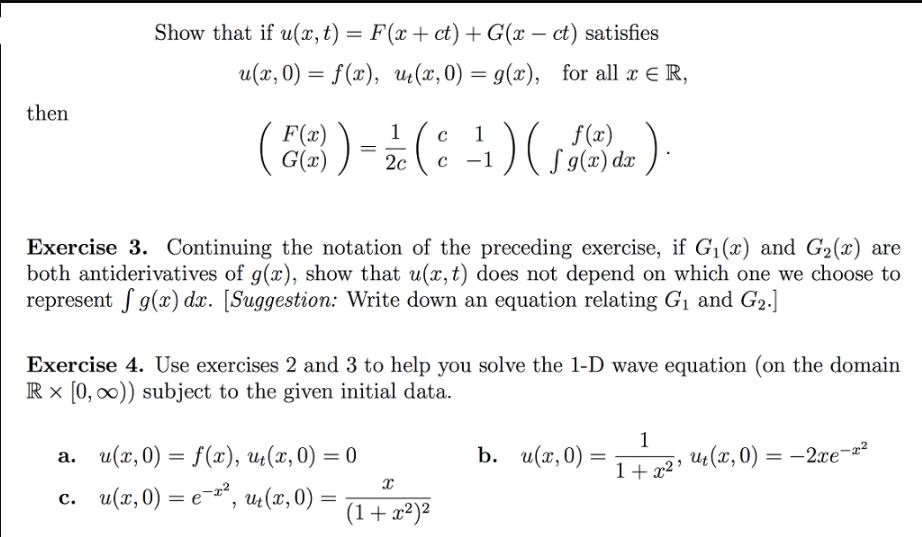
then Show that if u(x,t) = F(x + ct) + G(x - ct) satisfies u(x, 0) = f(x), u(x, 0) = g(x), for all x = R, 1 C 2c f(x) 9(x)dx ((x)) = (-1) (())- Exercise 3. Continuing the notation of the preceding exercise, if G(x) and G2(x) are both antiderivatives of g(x), show that u(x, t) does not depend on which one we choose to represent g(x) dx. [Suggestion: Write down an equation relating G and G2.] Exercise 4. Use exercises 2 and 3 to help you solve the 1-D wave equation (on the domain Rx [0, )) subject to the given initial data. a. u(x, 0) = f(x), u(x, 0) = 0 1 b. u(x, 0) = 1+x' ut(x, 0) = -2xe-x == c. u(x, 0) = ex, ut(x, 0) = = (1 + x2)2 X