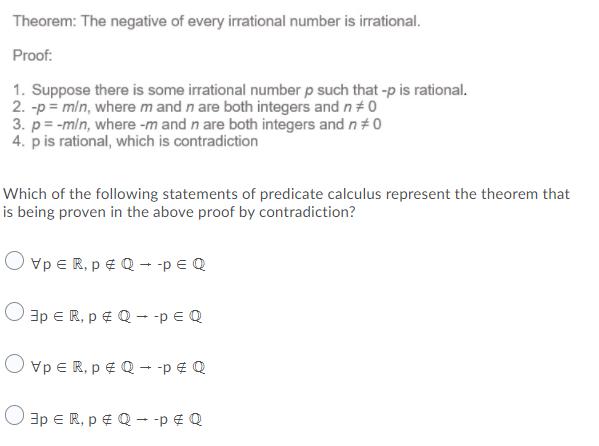
Theorem: The negative of every irrational number is irrational. Proof: 1. Suppose there is some irrational number p such that -p is rational. 2. -p = m/n, where m and n are both integers and n # 0 3. p = -min, where -m and n are both integers and n #0 4. p is rational, which is contradiction Which of the following statements of predicate calculus represent the theorem that is being proven in the above proof by contradiction? OVER,p #Q--P E Q O 3p R, p #Q- -PEQ OVER.p#Q- -p #Q 3p ER, P Q - -p #Q