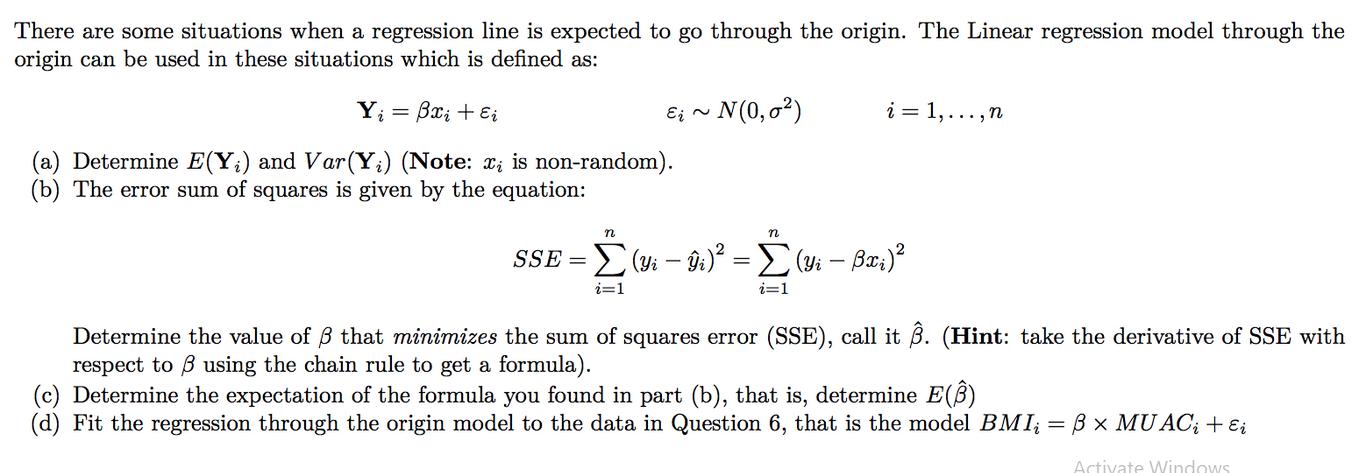
There are some situations when a regression line is expected to go through the origin. The Linear regression model through the origin can be used in these situations which is defined as: Y = xi + i E ~ N(0,0) i = 1,...,n (a) Determine E(Y) and Var(Y) (Note: x is non-random). (b) The error sum of squares is given by the equation: n n SSE = - - - i=1 - = i=1 Determine the value of that minimizes the sum of squares error (SSE), call it B. (Hint: take the derivative of SSE with respect to using the chain rule to get a formula). (c) Determine the expectation of the formula you found in part (b), that is, determine E(B) (d) Fit the regression through the origin model to the data in Question 6, that is the model BMI, Bx MUAC + Ei Activate Windows