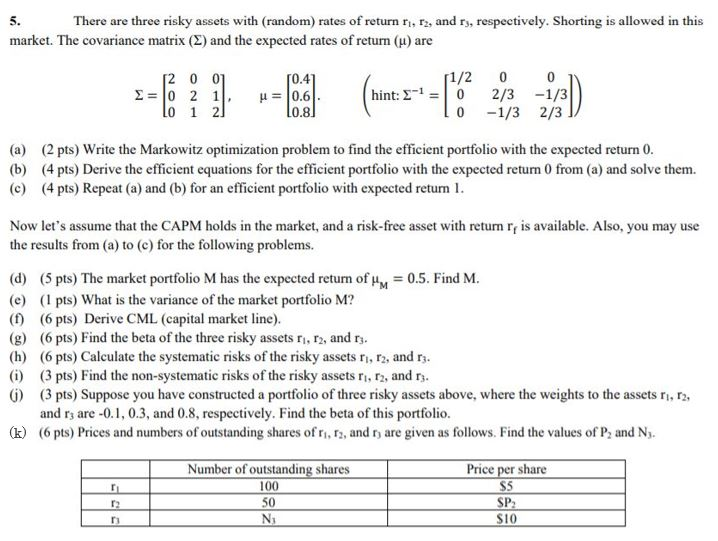
There are three risky assets with (random) rates of return r, 12, and rs, respectively. Shorting is allowed in this market. The covariance matrix (E) and the expected rates of return (s) are 12 001 = 10 2 1, lo 1 2 u = 11/ 2001 hint: -1 = 0 2/3 -1/3 10 -1/3 2/3 ] (a) (b) (c) (2 pts) Write the Markowitz optimization problem to find the efficient portfolio with the expected return 0. (4 pts) Derive the efficient equations for the efficient portfolio with the expected return 0 from (a) and solve them. (4 pts) Repeat (a) and (b) for an efficient portfolio with expected return 1. Now let's assume that the CAPM holds in the market, and a risk-free asset with retum ris available. Also, you may use the results from (a) to (c) for the following problems. (d) (5 pts) The market portfolio M has the expected return of f = 0.5. Find M. (e) (1 pts) What is the variance of the market portfolio M? (1) (6 pts) Derive CML (capital market line). (g) (6 pts) Find the beta of the three risky assets ri, 12, and ry. (h) (6 pts) Calculate the systematic risks of the risky assets 11, 12, and ry. (i) (3 pts) Find the non-systematic risks of the risky assets ri, 12, and rz. 0) (3 pts) Suppose you have constructed a portfolio of three risky assets above, where the weights to the assets 11, 12, and r3 are -0.1, 0.3, and 0.8, respectively. Find the beta of this portfolio. (k) (6 pts) Prices and numbers of outstanding shares of ri, , and r) are given as follows. Find the values of P, and Ny. Number of outstanding shares Price per share TL 100 SS 12 5 0 SP2 DNS10 There are three risky assets with (random) rates of return r, 12, and rs, respectively. Shorting is allowed in this market. The covariance matrix (E) and the expected rates of return (s) are 12 001 = 10 2 1, lo 1 2 u = 11/ 2001 hint: -1 = 0 2/3 -1/3 10 -1/3 2/3 ] (a) (b) (c) (2 pts) Write the Markowitz optimization problem to find the efficient portfolio with the expected return 0. (4 pts) Derive the efficient equations for the efficient portfolio with the expected return 0 from (a) and solve them. (4 pts) Repeat (a) and (b) for an efficient portfolio with expected return 1. Now let's assume that the CAPM holds in the market, and a risk-free asset with retum ris available. Also, you may use the results from (a) to (c) for the following problems. (d) (5 pts) The market portfolio M has the expected return of f = 0.5. Find M. (e) (1 pts) What is the variance of the market portfolio M? (1) (6 pts) Derive CML (capital market line). (g) (6 pts) Find the beta of the three risky assets ri, 12, and ry. (h) (6 pts) Calculate the systematic risks of the risky assets 11, 12, and ry. (i) (3 pts) Find the non-systematic risks of the risky assets ri, 12, and rz. 0) (3 pts) Suppose you have constructed a portfolio of three risky assets above, where the weights to the assets 11, 12, and r3 are -0.1, 0.3, and 0.8, respectively. Find the beta of this portfolio. (k) (6 pts) Prices and numbers of outstanding shares of ri, , and r) are given as follows. Find the values of P, and Ny. Number of outstanding shares Price per share TL 100 SS 12 5 0 SP2 DNS10







