These are 10 questions. Please answer accordingly.

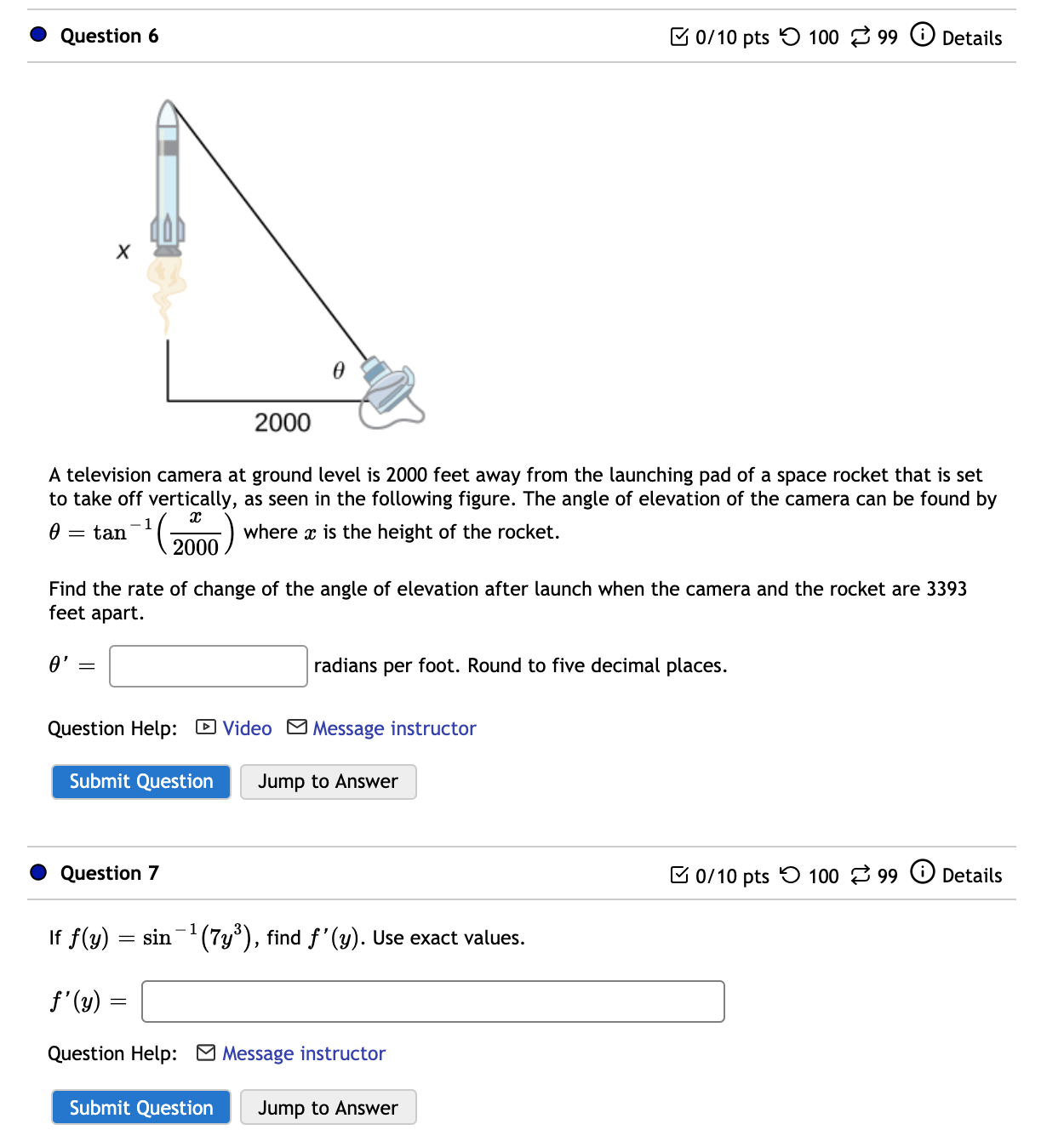
. Question 1 0/10 pts ) 100 99 0 Details For the linear function y = f(x) = - 3x - 2: a. Find dx at x = 6. f' (6) = b. Find a formula for x = f (y). f -1 (y) = df -1 c. Find - - at y = f(6). dy ( f - 1 ) ' ( f (6 ) ) = Question Help: Message instructor Submit Question Jump to Answer Question 2 0/10 pts ' 100 99 0 Details For the function y = f(x) = - 5x + 5: a. Find at x = - 9. dx f' ( - 9) = b. Find a formula for x = f-(y). f-1(y ) = df -1 c. Find at y = f( - 9). dy ( f - 1) ' (f ( - 9) ) = Question Help: Message instructor Submit Question Jump to Answer0 Question 3 E4 0/10 pts '0100 2:5 99 (D Details Let m) = (43mm + 20050:)i:.':1.11'1 a: f'lw)= :] Question Help: 8 Message instructor Submit Question Jump to Answer 0 Question 4 B 0/10 pts I0100 2:3 99 (D Details Differentiate f(a:) = sin' 1(23). Use exact values. we = Question Help: 8 Message instructor Submit Question Jump to Answer 0 Question 5 I11 0/10 pts '0100 2:\" 99 (D Details If f(t) = tan1(4t), find f'(t). Use exact values. \"9 = Question Help: 8 Message instructor Submit Question Jump to Answer 0 Question 6 I21 0/10 pts '0 100 3 99 (9 Details A television camera at ground level is 2000 feet away from the launching pad of a space rocket that is set to take off vertically, as seen in the following figure. The angle of elevation of the camera can be found by a: 0 = tan_1( 2000 Find the rate of change of the angle of elevation after launch when the camera and the rocket are 3393 feet apart. 6 ' = :] radians per foot. Round to five decimal places. Question Help: El Video 8 Message instructor Submit Question Jump to Answer ) where a: is the height of the rocket. 0 Question 7 B 0/10 pts '0 100 8 99 (9 Details If y) = 8111 1(7y3), find f'(y). Use exact values. W) = Question Help: 8 Message instructor Submit Question Jump to Answer Question 8 0/10 pts 100 99 0 Details If f(x) = 4tan -1(3 sin(2x)), f' (x) = Question Help: Message instructor Submit Question Jump to Answer Question 9 0/10 pts ' 100 99 0 Details Let f(x) = tan -(cos(7a)) f' (2) = Question Help: Video Message instructor Submit Question Jump to Answer Question 10 0/10 pts 100 99 0 Details If f(ac) = 2 sin -1 (a4), then f' (2) = Question Help: Video Message instructor Submit Question Jump to