

These questions seems to be difficult to me because I cannot get the correct answers
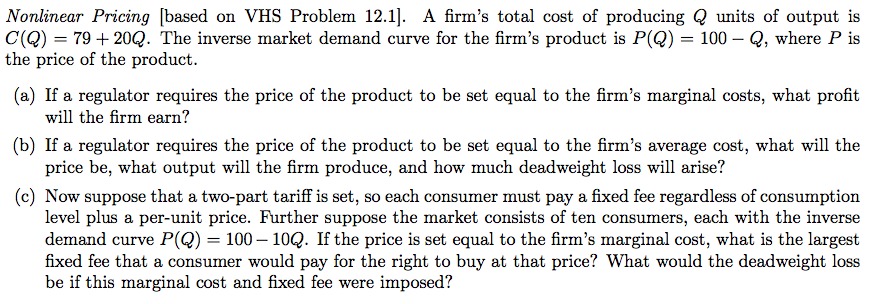
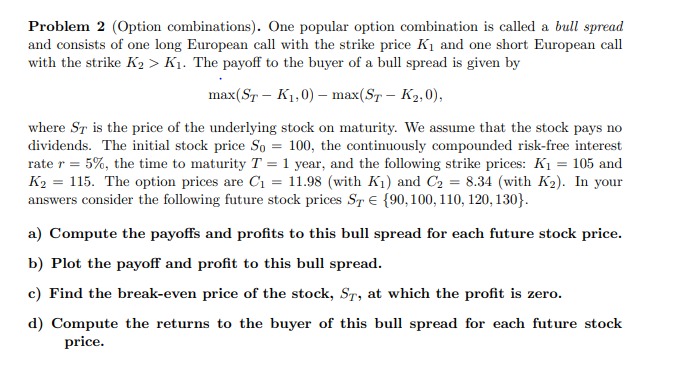
Nonlinear Pricing [based on 1VHS Problem 12.1]. A rm's total cost of producing Q units of output is C(Q) = 7'9 + ZUQ. The inverse market demand curve for the rm's product is P[Q) = 100 _. Q, where P is the price of the product. (a) If a regulator requires the price of the product to be set equal to the rm's marginal costs, what prot will the rm earn? (b) If a regulator requires the price of the product to be set equal to the rm's average cost, what will the price be, what output will the rm produce, and how much deadweight loss will arise? (c) Now suppose that a two-part tari\" is not, In each consumer must pay a xed fee regardless of consumption level plus a per-unit price. Further suppose the market consists of ten consumers, each with the inverse demand curve P(Q) = 100 -- lQ. If the price is set equal to the rm's marginal cost, what is the largest xed fee that a consumer would pay for the right to buy at that price? What would the deadweight loss be if this marginal cost and xed fee were imposed? Problem 2 [Option combinations]. Clue popular option combination is called a but! spread and consists of one long European call with the strike price K1 and one short European call with the strike K2 3&- H1. The payoff to the buyer of a hull spread is given by mafoT K111\" mExLST _ K2: I\": where ST is the price of the underlying stock on maturity. We assume that the stock pays no dividends. The initial stock price 5;; = ll], the continuously compounded risk-free interest rate r = 5%, the time to maturity T = 1 year, and the following strike prism: K1 = 105 and K3 = 115. The option prices are Cl = 11.98 {with K1} and Cg = 3.34 {with Kg}. In your answers consider the following future stock prices ST E {QlL 11B, 120, 131]}. a] Compute the payo's and prots to this hull spread for each future stock price. 1:} Plot the payoff and prot to this hull spread. c} Find the break-even price of the stock, 57, at which the prot is zero. d} Compute the returns to the buyer of this hull spread for each future stock price. Question 3 [subsidy + curve addition (total fuel supply)]: Suppose the demand for (blended) fuel F, can be given by the following demand function: PF = 200 -4F. Fuel retailers are indifferent between using corn derived ethanol E, and crude oil derived gasoline G, in the fuel they sell to consumers. To produce blended fuel, assume ethanol is splash blended in tanker trucks at no additional cost and there are no limits to how much ethanol can be blended into fuel. Thus fuel retailers are willing to supply fuel according to: F = [ + G. Assume that fuel retailers are the only demanders for ethanol and gasoline. Ethanol supply is given by: PF = 1.45 + 6E. Gasoline supply is given by: Pc = 1.25 + 2G. For simplicity suppose fuel, ethanol, and gasoline are all denominated in the above in energy equivalent gallons of gasoline (hereafter, simply "gallons"). Suppose all of the gasoline that is supplied is from crude oil imported from abroad. Suppose corn growers in Iowa convince the government to subsidize ethanol by $4.50/gallon. Relative to the no subsidy, competitive market equilibrium: a. What is the change in producer surplus to ethanol producers due to the subsidy? b. What is the change in producer surplus to gasoline producers due to the subsidy? c. What is the change in fuel demander's consumer surplus due to the subsidy? d. What is the final bill to taxpayers for the subsidy? e. What is the change in total welfare due to the subsidy? f. Iowa corn growers argued that the subsidy would help "reduce our dependence on foreign oil." This is the same thing as saying that they believe there is an additional societal benefit for each gallon of gasoline displaced by the subsidy relative to the competitive market equilibrium, given our prior assumptions. Suppose this is the case. Is the ethanol subsidy a good way to achieve this goal? Why or why not? Explain your reasoning. g. How large would the benefits from reducing oil dependency (per gallon of gasoline displaced) need to be to just justify the welfare loss due to the subsidy?(1) Suppose Home can produce two goods, clothing (C) and wheat (W). It has a total labor supply of 6,000 units. The unit labor requirements for clothing and wheat are as follows: Unit labor requirements Clothing Wheat Home 30 40 (1 point) a. Let Qc and Qw be the quantities of clothing and wheat produced, derive Home's production possibility frontier (PPF) and graph it (You can place Qc on the horizontal axis, Qw on the vertical axis). (1 point) b. What is the opportunity cost of clothing in terms of wheat? (1 point) c. What and how much will Home produce when the relative price of clothing is 1, i.e., Pc/ Pw = 1? (1 point) d. Do clothing workers or wheat workers earn higher wages when the relative price of clothing is 0.75, i.e., Pc/Pw = 0.757 (2) Home is as described above. Now suppose there is another country, Foreign, which also has a total labor supply of 6,000 units. The unit labor requirements for two goods in Foreign are as follows: Unit labor requirements Clothing Wheat Foreign 20 60 (1 point) a. Which country has an absolute advantage over the other in wheat production? Which has a comparative advantage over the other in wheat production? (2 points) b. What and how much will Home and Foreign produce, respectively, when the world relative price of clothing is 3, i.e., Po/ Pw = 3. (1 point) c. What is the range for the relative price of clothing in terms of wheat under free trade? (1 point) d. Suppose the world relative price of clothing in terms of wheat under free trade is 1/2, i.e., Pc/Pw = 1/2. Describe the pattern of trade between Home and Foreign. (2 points) e. Show that both Home and Foreign gain from trade at Pc/Pw = 1/2














