****THIRD POST***** NEED HELP SOLVING THE SOLUTION.

Information:

• An all-equity firm
• Earnings After Taxes: $10million
• Market value: 150million
• Shares Outstanding: 100,000
• Tax Rate: 50%
• Debt Borrowed: $60million
• Interest Rate on Debt: 8%
• Shares bought back: 40,000

Question: What should the interest rate on the debt be for the earnings per share effect to disappear.

INITIAL SOLUTION: With borrowing, EBT = (20 - r * 60) mil. Net Income = 0.5 * EBT = (10 - r * 30) mil. New EPS = Net Income / 60,000 = (10 - r * 30) mil / 60,000. If new EPS = 100, then theres no effect on EPS. This implies that (10 - r * 30) mil = 60,000 * 100 = 6 mil Thus, we obtain r = (10 - 6) / 30 = 13.33%

Answer should be 13.33%

NEED HELP SOLVING THE SOLUTION. I am not sure where "30" came from, Please clarify how to solve the equation above step-by-step with explanation. Please make sure I can calculate the correct answer clearly. Thank you.

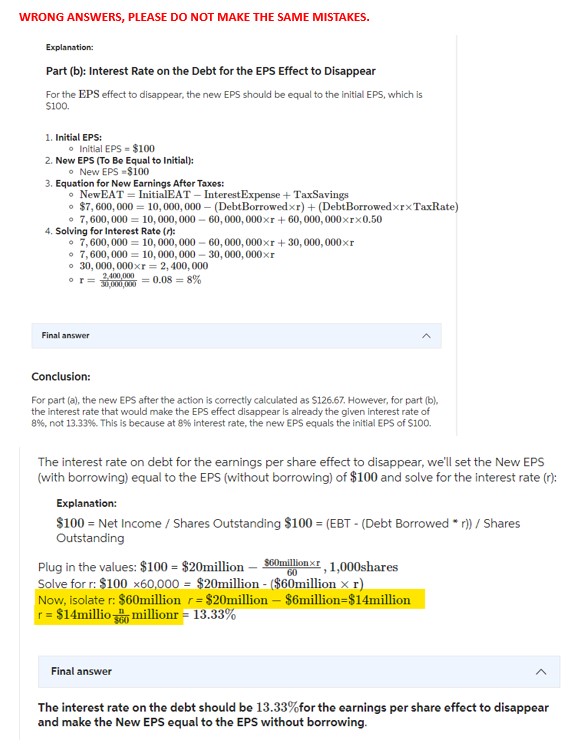
Explanation: Part (b): Interest Rate on the Debt for the EPS Effect to Disappear For the EPS effect to disappear, the new EPS should be equal to the initial EPS, which is $100. 1. Initial EPS: - Initial EPS =$100 2. New EPS (To Be Equal to Initial): - New EPS =$100 3. Equation for Now Earnings After Taxos: - NewEAT = InitialEAT - InterestExpense + TaxSavings - $7,600,000=10,000,000( DebtBorrowed r)+( DebtBorrowed r TaxRate ) - 7,600,000=10,000,00060,000,000r+60,000,000r0.50 4. Solving for Interest Rate (r) : - 7,600,000=10,000,00060,000,000r+30,000,000r - 7,600,000=10,000,00030,000,000r - 30,000,000r=2,400,000 r=30,000,0002,400,000=0.08=8% Final answer Conclusion: For part (a), the new EPS after the action is correctly calculated as \$126.67. However, for part (b), the interest rate that would make the EPS effect disappear is already the given interest rate of 8%, not 13.33%. This is because at 8% interest rate, the new EPS equals the initial EPS of $100. The interest rate on debt for the earnings per share effect to disappear, we'll set the New EPS (with borrowing) equal to the EPS (without borrowing) of $100 and solve for the interest rate ( r ): Explanation: $100= Net Income / Shares Outstanding $100=( EBT ( Debt Borrowed * r ))/ Shares Outstanding Plug in the values: $100=$20 million 60$60millionr,1,000 shares Solve for r : $10060,000=$20 million ($60 million r ) Now, isolate r: $60 million r=$20 million $6 million =$14 million r=$14 millio $60n millionr =13.33% Final answer The interest rate on the debt should be 13.33% for the earnings per share effect to disappear and make the New EPS equal to the EPS without borrowing. Explanation: Part (b): Interest Rate on the Debt for the EPS Effect to Disappear For the EPS effect to disappear, the new EPS should be equal to the initial EPS, which is $100. 1. Initial EPS: - Initial EPS =$100 2. New EPS (To Be Equal to Initial): - New EPS =$100 3. Equation for Now Earnings After Taxos: - NewEAT = InitialEAT - InterestExpense + TaxSavings - $7,600,000=10,000,000( DebtBorrowed r)+( DebtBorrowed r TaxRate ) - 7,600,000=10,000,00060,000,000r+60,000,000r0.50 4. Solving for Interest Rate (r) : - 7,600,000=10,000,00060,000,000r+30,000,000r - 7,600,000=10,000,00030,000,000r - 30,000,000r=2,400,000 r=30,000,0002,400,000=0.08=8% Final answer Conclusion: For part (a), the new EPS after the action is correctly calculated as \$126.67. However, for part (b), the interest rate that would make the EPS effect disappear is already the given interest rate of 8%, not 13.33%. This is because at 8% interest rate, the new EPS equals the initial EPS of $100. The interest rate on debt for the earnings per share effect to disappear, we'll set the New EPS (with borrowing) equal to the EPS (without borrowing) of $100 and solve for the interest rate ( r ): Explanation: $100= Net Income / Shares Outstanding $100=( EBT ( Debt Borrowed * r ))/ Shares Outstanding Plug in the values: $100=$20 million 60$60millionr,1,000 shares Solve for r : $10060,000=$20 million ($60 million r ) Now, isolate r: $60 million r=$20 million $6 million =$14 million r=$14 millio $60n millionr =13.33% Final answer The interest rate on the debt should be 13.33% for the earnings per share effect to disappear and make the New EPS equal to the EPS without borrowing