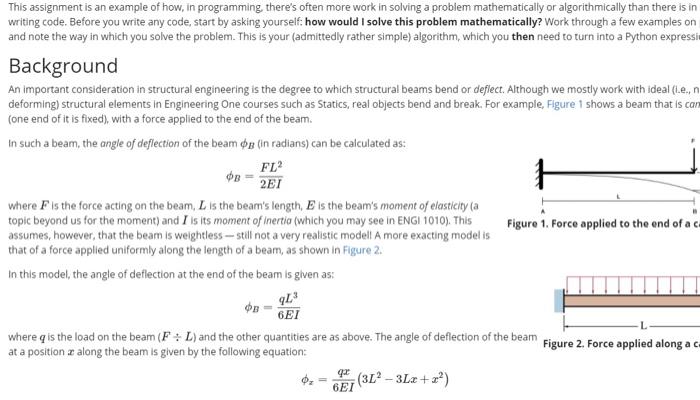
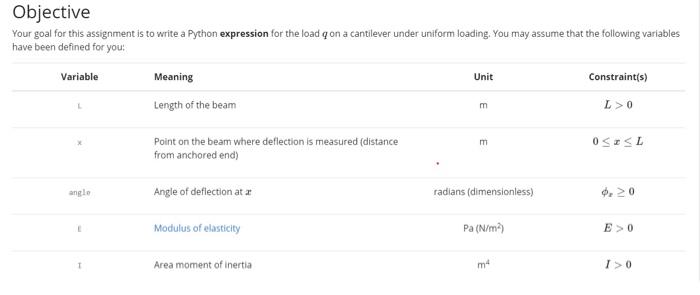
This assignment is an example of how, in programming, there's often more work in solving a problem mathematically or algorithmically than there is in writing code. Before you write any code, start by asking yourself: how would I solve this problem mathematically? Work through a few examples on and note the way in which you solve the problem. This is your (admittedly rather simple) algorithm, which you then need to turn into a Python expressi Background An important consideration in structural engineering is the degree to which structural beams bend or deflect. Although we mostly work with ideal(.e., deforming) structural elements in Engineering One courses such as Statics, real objects bend and break. For example, Figure 1 shows a beam that is can (one end of it is fixed), with a force applied to the end of the beam. In such a beam, the angle of deflection of the beam op (in radians) can be calculated as: 2ET where F is the force acting on the beam, L is the beam's length, E is the beam's moment of elasticity (a topic beyond us for the moment) and I Is its moment of inertia (which you may see in ENGI 1010). This assumes, however, that the beam is weightless - still not a very realistic modell A more exacting model is Figure 1. Force applied to the end of ac that of a force applied uniformly along the length of a beam, as shown in Figure 2. In this model, the angle of deflection at the end of the beam is given as: OL 6EI where is the load on the beam (F : L) and the other quantities are as above. The angle of deflection of the beam at a position along the beam is given by the following equation: Figure 2. Force applied along ac qa oz (3L2 - 3x + x) 6EI Objective Your goal for this assignment is to write a Python expression for the load qon a cantilever under uniform loading. You may assume that the following variables have been defined for you: Variable Meaning Unit Constraints) Length of the beam L> 0 m m OSEL Point on the beam where deflection is measured (distance from anchored end) angle Angle of deflection at a radians (dimensionless) 0.0 Modulus of elasticity Pa (N/m) E>0 1 Area moment of inertia m I > 0