Question
This is a question on determining Volume. Both the QUESTION and SOLUTION are shown below. My Query is related to the resulting function, which the
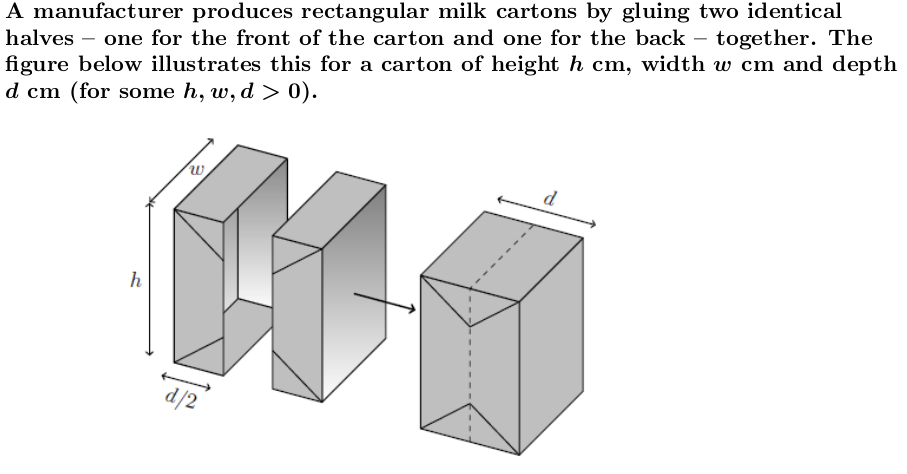
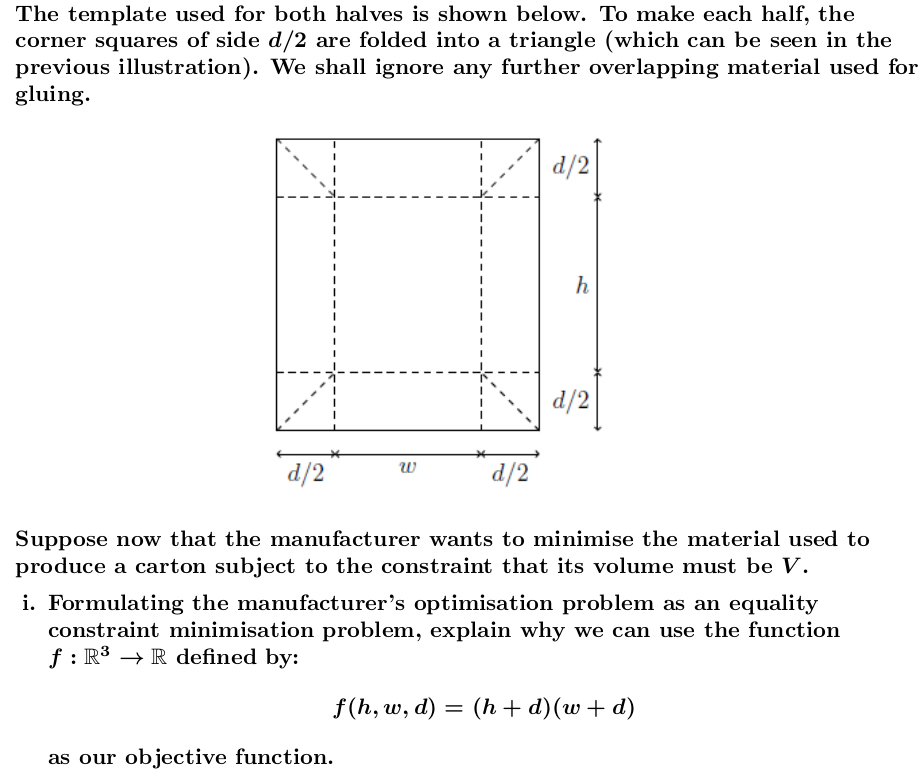

This is a question on determining Volume.
Both the QUESTION and SOLUTION are shown below.
My Query is related to the resulting function, which the SOLUTION shows as correct , highlighted by a RED box, which is
(h+22d)(w+22d)=(h+d)(w+d)
Also,
(h+d)(w+d)=hw+hd+wd+d2
The equation I identified is
[(2d2)wh]2=2(dwh)
since I thought that Volume is height x width x depth.
Please explain how they identified the equation ?
Please explain clearly showing each step as thoroughly as possible. If you are using hand written notes, then please ensure they are neat and legible, as it is difficult to interpret illegible hand-written notes. Alternatively, use LaTex.
QUESTION
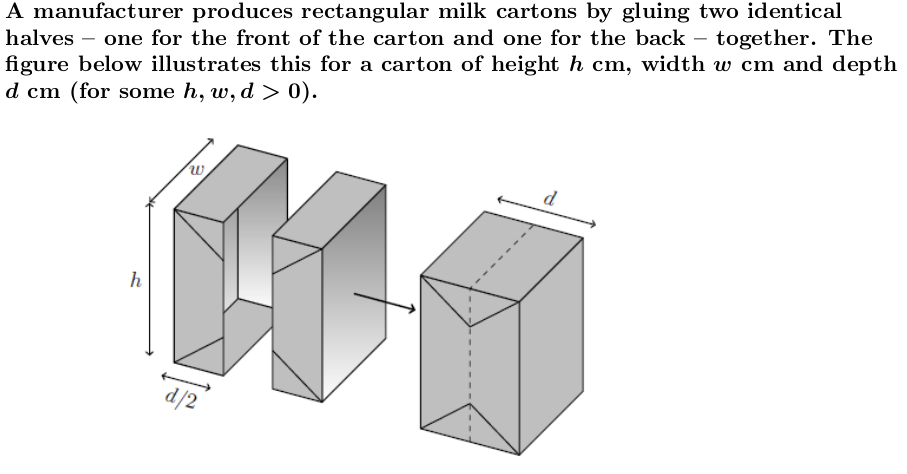


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


