Question
This is an calculus optimization type question. Both the QUESTION and SOLUTION are shown below. My first query relates to the statement 1. strictly
This is an calculus optimization type question.
Both the QUESTION and SOLUTION are shown below.
My first query relates to the statement
1. "strictly greater than M for all M > 0"
The statement is underlined in RED below.
Please show how, in more detail, it can be argued that "this", is greater than M for all M >0
For example, is it possible to say
Mlim(2M210M+25)
since the limit of a polynomial, whose leading coefficient is positive, is infinity.
2. My next query relates to
(M8,M,0)=M264+M2+0>M2M
which is underlined in BLUE below. Please explain the logic/reasoning for this ?
3. My final query relates to all text underlined in GREEN. Explain how
25=(x+y+z)2
is determined and also explain how
(x,y,z)=9
is determined.
Explain the conclusion underlined in GREEN (That is, our constraint set D lies on the 3-sphere .....)
Please explain clearly showing each step as thoroughly as possible. If you are using hand written notes, then please ensure they are neat and legible, as it is difficult to interpret illegible hand-written notes. Alternatively, use LaTex.
QUESTION
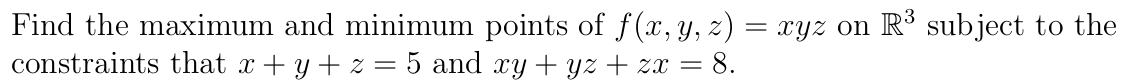

Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


