This is the question "Find the value of a European call option with an expiration of 6 months. The exercise price is $28. The value of the put on the stock with the same exercise price is $15 (with the same expiration date). The share price is $20, and the interest rate is 5% per year, compounded semi-annually."

This is the correct answer Put call parity: C = P+S-PV(K) = 15+20-28/1.025 = 7.68

I want to know an explanation to this answer and how this formula was derived? what base formula was used to derive this? also if its PV times K why do we end up dividing 28 by 1.025 instead of multiplying? Also is this connected to black Scholes formula or any of the formulas below in any way ?

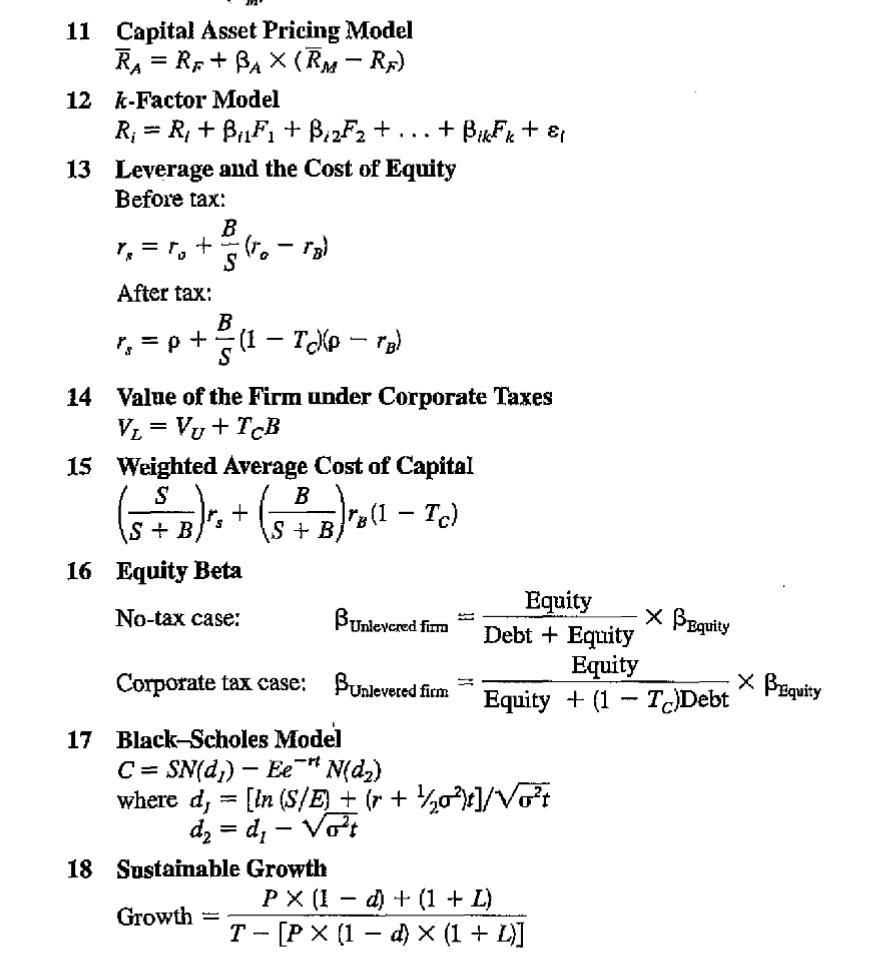
11 Capital Asset Pricing Model RA=RF+A(RMRF) 12k-Factor Model Ri=Ri+i1F1+i2F2++ikFk+i 13 Leverage and the Cost of Equity Before tax: rs=r0+SB(r0rB) After tax: rs=+SB(1TC)(rB) 14 Value of the Firm under Corporate Taxes VL=VU+TCB 15 Weighted Average Cost of Capital (S+BS)rs+(S+BB)rB(1TC) 16 Equity Beta No-tax case: No-taxcase:Corporatetaxcase:Unleveredfirm=Debt+EquityEquityEquityUnleveredfirm=Equity+(1TC)DebtEquityEquity 17 Black-Scholes Model C=SN(d1)EertN(d2)wheredI=[ln(S/E)+(r+1/22)t]/2td2=d12t 18 Sustainable Growth Growth=T[P(1d)(1+L)]P(1d)+(1+L)