Question
This problem combines equilibrium analysis with some of the things you learned in the chapter on intertemporal choice. It concerns the economics of saving and

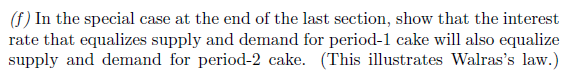
This problem combines equilibrium analysis with some of the things you learned in the chapter on intertemporal choice. It concerns the
economics of saving and the life cycle on an imaginary planet where life is short and simple. In advanced courses in macroeconomics, you would
study more-complicated versions of this model that build in more earthly realism. For the present, this simple model gives you a good idea of how
the analysis must go.
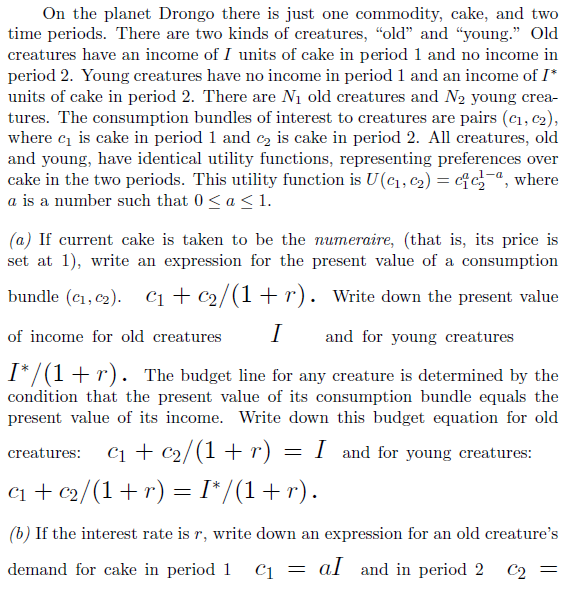
On the planet Drongo there is just one commodity, cake, and two time periods. There are two kinds of creatures, "old" and "young." Old
creatures have an income of I units of cake in period 1 and no income in period 2. Young creatures have no income in period 1 and an income of I?
units of cake in period 2. There are N1 old creatures and N2 young creatures. The consumption bundles of interest to creatures are pairs (c1, c2),
where c1 is cake in period 1 and c2 is cake in period 2. All creatures, old and young, have identical utility functions, representing preferences over
cake in the two periods. This utility function is U(c1, c2) = c1a c21-a , where a is a number such that 0 ? a ? 1.
(a) If current cake is taken to be the numeraire, (that is, its price is set at 1), create an expression for the present value of a consumption bundle
(c1, c2). Write down the present value of income for old creatures and for young creatures__________. The budget line for any creature is determined by the condition that the present value of its consumption bundle equals the present value of its income. Write down this budget equation for old
creatures: ______ and for young creatures:_______
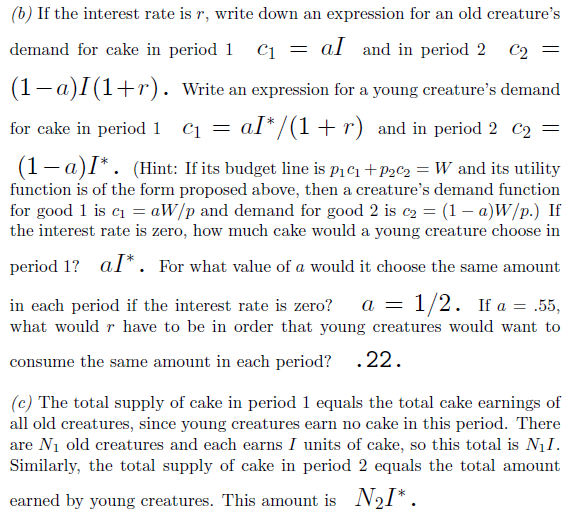
(b) If the interest rate is r, write down an expression for an old creature's demand for cake in period 1 ______ and in period 2 ______. create a expression for a young creature's demand for cake in period 1 _______ and in period 2_____. (Hint: If its budget line is p1c1+p2c2 = W and its utility
function is of the form proposed above, then a creature's demand function for good 1 is c1 = aW/p and demand for good 2 is c2 = (1?a)W/p.) If the interest rate is zero, how much cake would a young creature choose in period 1? _______. For what value of a would it choose the same amount
in each period if the interest rate is zero? ________ If a = .55, what would r have to be in order that young creatures would want to consume the same amount in each period?
(c) The total supply of cake in period 1 equals the total cake earnings of all old creatures, since young creatures earn no cake in this period. There
are N1 old creatures and each earns I units of cake, so this total is N1I. Similarly, the total supply of cake in period 2 equals the total amount
earned by young creatures. This amount is __________
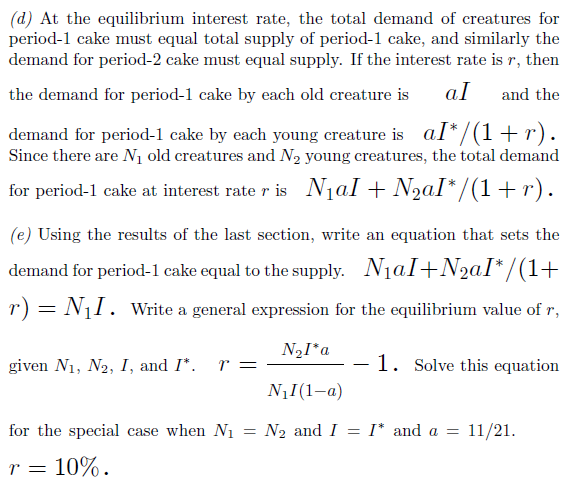
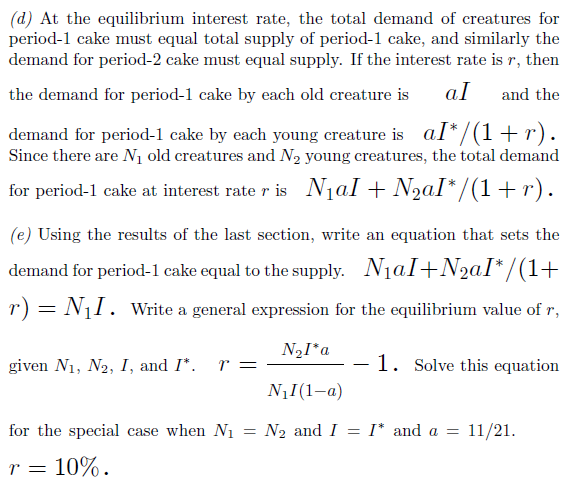
(d) At the equilibrium interest rate, the total demand of creatures for period-1 cake must equal total supply of period-1 cake, and similarly the
demand for period-2 cake must equal supply. If the interest rate is r, then the demand for period-1 cake by each old creature is _____ and the
demand for period-1 cake by each young creature is_______. Since there are N1 old creatures and N2 young creatures, the total demand
for period-1 cake at interest rate r is _________.
(e) Using the results of the last section, create a equation that sets the demand for period-1 cake equal to the supply. _____. create a general expression for the equilibrium value of r, given N1, N2, I, and I?. ________. Solve this equation for the special case when N1 = N2 and I = I? and a = 11/21.
(f ) In the special case at the end of the last section, show that the interest rate that equalizes supply and demand for period-1 cake will also equalize
supply and demand for period-2 cake. (This illustrates Walras's law.)
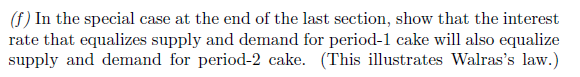
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


