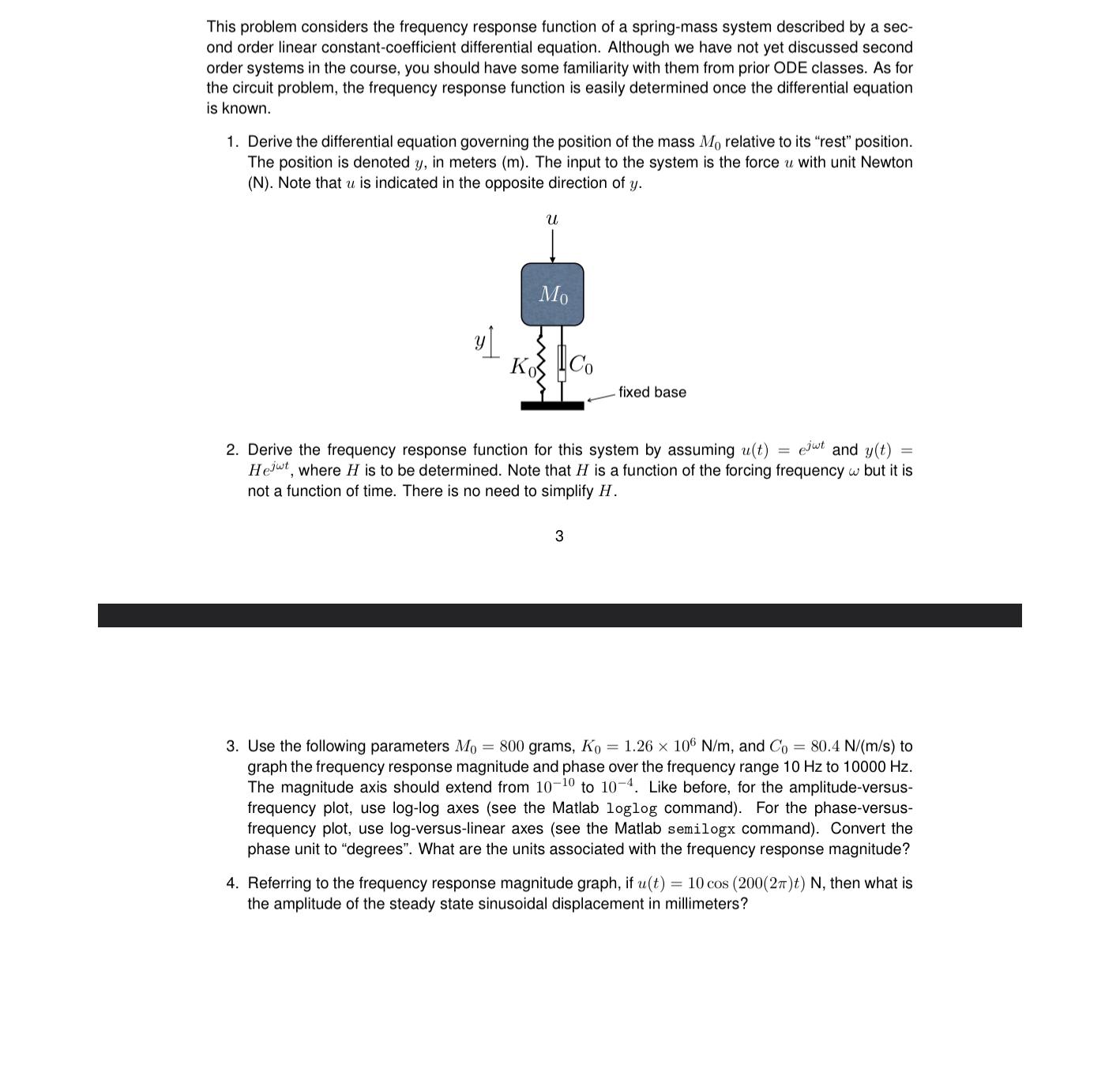
This problem considers the frequency response function of a spring-mass system described by a sec- ond order linear constant-coefficient differential equation. Although we have not yet discussed second order systems in the course, you should have some familiarity with them from prior ODE classes. As for the circuit problem, the frequency response function is easily determined once the differential equation is known. 1. Derive the differential equation governing the position of the mass Mo relative to its "rest" position. The position is denoted y, in meters (m). The input to the system is the force u with unit Newton (N). Note that u is indicated in the opposite direction of y. y] Ko U Mo fixed base 2. Derive the frequency response function for this system by assuming u(t) ejut and y(t) Hejut, where H is to be determined. Note that H is a function of the forcing frequency w but it is not a function of time. There is no need to simplify H. 3 = 3. Use the following parameters Mo = 800 grams, Ko = 1.26 x 106 N/m, and Co = 80.4 N/(m/s) to graph the frequency response magnitude and phase over the frequency range 10 Hz to 10000 Hz. The magnitude axis should extend from 10-10 to 10-4. Like before, for the amplitude-versus- frequency plot, use log-log axes (see the Matlab loglog command). For the phase-versus- frequency plot, use log-versus-linear axes (see the Matlab semilogx command). Convert the phase unit to "degrees". What are the units associated with the frequency response magnitude? 4. Referring to the frequency response magnitude graph, if u(t) = 10 cos (200(2T)t) N, then what is the amplitude of the steady state sinusoidal displacement in millimeters?