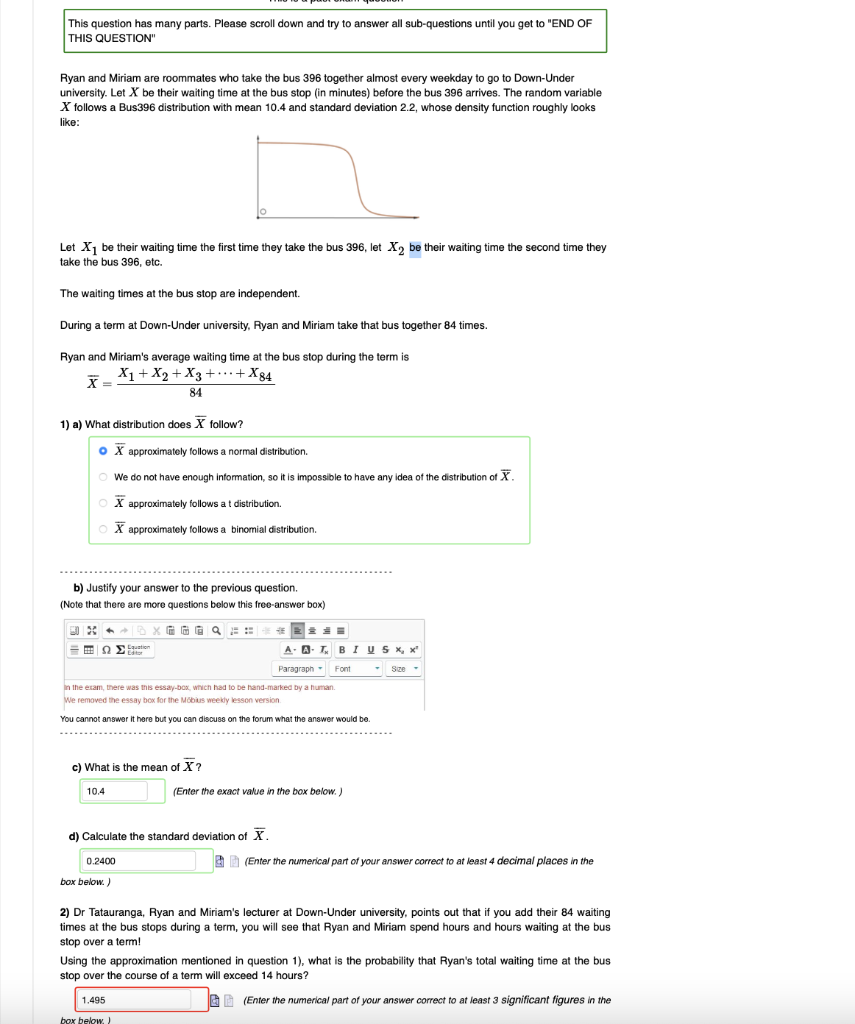
This question has many parts. Please scroll down and try to answer all sub-questions until you get to "END OF THIS QUESTION" Ryan and Miriam are roommates who take the bus 396 together almost every weekday to go to Down-Under university. Let X be their waiting time at the bus stop (in minutes) before the bus 396 arrives. The random variable X follows a Bus396 distribution with mean 10.4 and standard deviation 2.2, whose density function roughly looks like: Let X1 be their waiting time the first time they take the bus 396 , let X2 be their waiting time the second time they take the bus 396 , etc. The waiting times at the bus stop are independent. During a term at Down-Under university, Ryan and Miriam take that bus together 84 times. Ryan and Miriam's average waiting time at the bus stop during the term is X=84X1+X2+X3++X84 1) a) What distribution does X follow? X approximately follows a normal distribution. We do not have enough intormation, so it is impossible to have any idea of the distribution of X. X approximately follows a t distribution. X approximately follows a binomial distribution. b) Justify your answer to the previous question. (Note that there are more questions below this free-answer box) In the exam, there was this essay-bcx, which had to be hand-marked by a human. We removed the essay box for the Mobius weekly kesson version You cannot answer it here but you can discuss on the forum what the answer would be. c) What is the mean of X ? (Enter the exact value in the box below.) d) Calculate the standard deviation of X. (Enter the numerical part of your answer correct to at least 4 decimal places in the box below.) 2) Dr Tatauranga, Ryan and Miriam's lecturer at Down-Under university, points out that if you add their 84 waiting times at the bus stops during a term, you will see that Ryan and Miriam spend hours and hours waiting at the bus stop over a term! Using the approximation mentioned in question 1), what is the probability that Ryan's total waiting time at the bus stop over the course of a term will exceed 14 hours? A (Enter the numerical part of your answer correct to at least 3 significant figures in the This question has many parts. Please scroll down and try to answer all sub-questions until you get to "END OF THIS QUESTION" Ryan and Miriam are roommates who take the bus 396 together almost every weekday to go to Down-Under university. Let X be their waiting time at the bus stop (in minutes) before the bus 396 arrives. The random variable X follows a Bus396 distribution with mean 10.4 and standard deviation 2.2, whose density function roughly looks like: Let X1 be their waiting time the first time they take the bus 396 , let X2 be their waiting time the second time they take the bus 396 , etc. The waiting times at the bus stop are independent. During a term at Down-Under university, Ryan and Miriam take that bus together 84 times. Ryan and Miriam's average waiting time at the bus stop during the term is X=84X1+X2+X3++X84 1) a) What distribution does X follow? X approximately follows a normal distribution. We do not have enough intormation, so it is impossible to have any idea of the distribution of X. X approximately follows a t distribution. X approximately follows a binomial distribution. b) Justify your answer to the previous question. (Note that there are more questions below this free-answer box) In the exam, there was this essay-bcx, which had to be hand-marked by a human. We removed the essay box for the Mobius weekly kesson version You cannot answer it here but you can discuss on the forum what the answer would be. c) What is the mean of X ? (Enter the exact value in the box below.) d) Calculate the standard deviation of X. (Enter the numerical part of your answer correct to at least 4 decimal places in the box below.) 2) Dr Tatauranga, Ryan and Miriam's lecturer at Down-Under university, points out that if you add their 84 waiting times at the bus stops during a term, you will see that Ryan and Miriam spend hours and hours waiting at the bus stop over a term! Using the approximation mentioned in question 1), what is the probability that Ryan's total waiting time at the bus stop over the course of a term will exceed 14 hours? A (Enter the numerical part of your answer correct to at least 3 significant figures in the