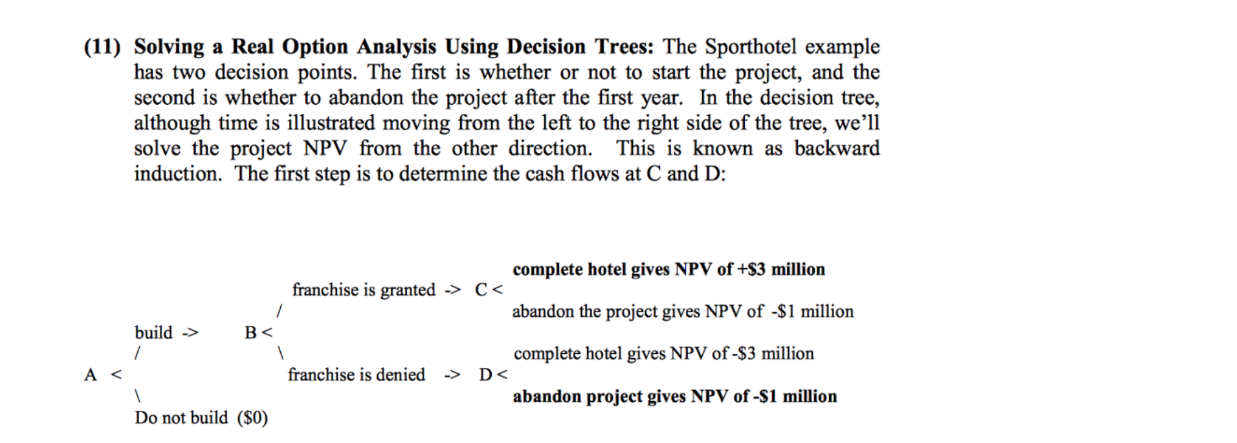
This question is a variant of the Sport Hotel example that was presented in class, in the class notes, and in the Real Option chapter. The change to consider is this: suppose that the value of the hotel is one of two values: $9.6 million if the city is successful in obtaining the franchise (and not $8 million as in the original problem) or $3.6 if the city is not successful in obtaining the franchise (and not $2 million as in the original problem). All other aspects of the problem are the same as originally presented, such as the costs per year. Assume that the probability of obtaining the franchise is 50%. Incorporating these new hotel values from above, and the real option, what is the new NPV of the project? million Place your answer in millions of dollars using at least three decimal places. For example, the answer of nine hundred seventy five thousand would be entered as 0.975 and not as 975000. (9) The Sporthotel - Traditional NPV: Hyatt International is now deciding whether or not to build a hotel next to a soon to be built state-of-the-art hockey arena. The hotel is in a city that is one of three vying for an NHL franchise. Hyatt's plan is for the property to be the official hotel of the team. The NHL will announce their franchise decision in exactly one year. If the franchise is granted, the games will begin two years from that point in time, or three years from today. To be the official hotel, the property must be ready for guests when the first game is played. It takes exactly three years to build the hotel. 2 3 Begin The Hotel Project NHL Makes Franchise Decision Hotel Building Continues Hotel Completed For simplicity sake, we'll remove time value from the analysis by assuming that the discount rate is zero. Unrealistic yes, but the calculations become easier. Question: Does the hotel have a positive or negative NPV? [Answer) With a 50% chance that the city gets the franchise, NPV = $0 1. Projected outflows from the hotel project (all figures in millions of dollars) First year (Purchase Right, Land, and Permits) $1 Second Year (Construct building shell $2 Third Year: (Finish interior and furnishings) $2 TOTAL $5 2. Projected Inflows from the hotel project (all figures in millions of dollars) If the city gets the franchise the hotel will be worth $8 million when it opens. If the city does not get the franchise the hotel will be worth $2 when it opens. 3. Apply some probability that the NHL awards the city the franchise: Try 50% Inflows = 50% * ($8) + 50% * ($2) = $5 Million Outflows = $1 + $2 + $2 = $5 Million NPV = -$5 + $5 = $0 Million With a 50% chance of the city getting the franchise, the NPV of the project is $0, and shareholders would be indifferent between accepting and rejecting the project. The same question could be asked in another way: What chance of getting the franchise would make NPV = $0? Since receiving the franchise results in an NPV of $8 - $5 = $3, and not getting the franchise results in an NPV of $2 - $5 = -$3, then (letting P stand for the probability): P* ($8 - $5) + (1-P) * ($2 - $5) = $0 P*(3) + (1-P)*(-3) = 0 3P - 3+3P = 0 6P = 3 P= 3/6 = 50% Any probability greater than 50% will make the hotel project acceptable, and any probability less than 50% would cause Hyatt to reject the project. (10) The Sporthotel - Real Option Analysis: Suppose that our NPV analysis incorporates the right to abandon the hotel project should the city get bad news. Including abandonment, what probability of the city being awarded the franchise will make this project have a zero NPV? [Answer] 25%. Here's how the abandonment option works. First, pay $1 million for all first year expenses to acquire the rights, plans and land. If after that first year the franchise is granted, then continue the project by building the hotel for another $4 million. Hyatt would then have a +$3 million NPV hotel since the hotel costs $5 million and is worth $8 million. On the other hand, if after one year the franchise is denied, Hyatt should abandon the hotel for a total loss of $1 million. The ability to abandon the project in the event of bad news is the real option in this project. Including the real option, what chance of getting the franchise would make NPV equal to zero? Since being awarded the franchise results in an NPV of $3 million, and abandoning the project if the franchise is not awarded results in an NPV of -$1, then: P* ($8 - $5) + (1-P) * (-$1) = $0 P*3+ (1-P)*-1 = 0 3P-1 + P=0 4P = 1 P=1/4 = 25% Thus, a franchise probability greater than 25% turns into a positive NPV when we include the real option to abandon. (11) Solving a Real Option Analysis Using Decision Trees: The Sporthotel example has two decision points. The first is whether or not to start the project, and the second is whether to abandon the project after the first year. In the decision tree, although time is illustrated moving from the left to the right side of the tree, we'll solve the project NPV from the other direction. This is known as backward induction. The first step is to determine the cash flows at C and D: complete hotel gives NPV of +$3 million franchise is granted -> C B D abandon project gives NPV of -$1 million Do not build ($0) This question is a variant of the Sport Hotel example that was presented in class, in the class notes, and in the Real Option chapter. The change to consider is this: suppose that the value of the hotel is one of two values: $9.6 million if the city is successful in obtaining the franchise (and not $8 million as in the original problem) or $3.6 if the city is not successful in obtaining the franchise (and not $2 million as in the original problem). All other aspects of the problem are the same as originally presented, such as the costs per year. Assume that the probability of obtaining the franchise is 50%. Incorporating these new hotel values from above, and the real option, what is the new NPV of the project? million Place your answer in millions of dollars using at least three decimal places. For example, the answer of nine hundred seventy five thousand would be entered as 0.975 and not as 975000. (9) The Sporthotel - Traditional NPV: Hyatt International is now deciding whether or not to build a hotel next to a soon to be built state-of-the-art hockey arena. The hotel is in a city that is one of three vying for an NHL franchise. Hyatt's plan is for the property to be the official hotel of the team. The NHL will announce their franchise decision in exactly one year. If the franchise is granted, the games will begin two years from that point in time, or three years from today. To be the official hotel, the property must be ready for guests when the first game is played. It takes exactly three years to build the hotel. 2 3 Begin The Hotel Project NHL Makes Franchise Decision Hotel Building Continues Hotel Completed For simplicity sake, we'll remove time value from the analysis by assuming that the discount rate is zero. Unrealistic yes, but the calculations become easier. Question: Does the hotel have a positive or negative NPV? [Answer) With a 50% chance that the city gets the franchise, NPV = $0 1. Projected outflows from the hotel project (all figures in millions of dollars) First year (Purchase Right, Land, and Permits) $1 Second Year (Construct building shell $2 Third Year: (Finish interior and furnishings) $2 TOTAL $5 2. Projected Inflows from the hotel project (all figures in millions of dollars) If the city gets the franchise the hotel will be worth $8 million when it opens. If the city does not get the franchise the hotel will be worth $2 when it opens. 3. Apply some probability that the NHL awards the city the franchise: Try 50% Inflows = 50% * ($8) + 50% * ($2) = $5 Million Outflows = $1 + $2 + $2 = $5 Million NPV = -$5 + $5 = $0 Million With a 50% chance of the city getting the franchise, the NPV of the project is $0, and shareholders would be indifferent between accepting and rejecting the project. The same question could be asked in another way: What chance of getting the franchise would make NPV = $0? Since receiving the franchise results in an NPV of $8 - $5 = $3, and not getting the franchise results in an NPV of $2 - $5 = -$3, then (letting P stand for the probability): P* ($8 - $5) + (1-P) * ($2 - $5) = $0 P*(3) + (1-P)*(-3) = 0 3P - 3+3P = 0 6P = 3 P= 3/6 = 50% Any probability greater than 50% will make the hotel project acceptable, and any probability less than 50% would cause Hyatt to reject the project. (10) The Sporthotel - Real Option Analysis: Suppose that our NPV analysis incorporates the right to abandon the hotel project should the city get bad news. Including abandonment, what probability of the city being awarded the franchise will make this project have a zero NPV? [Answer] 25%. Here's how the abandonment option works. First, pay $1 million for all first year expenses to acquire the rights, plans and land. If after that first year the franchise is granted, then continue the project by building the hotel for another $4 million. Hyatt would then have a +$3 million NPV hotel since the hotel costs $5 million and is worth $8 million. On the other hand, if after one year the franchise is denied, Hyatt should abandon the hotel for a total loss of $1 million. The ability to abandon the project in the event of bad news is the real option in this project. Including the real option, what chance of getting the franchise would make NPV equal to zero? Since being awarded the franchise results in an NPV of $3 million, and abandoning the project if the franchise is not awarded results in an NPV of -$1, then: P* ($8 - $5) + (1-P) * (-$1) = $0 P*3+ (1-P)*-1 = 0 3P-1 + P=0 4P = 1 P=1/4 = 25% Thus, a franchise probability greater than 25% turns into a positive NPV when we include the real option to abandon. (11) Solving a Real Option Analysis Using Decision Trees: The Sporthotel example has two decision points. The first is whether or not to start the project, and the second is whether to abandon the project after the first year. In the decision tree, although time is illustrated moving from the left to the right side of the tree, we'll solve the project NPV from the other direction. This is known as backward induction. The first step is to determine the cash flows at C and D: complete hotel gives NPV of +$3 million franchise is granted -> C B D abandon project gives NPV of -$1 million Do not build ($0)