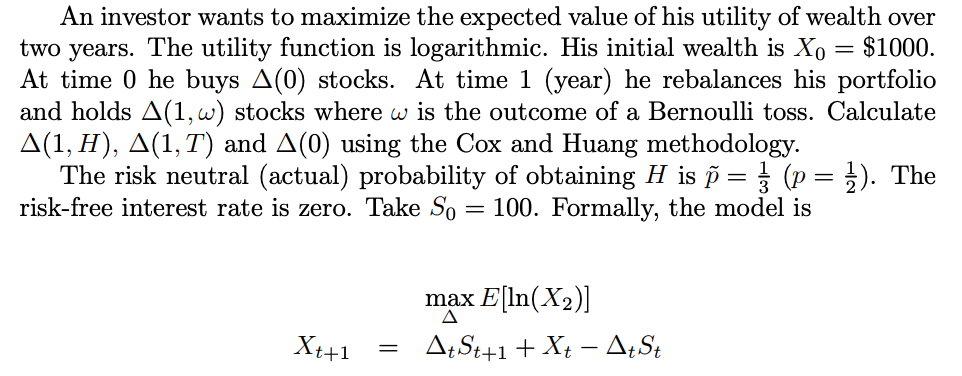
This question is for "Mathematics in Finance". Note that the question is complete and you don't need any other information.

An investor wants to maximize the expected value of his utility of wealth over two years. The utility function is logarithmic. His initial wealth is Xo = $1000. At time 0 he buys A(0) stocks. At time 1 (year) he rebalances his portfolio and holds A(1,w) stocks where w is the outcome of a Bernoulli toss. Calculate A(1,H), A(1, T) and A(0) using the Cox and Huang methodology. The risk neutral (actual) probability of obtaining H is = { (p = 1). The risk-free interest rate is zero. Take So = 100. Formally, the model is max E(ln(X2)] At St+1 + Xt At St Xt+1 = An investor wants to maximize the expected value of his utility of wealth over two years. The utility function is logarithmic. His initial wealth is Xo = $1000. At time 0 he buys A(0) stocks. At time 1 (year) he rebalances his portfolio and holds A(1,w) stocks where w is the outcome of a Bernoulli toss. Calculate A(1,H), A(1, T) and A(0) using the Cox and Huang methodology. The risk neutral (actual) probability of obtaining H is = { (p = 1). The risk-free interest rate is zero. Take So = 100. Formally, the model is max E(ln(X2)] At St+1 + Xt At St Xt+1 =