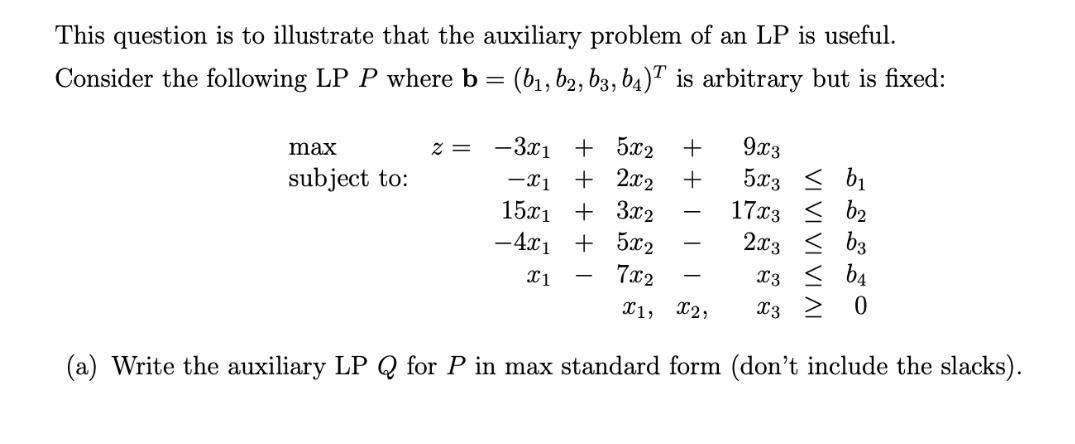
This question is to illustrate that the auxiliary problem of an LP is useful. Consider the following LP P where b = (b,b2, b3, b4)T is arbitrary but is fixed: max subject to: 9x3 5x3 b 17x3 b2 2x3 b3 x3 b4 X3 > 0 (a) Write the auxiliary LP Q for P in max standard form (don't include the slacks). 2 = -3x1 + 5x2 + + 2x2 15x13x2 -X1 + -4x + 5x2 7x2 x1 - X1, X2, (b) If b = (b1,b2, 63, b4) = = (11, 6, 2, 28), find, without performing pivots, a feasible solution X = = (xo|x1, x2, X3 | x4, x5, X6, x7)T for Q (now with slack variables) with x = x = x3 = 0. The solution need not be a basic solution of Q. (c) Do Part (b) again but with b = (-100, -50, -25, -800)T. (d) Do Part (b) again but with b = (0, 20, 5, 20). (e) Is Q feasible for any fixed b? If so, give a feasible solution x = (xo x1, x2, x3 | x4, 5, 6, 7)T for Q in terms of b= (b1, b2, b3, b4) where x = x2 = x3 = 0. If not, find values for b so that its corresponding Q is not feasible. (f) Is the feasible set of Q always bounded? Why or why not? (g) Is the LP Q always bounded? Why or why not? This question is to illustrate that the auxiliary problem of an LP is useful. Consider the following LP P where b = (b, b2, b3, b) is arbitrary but is fixed: max subject to: 9x3 5x3 b 17x3 b2 2x3 b3 x3 b4 X3 > 0 (a) Write the auxiliary LP Q for P in max standard form (don't include the slacks). 2 = -3x1 + 5x2 + + 2x2 15x13x2 -X1 + -4x5x2 x1 - 7x2 X1, X2, (b) If b = (b1,b2, 63, b4) = = (11, 6, 2, 28), find, without performing pivots, a feasible solution X = = (xo|x1, x2, X3 | x4, x5, X6, x7)T for Q (now with slack variables) with x = x = x3 = 0. The solution need not be a basic solution of Q. (c) Do Part (b) again but with b = (-100, -50, -25,-800)T. (d) Do Part (b) again but with b = (0, -20, 5, 20). (e) Is Q feasible for any fixed b? If so, give a feasible solution x = (xo x1, x2, x3 | x4, x5, 6, 7)T for Q in terms of b= (b1, 62, b3, b4) where x = x2 = x3 = 0. If not, find values for b so that its corresponding Q is not feasible. (f) Is the feasible set of Q always bounded? Why or why not? (g) Is the LP Q always bounded? Why or why not?