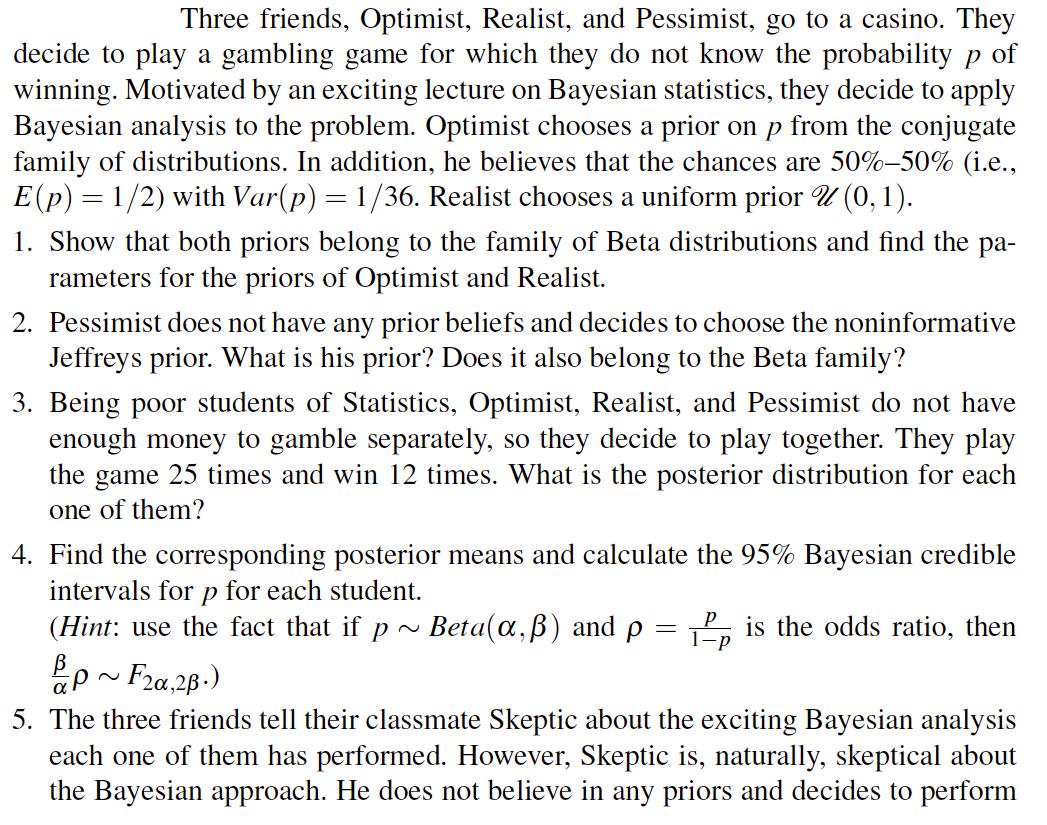
Three friends, Optimist, Realist, and Pessimist, go to a casino. They decide to play a gambling game for which they do not know the probability p of winning. Motivated by an exciting lecture on Bayesian statistics, they decide to apply Bayesian analysis to the problem. Optimist chooses a prior on p from the conjugate family of distributions. In addition, he believes that the chances are 50%-50% (i.e., E(p) = 1/2) with Var(p) = 1/36. Realist chooses a uniform prior (0, 1). 1. Show that both priors belong to the family of Beta distributions and find the pa- rameters for the priors of Optimist and Realist. 2. Pessimist does not have any prior beliefs and decides to choose the noninformative Jeffreys prior. What is his prior? Does it also belong to the Beta family? 3. Being poor students of Statistics, Optimist, Realist, and Pessimist do not have enough money to gamble separately, so they decide to play together. They play the game 25 times and win 12 times. What is the posterior distribution for each one of them? 4. Find the corresponding posterior means and calculate the 95% Bayesian credible intervals for p for each student. (Hint: use the fact that if p ~ Beta(,) and p = p is the odds ratio, then Bp~ F.28-) 1-p 5. The three friends tell their classmate Skeptic about the exciting Bayesian analysis each one of them has performed. However, Skeptic is, naturally, skeptical about the Bayesian approach. He does not believe in any priors and decides to perform a "classical" (non-Bayesian) analysis of the same data. What is his estimate and the 95% confidence interval for p? Compare the results and comment on them.