TOPIC: The pricing of stock and currency derivatives: BlackScholes
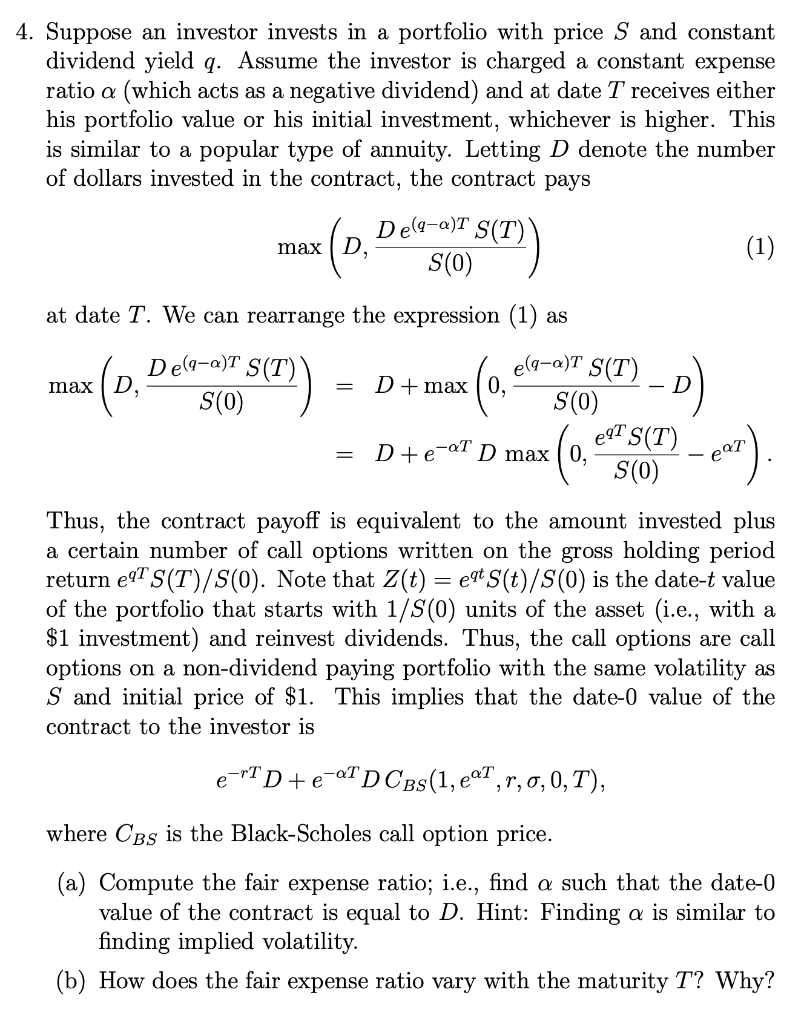
4. Suppose an investor invests in a portfolio with price S and constant dividend yield q. Assume the investor is charged a constant expense ratio a (which acts as a negative dividend) and at date T receives either his portfolio value or his initial investment, whichever is higher. This is similar to a popular type of annuity. Letting D denote the number of dollars invested in the contract, the contract pays Deq-a)T S(T) max (b, Deloslanska) max (1) S(0) at date T. We can rearrange the expression (1) as max (v. De asem sr>) - D+ max (o, cosOSCT) ) - D+2+1 D max (o, carca) cor). Thus, the contract payoff is equivalent to the amount invested plus a certain number of call options written on the gross holding period return e91 S(T)/S(0). Note that Z(t) = eqt S(t)/S(0) is the date-t value of the portfolio that starts with 1/S(0) units of the asset (i.e., with a $1 investment) and reinvest dividends. Thus, the call options are call options on a non-dividend paying portfolio with the same volatility as S and initial price of $1. This implies that the date-0 value of the contract to the investor is e-TD+e-al D Cbs(1, eat,r, 0, 0, T), where Cbs is the Black-Scholes call option price. (a) Compute the fair expense ratio; i.e., find a such that the date-0 value of the contract is equal to D. Hint: Finding a is similar to finding implied volatility. (b) How does the fair expense ratio vary with the maturity T? Why? 4. Suppose an investor invests in a portfolio with price S and constant dividend yield q. Assume the investor is charged a constant expense ratio a (which acts as a negative dividend) and at date T receives either his portfolio value or his initial investment, whichever is higher. This is similar to a popular type of annuity. Letting D denote the number of dollars invested in the contract, the contract pays Deq-a)T S(T) max (b, Deloslanska) max (1) S(0) at date T. We can rearrange the expression (1) as max (v. De asem sr>) - D+ max (o, cosOSCT) ) - D+2+1 D max (o, carca) cor). Thus, the contract payoff is equivalent to the amount invested plus a certain number of call options written on the gross holding period return e91 S(T)/S(0). Note that Z(t) = eqt S(t)/S(0) is the date-t value of the portfolio that starts with 1/S(0) units of the asset (i.e., with a $1 investment) and reinvest dividends. Thus, the call options are call options on a non-dividend paying portfolio with the same volatility as S and initial price of $1. This implies that the date-0 value of the contract to the investor is e-TD+e-al D Cbs(1, eat,r, 0, 0, T), where Cbs is the Black-Scholes call option price. (a) Compute the fair expense ratio; i.e., find a such that the date-0 value of the contract is equal to D. Hint: Finding a is similar to finding implied volatility. (b) How does the fair expense ratio vary with the maturity T? Why







