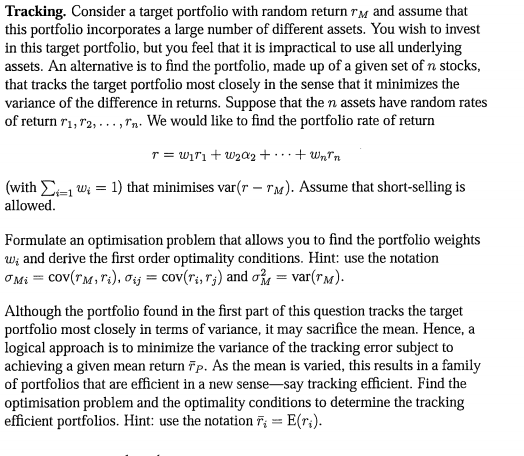
Tracking. Consider a target portfolio with random return rM and assume that this portfolio incorporates a large number of different assets. You wish to invest in this target portfolio, but you feel that it is impractical to use all underlying assets. An alternative is to find the portfolio, made up of a given set of n stocks, that tracks the target portfolio most closely in the sense that it minimizes the variance of the difference in returns. Suppose that the n assets have random rates of return r1, r2, ...,rn. We would like to find the portfolio rate of return T = wiri + W2a2 +... + WnTn (with E-1 Wi = 1) that minimises var(r rm). Assume that short-selling is allowed. Formulate an optimisation problem that allows you to find the portfolio weights W; and derive the first order optimality conditions. Hint: use the notation OMi = cov("m,r&), Oij = cov(ri, r;) and o = var(rm). Although the portfolio found in the first part of this question tracks the target portfolio most closely in terms of variance, it may sacrifice the mean. Hence, a logical approach is to minimize the variance of the tracking error subject to achieving a given mean return #p. As the mean is varied, this results in a family of portfolios that are efficient in a new sense-say tracking efficient. Find the optimisation problem and the optimality conditions to determine the tracking efficient portfolios. Hint: use the notation T; = E(r;). Tracking. Consider a target portfolio with random return rM and assume that this portfolio incorporates a large number of different assets. You wish to invest in this target portfolio, but you feel that it is impractical to use all underlying assets. An alternative is to find the portfolio, made up of a given set of n stocks, that tracks the target portfolio most closely in the sense that it minimizes the variance of the difference in returns. Suppose that the n assets have random rates of return r1, r2, ...,rn. We would like to find the portfolio rate of return T = wiri + W2a2 +... + WnTn (with E-1 Wi = 1) that minimises var(r rm). Assume that short-selling is allowed. Formulate an optimisation problem that allows you to find the portfolio weights W; and derive the first order optimality conditions. Hint: use the notation OMi = cov("m,r&), Oij = cov(ri, r;) and o = var(rm). Although the portfolio found in the first part of this question tracks the target portfolio most closely in terms of variance, it may sacrifice the mean. Hence, a logical approach is to minimize the variance of the tracking error subject to achieving a given mean return #p. As the mean is varied, this results in a family of portfolios that are efficient in a new sense-say tracking efficient. Find the optimisation problem and the optimality conditions to determine the tracking efficient portfolios. Hint: use the notation T; = E(r;)