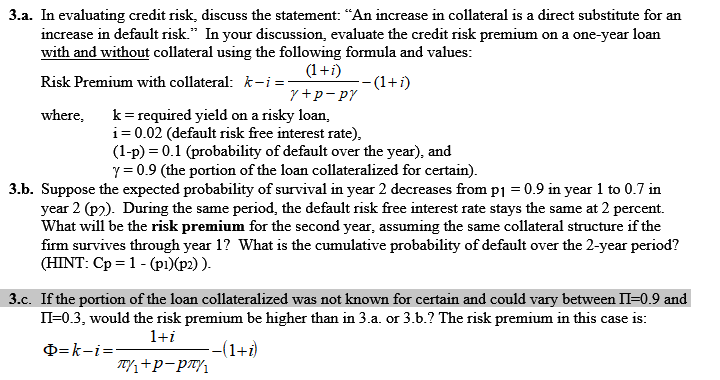
3.a. In evaluating credit risk, discuss the statement: An increase in collateral is a direct substitute for an increase in default risk. In your discussion, evaluate the credit risk premium on a one-year loan with and without collateral using the following formula and values: (1+i) Risk Premium with collateral: k-i== --(1+i) 7+p-py where, k=required yield on a risky loan, i= 0.02 (default risk free interest rate), (1-p)=0.1 (probability of default over the year), and y=0.9 (the portion of the loan collateralized for certain). 3.b. Suppose the expected probability of survival in year 2 decreases from p1 = 0.9 in year 1 to 0.7 in year 2 (p). During the same period, the default risk free interest rate stays the same at 2 percent. What will be the risk premium for the second year, assuming the same collateral structure if the firm survives through year 1? What is the cumulative probability of default over the 2-year period? (HINT: Cp = 1 - (p1)(p2)). 3.c. If the portion of the loan collateralized was not known for certain and could vary between II=0.9 and II=0.3, would the risk premium be higher than in 3.a. or 3.b.? The risk premium in this case is: 1+i =k-i= --(1+i) TT/1+P-PT/ 3.a. In evaluating credit risk, discuss the statement: An increase in collateral is a direct substitute for an increase in default risk. In your discussion, evaluate the credit risk premium on a one-year loan with and without collateral using the following formula and values: (1+i) Risk Premium with collateral: k-i== --(1+i) 7+p-py where, k=required yield on a risky loan, i= 0.02 (default risk free interest rate), (1-p)=0.1 (probability of default over the year), and y=0.9 (the portion of the loan collateralized for certain). 3.b. Suppose the expected probability of survival in year 2 decreases from p1 = 0.9 in year 1 to 0.7 in year 2 (p). During the same period, the default risk free interest rate stays the same at 2 percent. What will be the risk premium for the second year, assuming the same collateral structure if the firm survives through year 1? What is the cumulative probability of default over the 2-year period? (HINT: Cp = 1 - (p1)(p2)). 3.c. If the portion of the loan collateralized was not known for certain and could vary between II=0.9 and II=0.3, would the risk premium be higher than in 3.a. or 3.b.? The risk premium in this case is: 1+i =k-i= --(1+i) TT/1+P-PT/