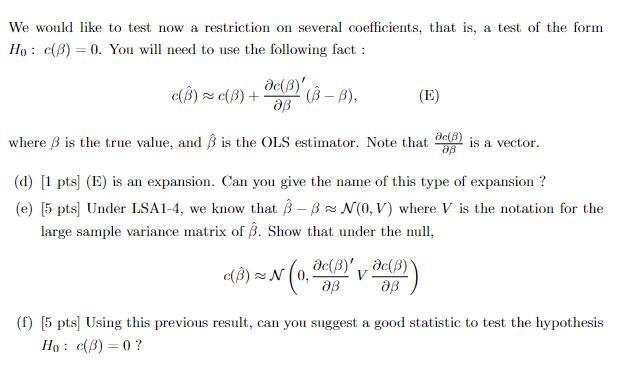
6. [25 pts] Exercise Consider the linear regression with three regressors Yi=1X1i+2X2i+3X3i+ui=Xi+ui, where =123 and Xi=X1iX2iX3i (a) [3 pts] You would like to test whether all coefficients are zero, i.e, 1=0,2=0 and 3=0. What is a naive approach to this test? Explain in a sentence why one should not use this approach. (b) [6 pts] The null hypothesis of this test can be written R=r where R is a matrix and r is a vector. i. [2 pts] What are R and r ? ii. [2 pts] We can use the Wald statistic to test this assumption. What is its distribution in large samples ? iii. [2 pts] Let us call this statistic W. For a given sample you obtain W=12. Do you reject the hypothesis at the 5% and at the 1% confidence level? Explain why. This method can be used only to test linear hypotheses, that is, hypotheses H0 that you can write as R=r. In some cases, you may want to test nonlinear hypotheses, that is, hypotheses of the form H0:c()=0 where c is a function and c() is a scalar number. For example, you can have c()=log(1)+2 and test H0:log(1)+2=0. We will now use tools that we studied in class to help us test nonlinear hypotheses. (c) [5 pts] Let us first look at a nonlinear restriction on only one coefficient. Suggest a test at the 5% confidence level for the hypothesis H0:exp(1)=2. We would like to test now a restriction on several coefficients, that is, a test of the form H0:c()=0. You will need to use the following fact : c(^)c()+c()(^), where is the true value, and ^ is the OLS estimator. Note that c() is a vector. (d) [1 pts] (E) is an expansion. Can you give the name of this type of expansion? (e) [5 pts] Under LSA1-4, we know that ^N(0,V) where V is the notation for the large sample variance matrix of ^. Show that under the null, c(^)N(0,c()Vc()) (f) [5 pts] Using this previous result, can you suggest a good statistic to test the hypothesis H0:c()=0 ? You might need the following formulae. Least Squares Assumptions For the multiple regression Yi=0+1X1i+2X2i++kXki+ui=Xi+ui,i=1,,n, the four Least Squares Assumptions are LSA1. Zero Correlation E(Xiui)=0, LSA2. No perfect multicollinearity, LSA3. (Xi,Yi),i=1,,n are i.i.d, LSA4. Large outliers are unlikely: X1,X2,,Xk and Y have finite fourth order moments, that is, E(X1i4)