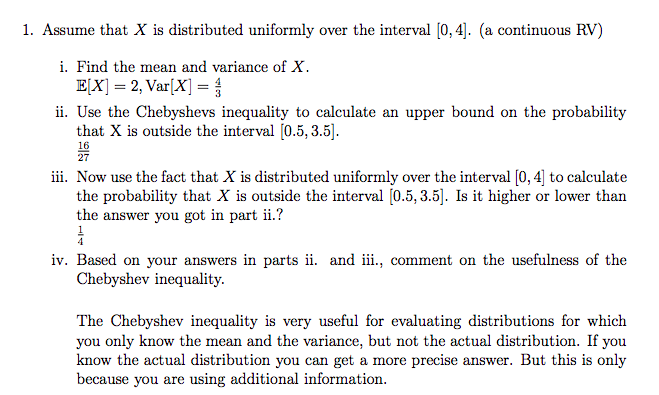
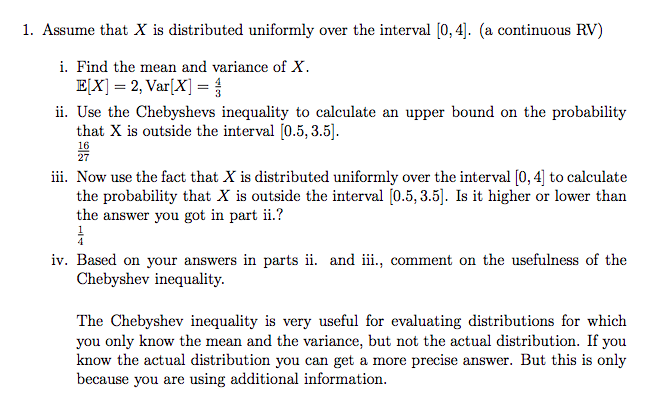
Question
TVs are employed out by a rental organization. The time X in months between major fixes has a remarkable circulation with mean 20 months. I.

TVs are employed out by a rental organization. The time X in months between major
fixes has a remarkable circulation with mean 20 months.
I. Find, to 3 huge figures, the likelihood that a TV recruited out by the
organization won't need a significant fix for in any event long term period.
ii. Track down the middle of X.
iii. The organization consents to substitute any set for which the time between significant fixes
is not exactly M months. In any case, the organization would not like to supplant more than
one set in five such sets. Discover the number worth that ought to be fixed for M.
@24@
A study of families uncovered that 58% of all families eat turkey at occasion suppers, 44% eat ham, and 16% have both turkey and ham to eat at occasion dinners. The graph beneath shows this data.
The issue says that 58% of families eat turkey at occasion suppers, so for what reason does the chart show 42% - what does 42% address?
Discover the likelihood that a haphazardly chosen family eats turkey or ham at occasion dinners.
A public report found that treating individuals suitably for hypertension diminished their general mortality by 20%. Treating individuals satisfactorily for hypertension has been troublesome on the grounds that it is assessed that half of hypertensives don't realize they have hypertension, half of the individuals who do know are insufficiently treated by their doctors, and half who are properly treated neglect to follow this treatment by taking the correct number of pills.
On the off chance that the first half rates were each diminished to 40% by enormous training program, at that point what impact would this change have on the general death rate among genuine hypertensives; that is, would the death rate decline and, assuming this is the case, which level of passings among hypertensives could be forestalled by the schooling program?
In a class of 130 SHS understudies, 41 offers agrarian science, 62 offers business and 31 offer visual workmanship. 16 of these understudies offer both agrarian science and business just and 14 offers both business and visual expressions as it were. Expecting none of the understudies offers every one of the three subjects.
I) what is the likelihood of choosing an understudy indiscriminately from the class who offers neither of the subject



Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


