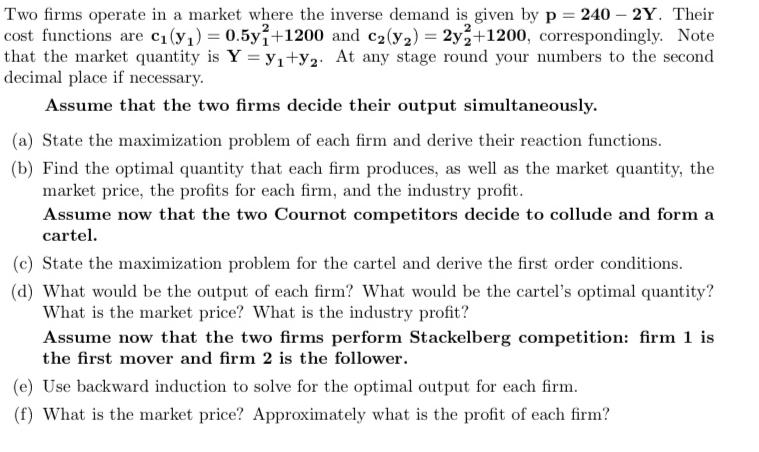
Two firms operate in a market where the inverse demand is given by p = 240-2Y. Their cost functions are c (y) = 0.5y+1200 and c2(y2) = 2y2+1200, correspondingly. Note that the market quantity is Y = y+y2. At any stage round your numbers to the second decimal place if necessary. Assume that the two firms decide their output simultaneously. (a) State the maximization problem of each firm and derive their reaction functions. (b) Find the optimal quantity that each firm produces, as well as the market quantity, the market price, the profits for each firm, and the industry profit. Assume now that the two Cournot competitors decide to collude and form a cartel. (c) State the maximization problem for the cartel and derive the first order conditions. (d) What would be the output of each firm? What would be the cartel's optimal quantity? What is the market price? What is the industry profit? Assume now that the two firms perform Stackelberg competition: firm 1 is the first mover and firm 2 is the follower. (e) Use backward induction to solve for the optimal output for each firm. (f) What is the market price? Approximately what is the profit of each firm?