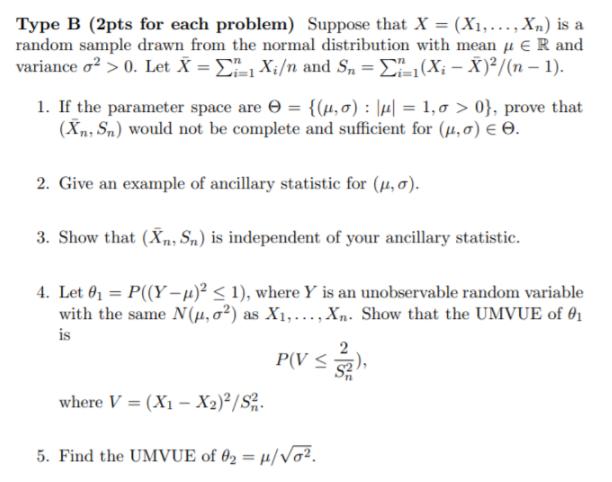
Type B (2pts for each problem) Suppose that X = (X,...,Xn) is a random sample drawn from the normal distribution with mean R and variance o > 0. Let X = 1 Xi/n and Sn = (X-X)/(n-1). 1. If the parameter space are = {(0): || = 1,0 >0}, prove that (Xn, Sn) would not be complete and sufficient for (,0) 0. 2. Give an example of ancillary statistic for (, ). 3. Show that (Xn, Sn) is independent of your ancillary statistic. 4. Let 0 = P((Y-)2 < 1), where Y is an unobservable random variable with the same N(, o2) as X,..., Xn. Show that the UMVUE of 01 is where V = (X X2)/S. 2 P(V2), 5. Find the UMVUE of 02 = /.