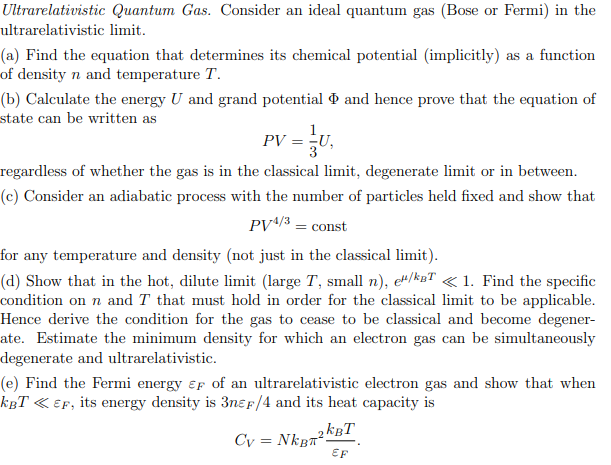
Ultrarelativistic Quantum Gas. Consider an ideal quantum gas (Bose or Fermi) in the ultrarelativistic limit. (a) Find the equation that determines its chemical potential (implicitly) as a function of density n and temperature T. (b) Calculate the energy U and grand potential and hence prove that the equation of state can be written as PV==U, U regardless of whether the gas is in the classical limit, degenerate limit or in between. (c) Consider an adiabatic process with the number of particles held fixed and show that PV4/3 = const for any temperature and density (not just in the classical limit). (d) Show that in the hot, dilute limit (large T, small n), e#/BT