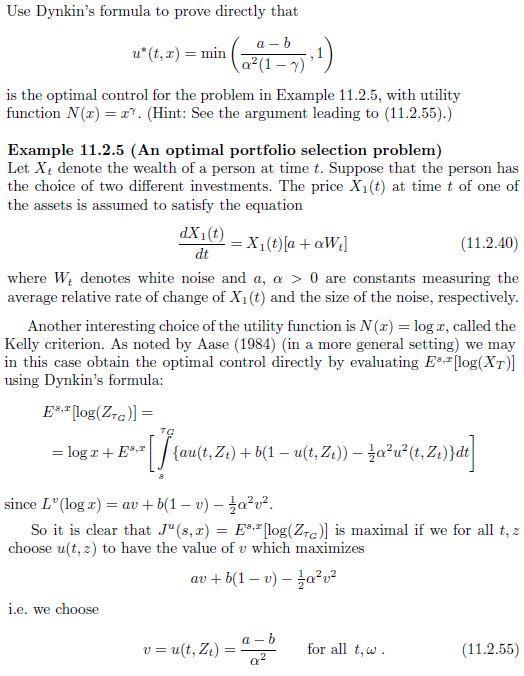
Use Dynkin's formula to prove directly that u* (t, x) = min a-b a (1-7) is the optimal control for the problem in Example 11.2.5, with utility function N(x) = 27. (Hint: See the argument leading to (11.2.55).) Example 11.2.5 (An optimal portfolio selection problem) Let X, denote the wealth of a person at time t. Suppose that the person has the choice of two different investments. The price X (t) at time t of one of the assets is assumed to satisfy the equation dX(t) dt = X (t) [a+aW] (11.2.40) where W denotes white noise and a, a > 0 are constants measuring the average relative rate of change of X (t) and the size of the noise, respectively. E. [log(ZTG)] = b.1) Another interesting choice of the utility function is N(x) = log.x, called the Kelly criterion. As noted by Aase (1984) (in a more general setting) we may in this case obtain the optimal control directly by evaluating Es. [log(XT)] using Dynkin's formula: TO = logr+E8,2 = [] {au(t, Zi) + b(1 = u(t, Zi)) au (tZ)) - t. Ze)}de] since L (log x) = av + b(1 v) av. - i.e. we choose So it is clear that J"(s,x) = Es [log(Za)] is maximal if we for all t, z choose u(t, 2) to have the value of u which maximizes av + b(1-v) - av v = u(t, Z) for all t,w. (11.2.55)