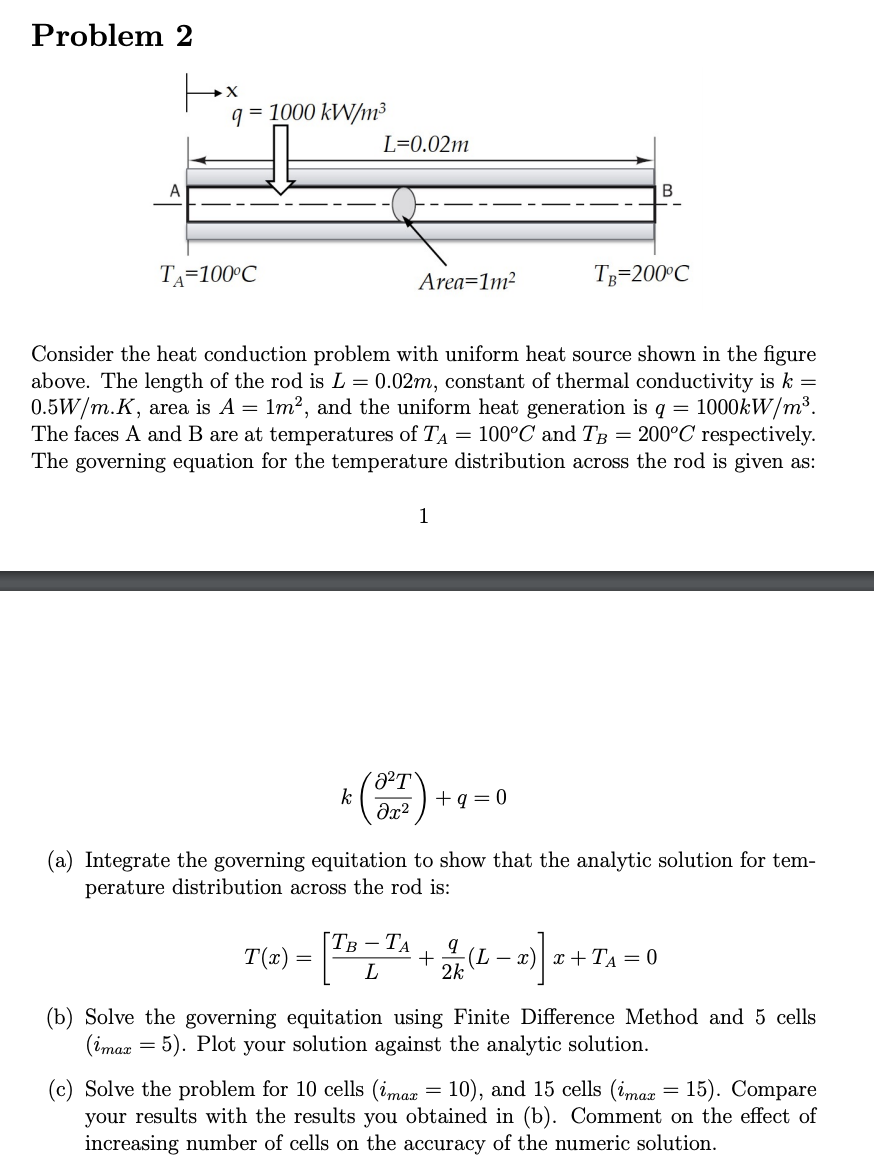
Use Python codes to solve

Problem 2 9 = 1000 kW/m3 L=0.02m TA=100C Area=1m2 Te=200C Consider the heat conduction problem with uniform heat source shown in the figure above. The length of the rod is L= 0.02m, constant of thermal conductivity is k = 0.5W/m.K, area is A = 1m, and the uniform heat generation is q = 1000kW/m3. The faces A and B are at temperatures of TA = 100C and TB = 200C respectively. The governing equation for the temperature distribution across the rod is given as: (a) Integrate the governing equitation to show that the analytic solution for tem- perature distribution across the rod is: T(a) = [To ; TA + (1 - 2) +TA=0 (b) Solve the governing equitation using Finite Difference Method and 5 cells (imax = 5). Plot your solution against the analytic solution. (c) Solve the problem for 10 cells (imar = 10), and 15 cells (imax = 15). Compare your results with the results you obtained in (b). Comment on the effect of increasing number of cells on the accuracy of the numeric solution. Problem 2 9 = 1000 kW/m3 L=0.02m TA=100C Area=1m2 Te=200C Consider the heat conduction problem with uniform heat source shown in the figure above. The length of the rod is L= 0.02m, constant of thermal conductivity is k = 0.5W/m.K, area is A = 1m, and the uniform heat generation is q = 1000kW/m3. The faces A and B are at temperatures of TA = 100C and TB = 200C respectively. The governing equation for the temperature distribution across the rod is given as: (a) Integrate the governing equitation to show that the analytic solution for tem- perature distribution across the rod is: T(a) = [To ; TA + (1 - 2) +TA=0 (b) Solve the governing equitation using Finite Difference Method and 5 cells (imax = 5). Plot your solution against the analytic solution. (c) Solve the problem for 10 cells (imar = 10), and 15 cells (imax = 15). Compare your results with the results you obtained in (b). Comment on the effect of increasing number of cells on the accuracy of the numeric solution