Answered step by step
Verified Expert Solution
Question
1 Approved Answer
Use python! The actuaries for an insurance company represent the total losses experienced during a week as follows: Let N represent the number of policies
Use python!
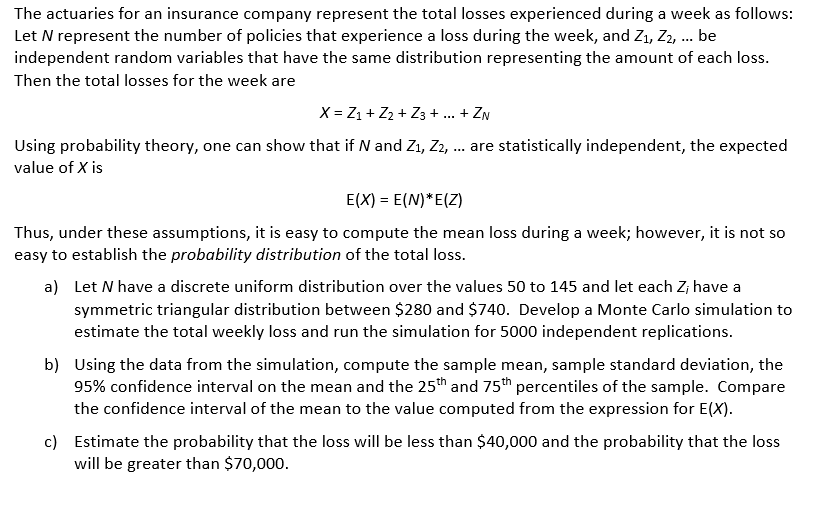
The actuaries for an insurance company represent the total losses experienced during a week as follows: Let N represent the number of policies that experience a loss during the week, and Z1, Z2,. be independent random variables that have the same distribution representing the amount of each loss. Then the total losses for the week are X=Z1 + Z2 + Z3 + + ZN Using probability theory, one can show that if N and Zi, Z2,. are statistically independent, the expected value of X is E(X) E(N) E(Z) Thus, under these assumptions, it is easy to compute the mean loss during a week; however, it is not so easy to establish the probability distribution of the total loss. a) Let N have a discrete uniform distribution over the values 50 to 145 and let each Z, have a symmetric triangular distribution between $280 and $740. Develop a Monte Carlo simulation to estimate the total weekly loss and run the simulation for 5000 independent replications. Using the data from the simulation, compute the sample mean, sample standard deviation, the 95% confidence interval on the mean and the 25th and 75th percentiles of the sample. Compare the confidence interval of the mean to the value computed from the expression for E(X) Estimate the probability that the loss will be less than $40,000 and the probability that the loss will be greater than $70,000. b) c) The actuaries for an insurance company represent the total losses experienced during a week as follows: Let N represent the number of policies that experience a loss during the week, and Z1, Z2,. be independent random variables that have the same distribution representing the amount of each loss. Then the total losses for the week are X=Z1 + Z2 + Z3 + + ZN Using probability theory, one can show that if N and Zi, Z2,. are statistically independent, the expected value of X is E(X) E(N) E(Z) Thus, under these assumptions, it is easy to compute the mean loss during a week; however, it is not so easy to establish the probability distribution of the total loss. a) Let N have a discrete uniform distribution over the values 50 to 145 and let each Z, have a symmetric triangular distribution between $280 and $740. Develop a Monte Carlo simulation to estimate the total weekly loss and run the simulation for 5000 independent replications. Using the data from the simulation, compute the sample mean, sample standard deviation, the 95% confidence interval on the mean and the 25th and 75th percentiles of the sample. Compare the confidence interval of the mean to the value computed from the expression for E(X) Estimate the probability that the loss will be less than $40,000 and the probability that the loss will be greater than $70,000. b) c)
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


