Use the data in housingdata.xls to answer the following questions. 

(a) Consider a simple linear regression model to explain the house price in terms of square footage: price = β0 + β1sqrf t + u 

Estimate this model by OLS and interpret the estimated effect of sqrf t on price? Is the estimated coefficient statistically significant at the 5% significance level against a two-sided alternative? Construct a 95% confidence interval. 

(b) Now, consider a multiple linear regression model: price = α0 + α1sqrf t + α2bdrms + α3lotsize + v 

i. Estimate this model by OLS and interpret the estimated effects of number of bedrooms (bdrms) and size of lot (lotsize) on house price (price). Are these two variables statistically significant at the 5% significance level? 

ii. Test H0 : α2 = α3 against a two-sided alternative. Do you reject H0 at the 5% significance level? iii. Is the estimated effect of sqrf t on price substantially different from the regression that excludes bdrms and lotsize? Does the regression in (a) seem to suffer from omitted variable bias? Explain. 

iv. Explain why se(βˆ 1) can be smaller or greater than se( ˆα1). [se stands for standard errors] (c) Now, estimate the following two linear regression models price = γ0 + γ1sqrf t + γ2lotsize + w lotsize = κ0 + κ1sqrf t + z 2 Assignment 

2 i. What is the variance inflation factor for the slope coefficients in the first model? 

Do you think there is little, moderate or strong collinearity between sqrf t and lotsize? ii. Show that βˆ 1 = ˆγ1 + ˆγ2κˆ1 where βˆ 1 is the estimated coefficient of sqrf t on price (comes from question(a)).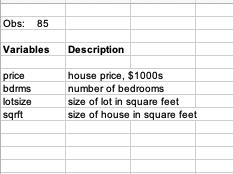


   

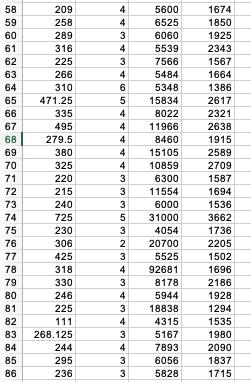
Obs: 85 Variables price bdrms lotsize sqrft Description house price, $1000s number of bedrooms size of lot in square feet size of house in square feet