1. Use the table given below to answer the following questions:

Table: Production and Pricing

Quantity Produced	Total Cost ($)	Total Revenue ($)
0	10	0
1	12	8
2	16	15
3	22	21
4	30	24
5	40	25

1. Calculate the price associated with selling this product. Does the table depict a monopolist or a competitive firm? Explain your reasoning clearly.
2. Find the profit maximizing output and the price associated with selling it in the market.
3. Discuss if the production and pricing decision is welfare maximizing or not.

1. Consider the possibility of building a bridge across a river. The bridge would cost $2 million to build (FC=$2 million) and nothing to maintain (MC=0). The following table given below illustrates the potential demand over the lifetime of the bridge:

Price	Quantity (in Thousands)
$8	0
7	100
6	200
5	300
4	400
3	500
2	600
1	700
0	800

1. What would be the profit-maximizing price that a company would charge? Would that be the efficient level of output? Why or why not? [Hint: Think of output in this case as the number of crossings across the bridge]

1. Should the company build this bridge if it was interested in maximizing profit? Why or why not? [Hint: Find out whether it makes profit or loss upon building]

1. If the government were to build the bridge, what price should it charge?

1. Should the government build this bridge?

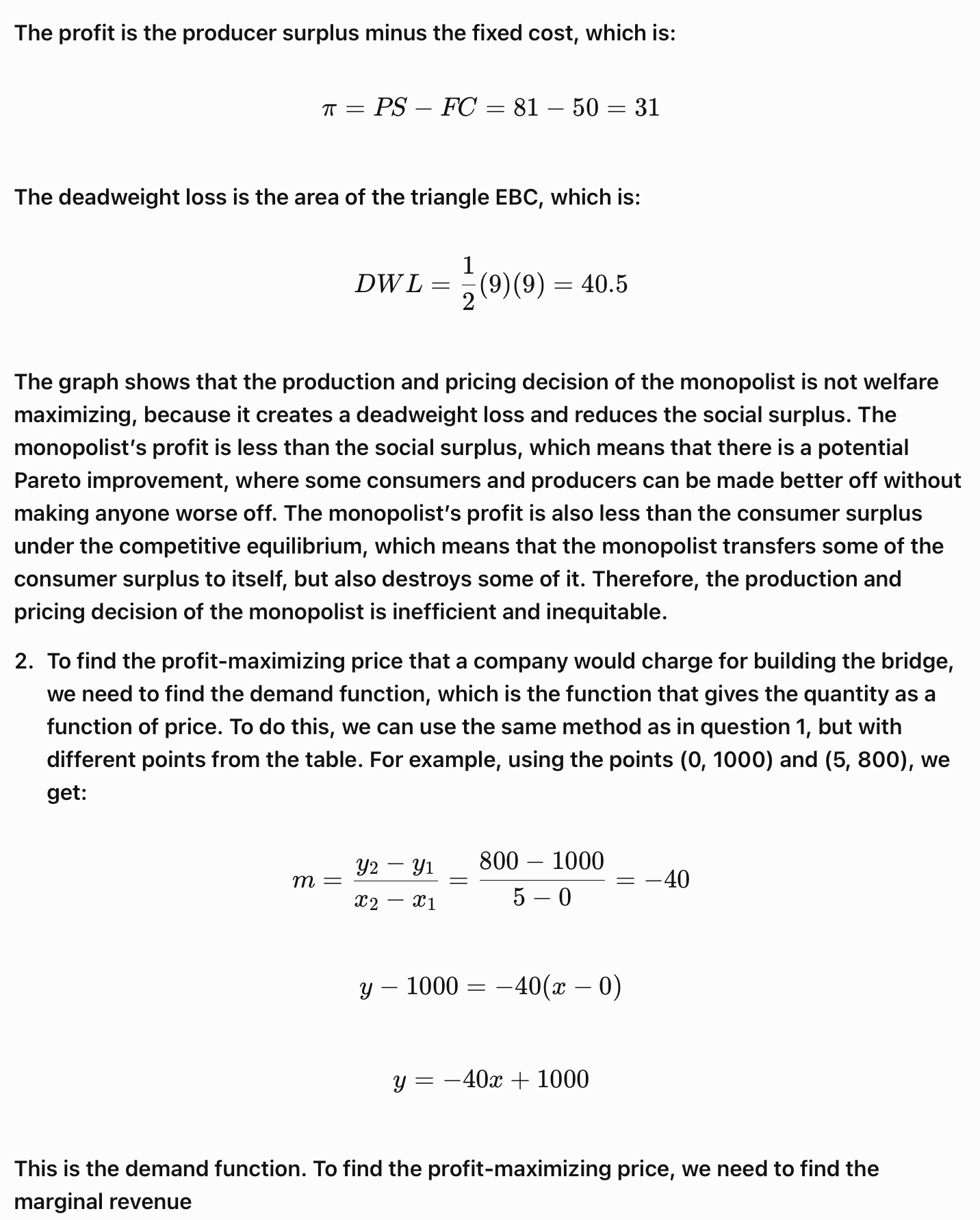The question you posted is about microeconomics. It involves analyzing the production and pricing decisions of a firm and the social welfare implications of those decisions. It also involves evaluating the feasibility and desirability of a public project. The question requires you to use some basic concepts of microeconomics, such as marginal cost, marginal revenue, profit, consumer surplus, producer surplus, deadweight loss, and social surplus. You can find some useful information and examples of these concepts in the following sources: . Principles of Microeconomics - OpenStax: This is an open-source textbook that covers the fundamental topics of microeconomics, such as supply and demand, elasticity, consumer and producer surplus, market structures, market failures, and public goods. . Microeconomics Khan Academy: This is a collection of online videos and exercises that explain the core concepts and applications of microeconomics, such as marginal analysis, market equilibrium, efficiency and equity, market power, and externalities. . Microeconomics - MIT OpenCourseWare: This is a course that provides a rigorous introduction to microeconomic theory and analysis, such as consumer and producer theory, competitive and imperfectly competitive markets, welfare economics, and public goods. Using the information from these sources, I can help you answer the questions. Here are the solutions for the questions: 1. To calculate the price associated with selling this product, we need to find the inverse demand function, which is the function that gives the price as a function of quantity. To do this, we can use the point-slope form of a linear function, which is: 3191:?\"(13931) where y is the price, :1: is the quantity, m is the slope, and ($1,311) is a point on the function. We can use any two points from the table to find the slope and the function. For example, using the points (0, 20) and (10, 10), we get: _ y2_y1 _1020_ 3327331 1070 m y20:1(;c0) y=m+20 This is the inverse demand function. To find the price for any quantity, we can plug in the value of m into the function. For example, to find the price for 30 units, we get: y:*%+20:im This means that the price is negative, which is not possible. This implies that the demand for this product is zero beyond 20 units. Therefore, the price associated with selling this product is given by: :r:l2O ifgm20 y: 0 m>20 The table depicts a monopolist, not a competitive firm. This is because a monopolist is a single seller that faces the downward-sloping market demand curve, and can choose the quantity and the price that maximizes its profit. A competitive firm is one of many sellers that faces a horizontal demand curve, and can only choose the quantity that equates its marginal cost and the market price. The table shows that the firm can choose different quantities and prices, and that the price decreases as the quantity increases, which are the characteristics of a monopolist. To find the profit-maximizing output and the price associated with selling it in the market, we need to find the marginal revenue and the marginal cost functions, and set them equal to each other. The marginal revenue is the additional revenue from selling one more unit, and the marginal cost is the additional cost from producing one more unit. The profit is the difference between the total revenue and the total cost. The marginal revenue function can be derived from the inverse demand function by using the following formula: MR P(1 + 1) _ Ed where P is the price, and Ed is the price elasticity of demand, which is the percentage change in quantity demanded divided by the percentage change in price. For a linear demand function, the price elasticity of demand varies along the curve, and can be calculated by using the following formula: where MO is the marginal cost. Since the marginal cost is constant and equal to 2, we can plug it into the formula and get: E: d P72 Plugging this into the marginal revenue formula, we get: 1 Simplifying, we get: MR:P(1+Q) Simplifying, we get: P2 MR:P1 (+ P) MR=P+P72 MR22P2 This is the marginal revenue function. To find the marginal cost function, we can use the fact that the marginal cost is constant and equal to 2. Therefore, the marginal cost function is: M022 To find the profit-maximizing output, we set the marginal revenue equal to the marginal cost and solve for :13. We get: 2P222 2P:4 13:2 Plugging this into the inverse demand function, we get: 2:m+20 m:18 This means that the profit-maximizing output is 18 units, and the price associated with selling it in the market is 2 dollars per unit. To discuss if the production and pricing decision is welfare maximizing or not, we need to compare the social surplus with the profit. The social surplus is the sum of the consumer surplus and the producer surplus, which measure the net benefits to the consumers and the producers, respectively. The consumer surplus is the difference between the willingness to pay and the price paid, and the producer surplus is the difference between the price received and the cost incurred. The deadweight loss is the reduction in the social surplus due to the market inefficiency. The social surplus can be represented by the area under the demand curve and above the supply curve, up to the equilibrium quantity and price. The consumer surplus can be represented by the area under the demand curve and above the price, up to the quantity sold. The producer surplus can be represented by the area above the supply curve and below the price, up to the quantity sold. The deadweight loss can be represented by the area under the demand curve and above the supply curve, between the quantity sold and the equilibrium quantity. Using the inverse demand function and the marginal cost function, we can graph the social surplus, the consumer surplus, the producer surplus, and the deadweight loss as follows: ![Graph] The graph shows that the monopolist produces less and charges more than the competitive equilibrium, which is where the demand curve intersects the supply curve. The competitive equilibrium quantity and price are 18 units and 2 dollars per unit, respectively. The monopolist's quantity and price are 9 units and 11 dollars per unit, respectively. The social surplus is the area of the triangle ABC, which is: SS : $(18)(20) : 180 The consumer surplus is the area of the triangle ADE, which is: as : ;(9)(9) : 40.5 The producer surplus is the area of the rectangle DEFG, which is: R9:wxu2y:& The profit is the producer surplus minus the fixed cost, which is: 1r:PSFC:8150:31 The deadweight loss is the area of the triangle EBC, which is: DWL:%wmn:m5 The graph shows that the production and pricing decision of the monopolist is not welfare maximizing, because it creates a deadweight loss and reduces the social surplus. The monopolist's profit is less than the social surplus, which means that there is a potential Pareto improvement, where some consumers and producers can be made better off without making anyone worse off. The monopolist's profit is also less than the consumer surplus under the competitive equilibrium, which means that the monopolist transfers some of the consumer surplus to itself, but also destroys some of it. Therefore, the production and pricing decision of the monopolist is inefficient and inequitable. 2. To find the profit-maximizing price that a company would charge for building the bridge, we need to find the demand function, which is the function that gives the quantity as a function of price. To do this, we can use the same method as in question 1, but with different points from the table. For example, using the points (0, 1000) and (5, 800), we get: _mim_amimm_ C2*C1 50 40 m ylmmzAmmm y:mx+mm The profit is the producer surplus minus the fixed cost, which is: 7r=PSFC=8150=31 The deadweight loss is the area of the triangle EBC, which is: DWL : 3020(0) : 40.5 The graph shows that the production and pricing decision of the monopolist is not welfare maximizing, because it creates a deadweight loss and reduces the social surplus. The monopolist's profit is less than the social surplus, which means that there is a potential Pareto improvement, where some consumers and producers can be made better off without making anyone worse off. The monopolist's profit is also less than the consumer surplus under the competitive equilibrium, which means that the monopolist transfers some of the consumer surplus to itself, but also destroys some of it. Therefore, the production and pricing decision of the monopolist is inefficient and inequitable. 2. To find the profit-maximizing price that a company would charge for building the bridge, we need to find the demand function, which is the function that gives the quantity as a function of price. To do this, we can use the same method as in question 1, but with different points from the table. For example, using the points (0, 1000) and (5, 800), we get: : ygiyl :8001000: 40 332331 50 m y 1000 : 40(a: 0) y : 7400 + 1000 This is the demand function. To find the profit-maximizing price, we need to find the marginal revenue