Using MATLAB: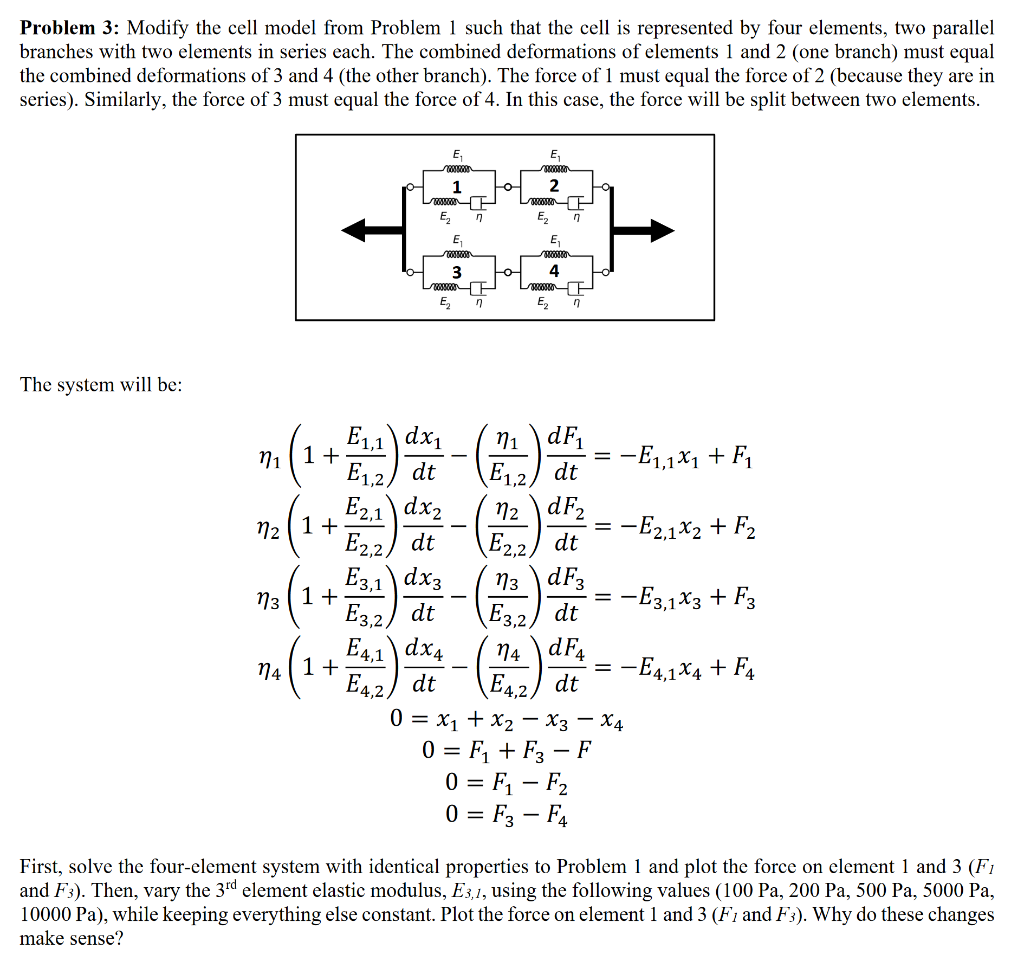

Problem 3: Modify the cell model from Problem 1 such that the cell is represented by four elements, two parallel branches with two elements in series each. The combined deformations of elements 1 and 2 (one branch) must equal the combined deformations of 3 and 4 (the other branch). The force of 1 must equal the force of 2 (because they are in series). Similarly, the force of 3 must equal the force of 4. In this case, the force will be split between two elements. E E 1 www. E, 2 LP E2 n n E SOLO 4 wa E n E2 n The system will be: = E1,2) = E2,2) = E1,1) dxi n1 dF ni (1+ -E1,1X1 + F E1,2) dt dt E2,1 dx2 n2 dF2 n2 1+ -E2,1X2 + F2 E2,2) dt dt E3,1 dxz n3 dFz n3 (1+ -E3,1X3 + F3 E3,2) dt E3,2) dt E4,1) dx4 n4 dF4 1 + E4,2) dt = -E4,1*4 + F4 E4,2) dt 0 = x1 + x2 X3 X4 0 = F + Fz F 0 = F F2 0 = F3 F4 n4 = First, solve the four-element system with identical properties to Problem 1 and plot the force on element 1 and 3 (F1 and F3). Then, vary the 3rd element elastic modulus, E3,1, using the following values (100 Pa, 200 Pa, 500 Pa, 5000 Pa, 10000 Pa), while keeping everything else constant. Plot the force on element 1 and 3 (Fi and F3). Why do these changes make sense? Problem 3: Modify the cell model from Problem 1 such that the cell is represented by four elements, two parallel branches with two elements in series each. The combined deformations of elements 1 and 2 (one branch) must equal the combined deformations of 3 and 4 (the other branch). The force of 1 must equal the force of 2 (because they are in series). Similarly, the force of 3 must equal the force of 4. In this case, the force will be split between two elements. E E 1 www. E, 2 LP E2 n n E SOLO 4 wa E n E2 n The system will be: = E1,2) = E2,2) = E1,1) dxi n1 dF ni (1+ -E1,1X1 + F E1,2) dt dt E2,1 dx2 n2 dF2 n2 1+ -E2,1X2 + F2 E2,2) dt dt E3,1 dxz n3 dFz n3 (1+ -E3,1X3 + F3 E3,2) dt E3,2) dt E4,1) dx4 n4 dF4 1 + E4,2) dt = -E4,1*4 + F4 E4,2) dt 0 = x1 + x2 X3 X4 0 = F + Fz F 0 = F F2 0 = F3 F4 n4 = First, solve the four-element system with identical properties to Problem 1 and plot the force on element 1 and 3 (F1 and F3). Then, vary the 3rd element elastic modulus, E3,1, using the following values (100 Pa, 200 Pa, 500 Pa, 5000 Pa, 10000 Pa), while keeping everything else constant. Plot the force on element 1 and 3 (Fi and F3). Why do these changes make sense