Using the handout solve all pls


1. Using sketches (i.e. re-draw the diagrams as seen in the hand-out), illustrate the symmetry elements (Point Groups) present as a result of adding a center of symmetry (i.e. Inversion Center) to the following groups: 1,m, 2mm, 4mm.
2. Referring again to the diagrams from the hand-out, list (1) of the 32 possible Point Groups which results from each combination below: a) (2) mirror planes oriented at 90 with respect to one another, and a diad (2-fold rotational axis) perpendicular to both. b) A 4 axis with a mirror oriented with its normal perpendicular to the roto-inversion axis.
3. Consider the image of a snowflake below. Assuming this is a well-formed crystal, and that there is some thickness associated (it is not simply a flat 2-D pattern), demonstrate the expected Point Group present by drawing and/or describing the symmetry elements present. Please also identify the Point Group you believe is associated with this crystal.

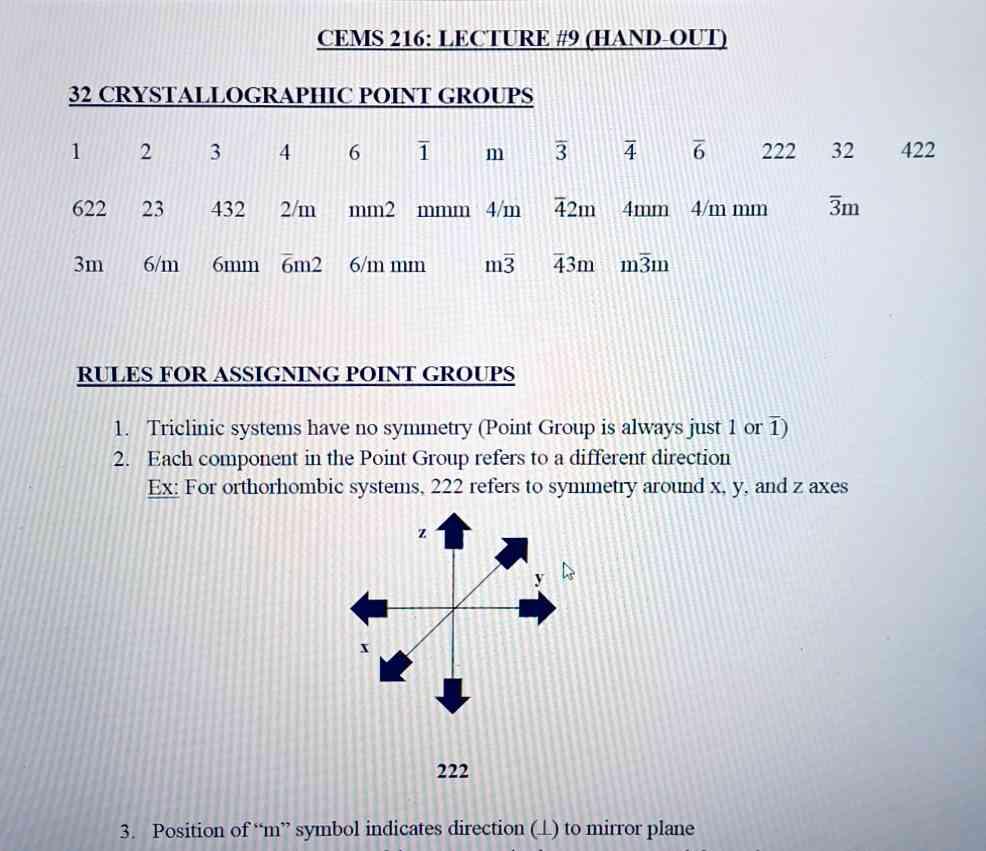
CEMS 216: LECTURE \#9 (HAND-OUT) RULES FOR ASSIGNING POINT GROUPS 1. Triclinic systems have no symmetry (Point Group is always just 1 or 1 ) 2. Each component in the Point Group refers to a different direction Ex: For orthorhombic systems, 222 refers to symmetry around x,y. and z axes 222 3. Position of " m " symbol indicates direction () to mirror plane 4. Fractions mean the axes of the operators in the numerator and denominator are () Ex: For monoclinic systems, 2/m means there is a mirror plane () to a 2 -fold rotational axis ("diad") 5. For orthorhombic systems, the (3) symbols refer to the (3) mutually ()x,y, and z axes 6. All tetragonal groups have a 4 or 4 rotation axis in the z-direction, and this distinctive feature is listed 1st - 2nd symbol refers to symmetry about ()x and y axes - 3rd symbol refers to the directions in the x-y plane that bisect the x and y axes 7. For trigonal (always have a 3 or 3 axis first) and hexagonal (always have a 6 or 6 axis first) systems, 2nd symbol describes symmetry around equivalent directions (120 or 60 apart) in the plane ( ( ) to the 3,3,6, or 6 axis 5. For orthorhombic systems, the (3) symbols refer to the (3) mutually ()x,y, and z axes 6. All tetragonal groups have a 4 or 4 rotation axis in the z-direction, and this distinctive feature is listed 1st - 2nd symbol refers to symmetry about ()x and y axes - 3rd symbol refers to the directions in the xy plane that bisect the x and y axes 7. For trigonal (always have a 3 or 3 axis first) and hexagonal (always have a 6 or 6 axis first) systems, 2nd symbol describes symmetry around equivalent directions (120 or 60 apart) in the plane () to the 3,3,6, or 6 axis - 3rd symbol in hexagonal groups refers to directions that bisect the angles between axes specified by 2nd symbol 8. If there is a " 3 " symbol in the 2nd position, point group is associated with a cubic system - " 3 " refers to rotation "triads" along the (4) body diagonals of the cube - 1st symbol refers to the cube axis - 3rd symbol refers to the face diagonals CEMS 216: LECTURE \#9 (HAND-OUT) RULES FOR ASSIGNING POINT GROUPS 1. Triclinic systems have no symmetry (Point Group is always just 1 or 1 ) 2. Each component in the Point Group refers to a different direction Ex: For orthorhombic systems, 222 refers to symmetry around x,y. and z axes 222 3. Position of " m " symbol indicates direction () to mirror plane 4. Fractions mean the axes of the operators in the numerator and denominator are () Ex: For monoclinic systems, 2/m means there is a mirror plane () to a 2 -fold rotational axis ("diad") 5. For orthorhombic systems, the (3) symbols refer to the (3) mutually ()x,y, and z axes 6. All tetragonal groups have a 4 or 4 rotation axis in the z-direction, and this distinctive feature is listed 1st - 2nd symbol refers to symmetry about ()x and y axes - 3rd symbol refers to the directions in the x-y plane that bisect the x and y axes 7. For trigonal (always have a 3 or 3 axis first) and hexagonal (always have a 6 or 6 axis first) systems, 2nd symbol describes symmetry around equivalent directions (120 or 60 apart) in the plane ( ( ) to the 3,3,6, or 6 axis 5. For orthorhombic systems, the (3) symbols refer to the (3) mutually ()x,y, and z axes 6. All tetragonal groups have a 4 or 4 rotation axis in the z-direction, and this distinctive feature is listed 1st - 2nd symbol refers to symmetry about ()x and y axes - 3rd symbol refers to the directions in the xy plane that bisect the x and y axes 7. For trigonal (always have a 3 or 3 axis first) and hexagonal (always have a 6 or 6 axis first) systems, 2nd symbol describes symmetry around equivalent directions (120 or 60 apart) in the plane () to the 3,3,6, or 6 axis - 3rd symbol in hexagonal groups refers to directions that bisect the angles between axes specified by 2nd symbol 8. If there is a " 3 " symbol in the 2nd position, point group is associated with a cubic system - " 3 " refers to rotation "triads" along the (4) body diagonals of the cube - 1st symbol refers to the cube axis - 3rd symbol refers to the face diagonals










