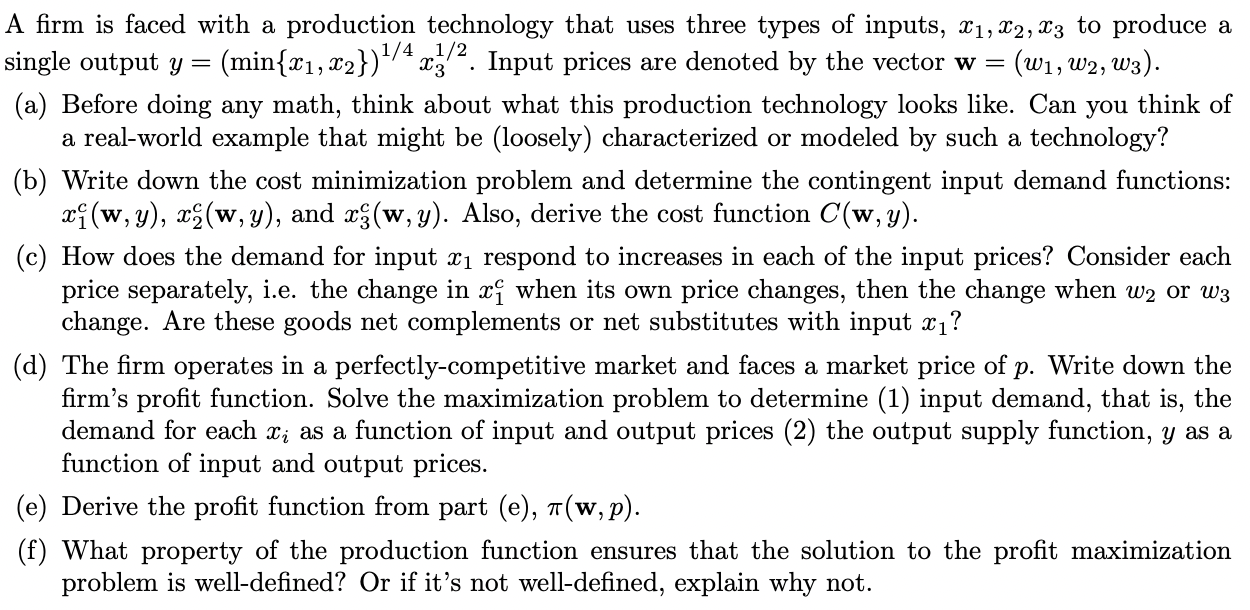
= V A firm is faced with a production technology that uses three types of inputs, X1, X2, X3 to produce a single output y = (min{21, 22})1/4 xz/?. Input prices are denoted by the vector w= ' (W1, W2, W3). (a) Before doing any math, think about what this production technology looks like. Can you think of a real-world example that might be (loosely) characterized or modeled by such a technology? (b) Write down the cost minimization problem and determine the contingent input demand functions: x (w,y), xz(w,y), and (w, y). Also, derive the cost function C(w,y). (c) How does the demand for input x respond to increases in each of the input prices? Consider each price separately, i.e. the change in x when its own price changes, then the change when w2 or w3 change. Are these goods net complements or net substitutes with input x? (d) The firm operates in a perfectly-competitive market and faces a market price of p. Write down the firm's profit function. Solve the maximization problem to determine (1) input demand, that is, the demand for each xi as a function of input and output prices (2) the output supply function, y as a function of input and output prices. (e) Derive the profit function from part (e), 7(w,p). (f) What property of the production function ensures that the solution to the profit maximization problem is well-defined? Or if it's not well-defined, explain why not. = V A firm is faced with a production technology that uses three types of inputs, X1, X2, X3 to produce a single output y = (min{21, 22})1/4 xz/?. Input prices are denoted by the vector w= ' (W1, W2, W3). (a) Before doing any math, think about what this production technology looks like. Can you think of a real-world example that might be (loosely) characterized or modeled by such a technology? (b) Write down the cost minimization problem and determine the contingent input demand functions: x (w,y), xz(w,y), and (w, y). Also, derive the cost function C(w,y). (c) How does the demand for input x respond to increases in each of the input prices? Consider each price separately, i.e. the change in x when its own price changes, then the change when w2 or w3 change. Are these goods net complements or net substitutes with input x? (d) The firm operates in a perfectly-competitive market and faces a market price of p. Write down the firm's profit function. Solve the maximization problem to determine (1) input demand, that is, the demand for each xi as a function of input and output prices (2) the output supply function, y as a function of input and output prices. (e) Derive the profit function from part (e), 7(w,p). (f) What property of the production function ensures that the solution to the profit maximization problem is well-defined? Or if it's not well-defined, explain why not