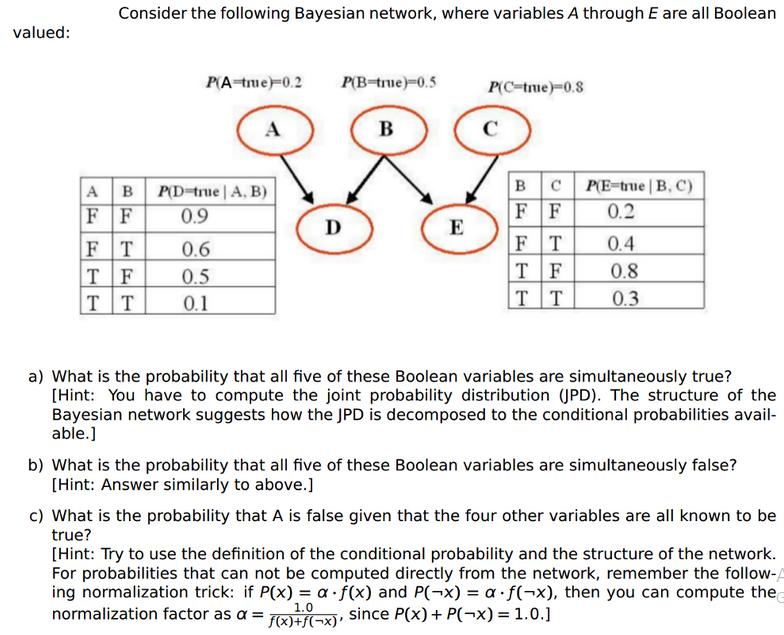
valued: Consider the following Bayesian network, where variables A through E are all Boolean P(A-true)-0.2 P(B-true)=0.5 A B P(C=true)=0.8 A B P(D=true |A, B) BCPE-true | B, C) FF 0.9 FF 0.2 D E FT 0.6 FT 0.4 TF 0.5 T F 0.8 TT 0.1 TT 0.3 a) What is the probability that all five of these Boolean variables are simultaneously true? [Hint: You have to compute the joint probability distribution (JPD). The structure of the Bayesian network suggests how the JPD is decomposed to the conditional probabilities avail- able.] b) What is the probability that all five of these Boolean variables are simultaneously false? [Hint: Answer similarly to above.] c) What is the probability that A is false given that the four other variables are all known to be true? [Hint: Try to use the definition of the conditional probability and the structure of the network. For probabilities that can not be computed directly from the network, remember the follow-A ing normalization trick: if P(x) = a f(x) and P(x) = a f(x), then you can compute the normalization factor as a = f(x)+f(x), since P(x) + P(x) = 1.0.] 1.0