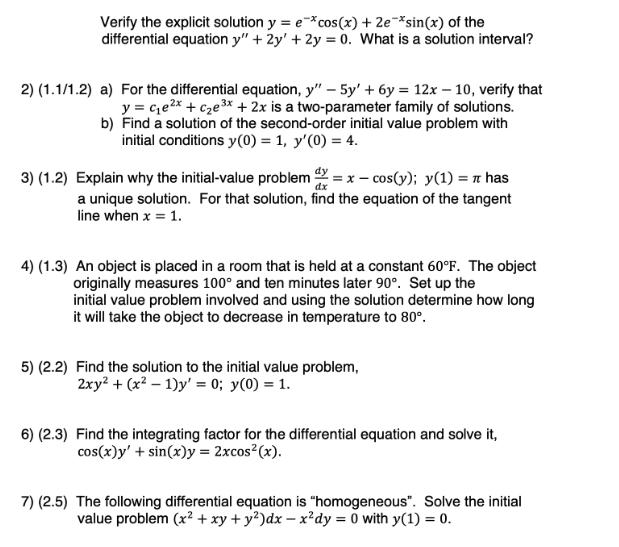
Verify the explicit solution y = e* cos(x) + 2e*sin(x) of the differential equation y" +2y' + 2y = 0. What is a solution interval? 2) (1.1/1.2) a) For the differential equation, y" - 5y' + 6y=12x-10, verify that y=e2x + czex + 2x is a two-parameter family of solutions. b) Find a solution of the second-order initial value problem with initial conditions y(0) = 1, y'(0) = 4. 3) (1.2) Explain why the initial-value problem dx = x = cos(y); y(1) = has dx a unique solution. For that solution, find the equation of the tangent line when x = 1. 4) (1.3) An object is placed in a room that is held at a constant 60F. The object originally measures 100 and ten minutes later 90. Set up the initial value problem involved and using the solution determine how long it will take the object to decrease in temperature to 80. 5) (2.2) Find the solution to the initial value problem, 2xy+(x-1)y' = 0; y(0) = 1. 6) (2.3) Find the integrating factor for the differential equation and solve it, cos(x)y' + sin(x)y = 2xcos(x). 7) (2.5) The following differential equation is "homogeneous". Solve the initial value problem (x + xy + y)dx - xdy = 0 with y(1) = 0.