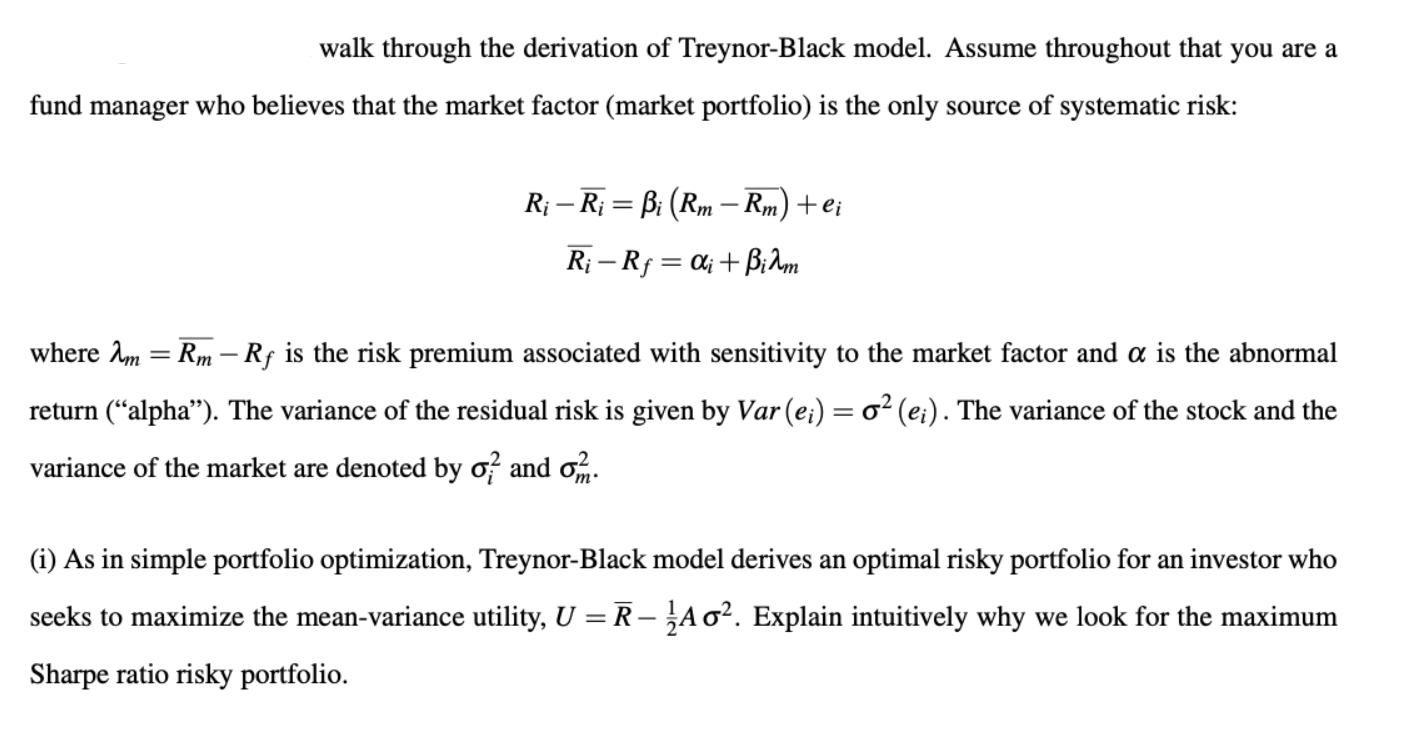
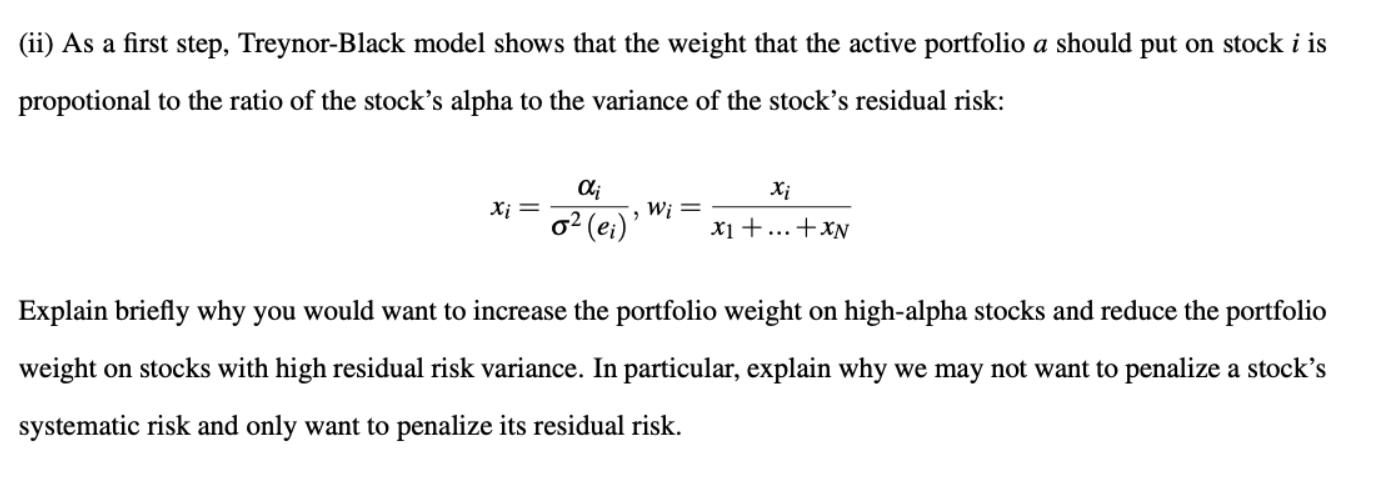
walk through the derivation of Treynor-Black model. Assume throughout that you are a fund manager who believes that the market factor (market portfolio) is the only source of systematic risk: Ri - Ri= Bi (Rm - Rm) + ei Ri-Rf=ai + Bim where 2m = Rm - Rf is the risk premium associated with sensitivity to the market factor and a is the abnormal return ("alpha"). The variance of the residual risk is given by Var (ei) = 0 (ei). The variance of the stock and the variance of the market are denoted by of and om. (i) As in simple portfolio optimization, Treynor-Black model derives an optimal risky portfolio for an investor who seeks to maximize the mean-variance utility, U =R-Ao. Explain intuitively why we look for the maximum Sharpe ratio risky portfolio. (ii) As a first step, Treynor-Black model shows that the weight that the active portfolio a should put on stock i is propotional to the ratio of the stock's alpha to the variance of the stock's residual risk: Xi = i Xi Wi = 0 (ei)' x + ... + XN Explain briefly why you would want to increase the portfolio weight on high-alpha stocks and reduce the portfolio weight on stocks with high residual risk variance. In particular, explain why we may not want to penalize a stock's systematic risk and only want to penalize its residual risk.